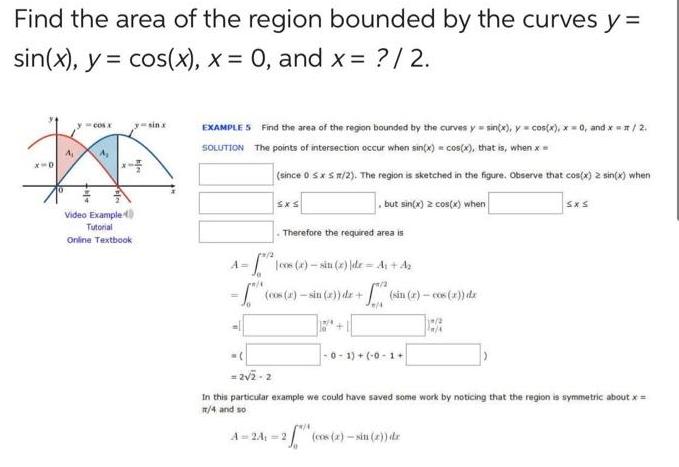
Find the area of the region bounded by the curves y = sin(x), y = cos(x), x = 0, and x = ? / 2. x=0 A y cox A y sin x Video Example) Tutorial Online Textbook EXAMPLE 5 Find the area of the region bounded by the curves y=sin(x), y = cos(x), x=0, and x = x/2. SOLUTION The points of intersection occur when sin(x) = cos(x), that is, when x = (since 0 5 x 5 m/2). The region is sketched in the figure. Observe that cos(x) 2 sin(x) when .but sin(x) = cos(x) when A= -1." SXS Therefore the required area is */2 [cos (x) - sin(x) \de=A + A (cos(r)-sin (r)) de + m/2 Jell (sin (r)-cos(x)) de -0-1)+(-0-1+ 10/2 10/1 SXS = 22-2 In this particular example we could have saved some work by noticing that the region is symmetric about x = n/4 and so 2 " (cos (2) -sin (2)) de