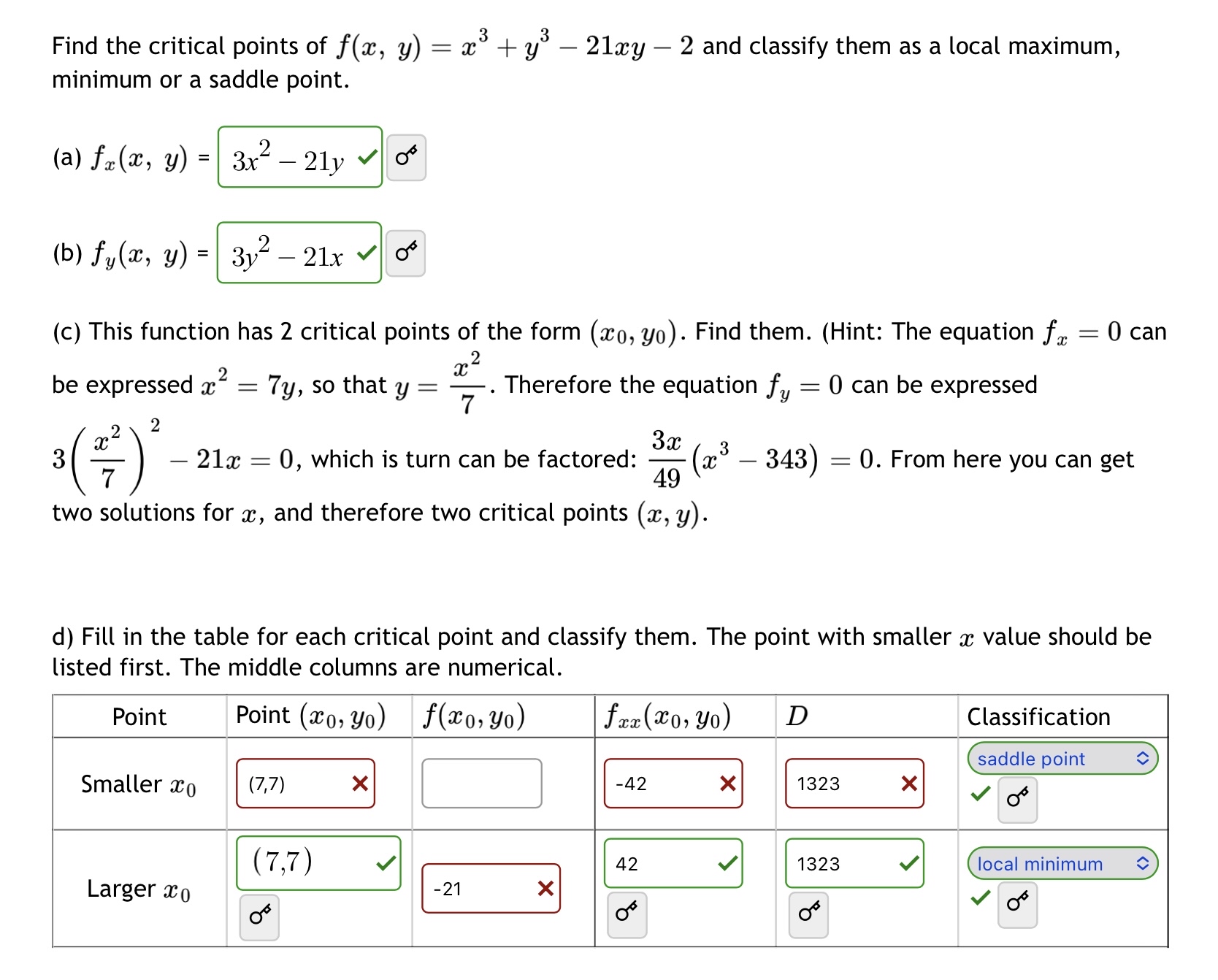
Find the critical points of $f(x,y)=x^3+y^3-21xy-2$ and classify them as a local maximum, minimum or a saddle point.

$($a$)f_x(x,y)=$

$($b$)f_y(x,y)=$

$($c$)$ This function has $2$ critical points of the form $(x_0,y_0).$ Find them. $($Hint: The equation $f_x=0$ can be expressed $x^2=7y,$ so that $y=\frac{x^2}{7}.$ Therefore the equation $f_y=0$ can be expressed $3(\frac{x^2}{7}{)}^2-21x=0,$ which is turn can be factored: $\frac{3x}{49}(x^3-343)=0.$ From here you can get two solutions for $x,$ and therefore two critical points $(x,y).$

d$)$ Fill in the table for each critical point and classify them. The point with smaller $x$ value should be listed first. The middle columns are numerical.

$\backslash$table$[[$Point$,$Point $(x_0,y_0),f(x_0,y_0),f_{}(x_0,y_0),D,$Classification$],[$Smaller $x_0,,,,,$saddle point$],[,,,,0$