Find The Indefinite Integral (Antiderivative) Of 2x E- Dx Using Integration By Parts 1. Split The Integral Into Two Factors: U = 2x And Dy = E* Dx Then Find The Derivative Of U And The Antiderivative Of Dv. Du = Derivative Of U Multiplied By Dx (Du = V'dx) V = Antiderivative Of Dv (Do Not Include "+C") U = 2x VE -E* Du = 2dx Dv = E** Dx 2. Use The Above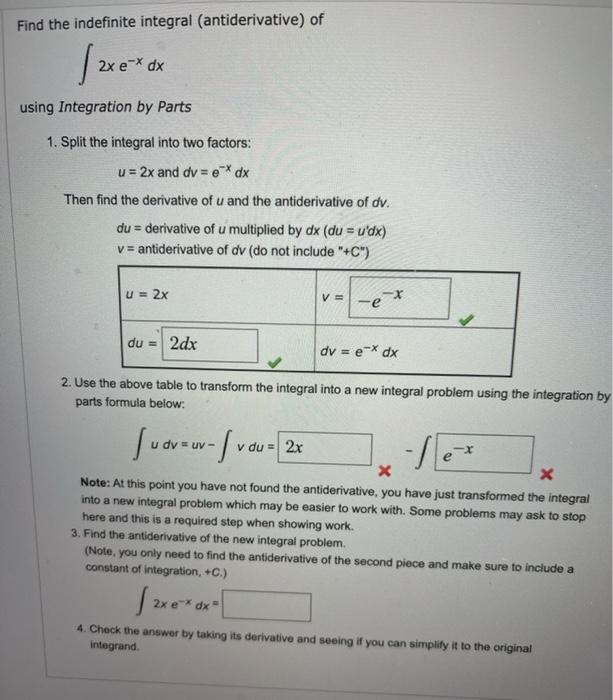

Find the indefinite integral (antiderivative) of 2x ex 2x ex dx using Integration by Parts 1. Split the integral into two factors: u=2x and dv=ex dx Then find the derivative of u and the antiderivative of dv. = du derivative of u multiplied by dx (du = u'dx) vantiderivative of dv (do not include "+C") u = 2x V= -ex du = 2dx dv = ex dx 2. Use the above table to transform the integral into a new integral problem using the integration by parts formula below: Judv=u-vous vdu = 2x -x X Note: At this point you have not found the antiderivative, you have just transformed the integral into a new integral problem which may be easier to work with. Some problems may ask to stop here and this is a required step when showing work. 3. Find the antiderivative of the new integral problem. (Note, you only need to find the antiderivative of the second piece and make sure to include a constant of integration, +C.) 2x ex d dx= 4. Check the answer by taking its derivative and seeing if you can simplify it to the original integrand.