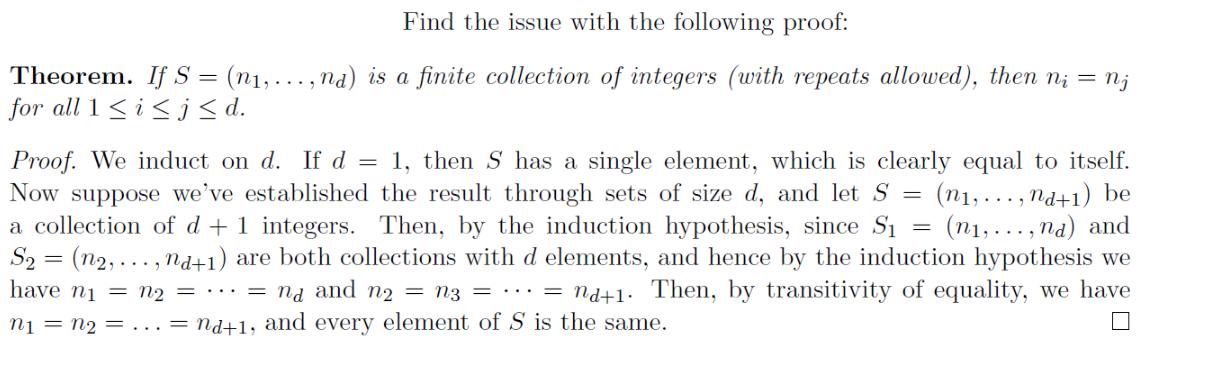
Find the issue with the following proof: Theorem. If S = (n1,..., nd) is a finite collection of integers (with repeats allowed), then ni = nj for all 1i j d. = === Proof. We induct on d. If d = 1, then S has a single element, which is clearly equal to itself. Now suppose we've established the result through sets of size d, and let S (n1,...,nd+1) be a collection of d + 1 integers. Then, by the induction hypothesis, since S (n1,...,nd) and S2 = (n2,...,nd+1) are both collections with d elements, and hence by the induction hypothesis we have n = n2 = ... = nd and n2 = n3 = ... = nd+1. Then, by transitivity of equality, we have n = n2 = ... = nd+1, and every element of S is the same.