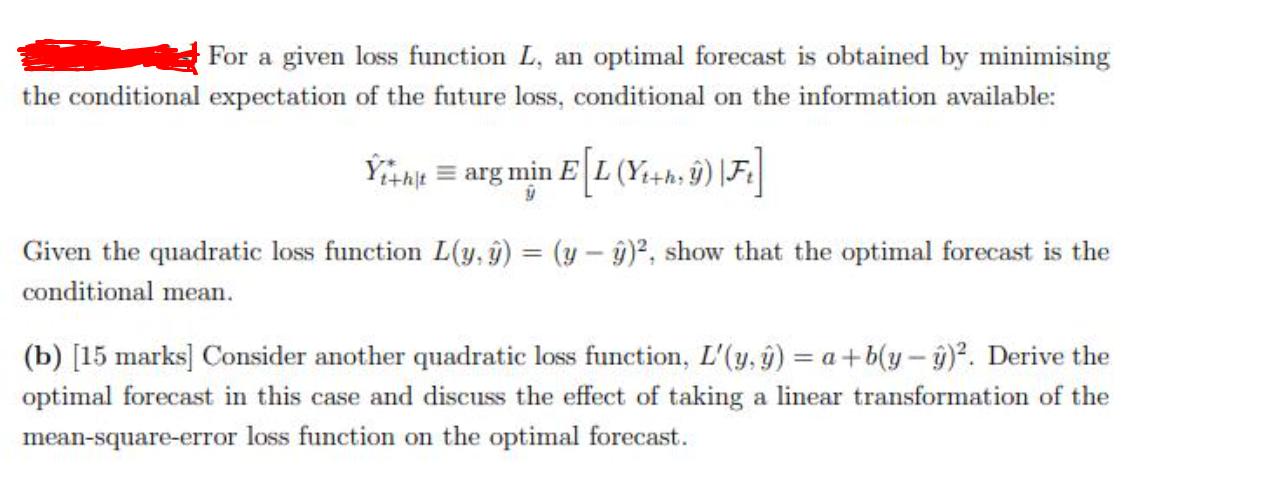
For a given loss function L, an optimal forecast is obtained by minimising the conditional expectation of the future loss, conditional on the information available: Yi+Alt = arg min E L (Y1+h; 9) |Fr] t+ht y Given the quadratic loss function L(y, y) = (y- y), show that the optimal forecast is the conditional mean. (b) [15 marks] Consider another quadratic loss function, L' (y, y) = a +b(y- y). Derive the optimal forecast in this case and discuss the effect of taking a linear transformation of the mean-square-error loss function on the optimal forecast.