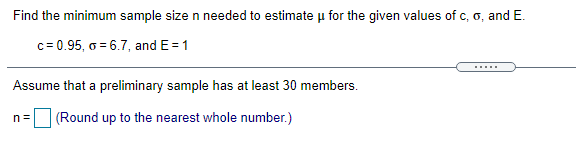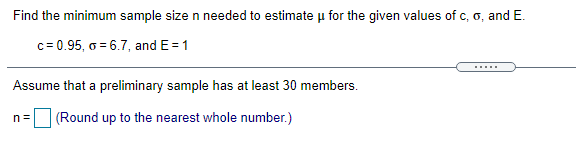
For each level of confidence c below, determine the corresponding normal confidence interval. Assume each confidence interval is constructed for the same sample statistics. 17. c = 0.85 18. c= 0.88 19. c = 0.95 20. c = 0.98 55 x = 56.7 58.4 54.9 x = 56.7 58.5 52 53 54 55 56 57 58 59 60 61 52 53 54 55 56 57 58 59 60 61 54.4 x = 56.7 59 53.9 x = 56.7 59.5 52 53 54 55 56 57 58 59 60 61 52 53 54 55 56 57 58 59 60 61 Drag each normal confidence interval given above to the level of confidence c. 17. 18. 19. 20.Find the critical value to for the confidence level c = 0.98 and sample size n = 29. = Click the icon to view the t-distribution table. (Round to the nearest thousandth as needed.)Find me critical 1value 2:: necessary to form a condence interval at the level of condence shown below. c=l].35 25D {Round to two decimal places as needed.) You are given the sample mean and the population standard deviation. Use this information to constmct the 90% and 95% condence intervals for the population mean. Interpret the results and compare the widths of the condence intervals. From a random sample of48 business days, the mean closing price of a certain stock was $113.99. Assume the populaon standard deviation is $10.?'l. ( ..... The 90% condence interval is (H. 1. (Round to two decimal places as needed.) The 95% condence interval is [Ll,_]. (Round to two decimal places as needed.) Which interval is wider? Choose the correct answer below. 0 The 95% condence interval 0 The 90% condence interval Interpret the results. 0 A. You can be certain that the closing price of the stock was within the 90% condence interval for approximately 43 of the 48 days. and was within the 95% condence interval for approximately 46 of the 48 days. 0 B. You can be 90% condent that the population mean price of the stock is between the bounds of the 90% condence interval, and 95% condent for the 95% interval. 0 C. You can be certain that the population mean price of the stock is either between the lower bounds of the 90% and 95% condence intervals or the upper bounds of the 90% and 95% condence intervals. 0 D. You can be 90% condent that the population mean price 01 the stock is outside the bounds of the 90% condence interval, and 95% condent for the 95% interval. Acompany manufactures tennis balls. When its tennis balls are dropped onto a concrete surface from a height of 100 inches, the company wants the mean height the balls bounce upward to be 55.1 inches. This average is maintained by periodically testing random samples of 25 tennis balls. If the t-value falls between toso and toso- then the company will be satised that it is manufacturing acceptable tennis ballsAsample oi 25 balls is randomly selected and tested. The mean bounce height of the sample is 56.8 inches and the standard deviation is 0.25 inch. Assume the bounce heights are approximately normally distributed. Is the company making acceptable tennis balls? Find 4030 and topo- 'toso 2 El tcoo = D (Round to three decimal places as needed.) Find the t-value. tvalue = B Is the company making acceptable tennis balls? Choose the correct answer below. C) it. The tennis balls are acceptable because the tvalue tails between toso and topo- O B. The tennis balls are not acceptable because the tvalue falls outside toso and toso- O C. The sample is not large enough to determine if the tennis balls are acceptable. Find the minimum sample size n needed to estimate p for the given values of e, a, and E. e=l].95, U: 6?, and E=1 Assume that a preliminary sample has at least 30 members. n = D (Round up tn the nearest whole number} Acheese processing company wants to estimate the mean cholesterol content of all oneounce servings of a type at cheese. The estimate must be within 0.?9 milligram of the population mean. (a) Determine the minimum sample size required to constmct a 95% condence interval for the population mean. Assume the population standard deviation is 3.11 milligrams. (b) The sample mean is 34 milligrams. Using the minimum sample size with a 95% level of condence, does it seem likely that the population mean could be w'rthin 3% oi the sample mean? within 113% of the sample mean? Explain. Click here to view page 1 of the Standard Normal Table. Click here view page 2 ofthe Standard Normal Table. (a) The minimum sample size required to consthct a 95% condence interval is servings. (Round up to the nearest whole number.) (b) The 95% condence interval is l[ I I 1. lt IE seem likely that the population mean could be within 3% of the sample mean because 3% o from the sample mean would fall E the condence interval. It E seem likely that the population mean could be within 0.3% of the sample mean because 0.3% o from the sample mean would tall I:I the condence interval. (Round to two decimal places as needed.)