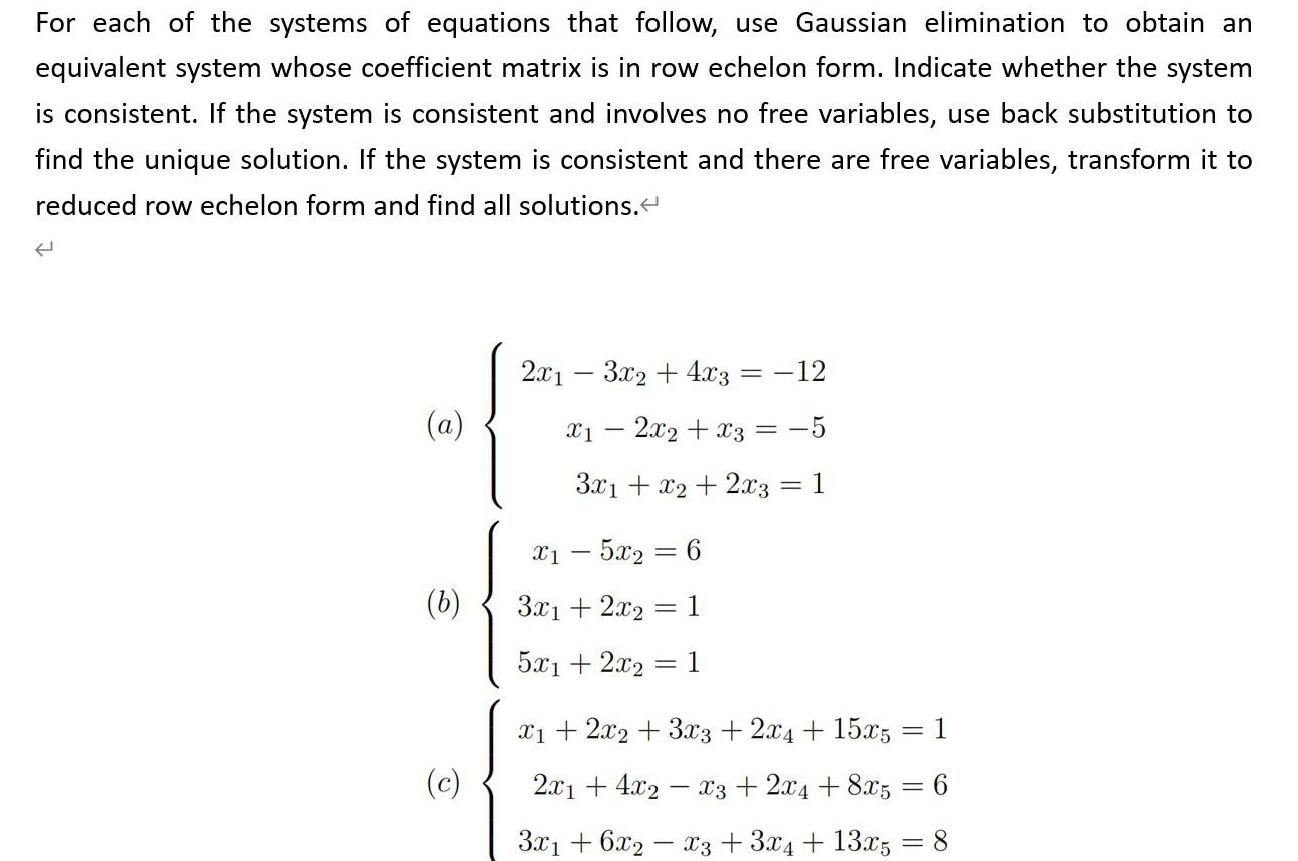
For each of the systems of equations that follow, use Gaussian elimination to obtain an equivalent system whose coefficient matrix is in row echelon form. Indicate whether the system is consistent. If the system is consistent and involves no free variables, use back substitution to find the unique solution. If the system is consistent and there are free variables, transform it to reduced row echelon form and find all solutions. < 2x13x2+4x3 = = -12 (a) x12x2 x3 = -5 3x1+x22x3 = 1 x1 - 5x2 = 6 (b) 3x1 + 2x2 == 1 5x1 + 2x2 == 1 x12x2 3x3 + 2x4 + 155 (c) 2x1 + 4x2 - 1 x32x4 +8x5 = 3x16x2 x3 + 3x4 + 13x5 - 8