Question: For equation: 1 = (60 = (ex + cos x) dx a. Calculate the integral using Simpson 3/8. b. Calculate the integral using Romberg

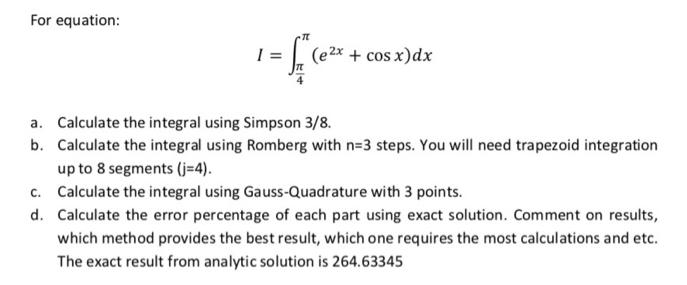
For equation: 1 = (60 = (ex + cos x) dx a.

For equation: 1 = (60 = (ex + cos x) dx a. Calculate the integral using Simpson 3/8. b. Calculate the integral using Romberg with n=3 steps. You will need trapezoid integration up to 8 segments (j=4). c. Calculate the integral using Gauss-Quadrature with 3 points. d. Calculate the error percentage of each part using exact solution. Comment on results, which method provides the best result, which one requires the most calculations and etc. The exact result from analytic solution is 264.63345

Step by Step Solution

★★★★★

3.60 Rating (186 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock

To calculate the integral using different methods and evaluate their errors well need to define the integrand function and perform the calculations step by step Lets start with the Simpson 38 rule a S... View full answer

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Accounting Questions!

Consider the diode bridge rectifier shown below with the voltage regulating capacitor in parallel with the load resistor. 60Hz 120V 10:1 C-470F IL R=1kQ a) For the input signal, sketch waveforms of...

Which inventory method (perpetual or periodic) provides better control over a firms inventory?

Integration provides a means to compute how much mass enters or leaves a reactor over a specified time period, as in Where t1 and t2 = the initial and final times, respectively. This formula makes...

For what values of is e ax cos x dx convergent? Evaluate the integral for those values of a.

Refer to Exercise 71. a. The following table shows the method of tabular integration applied to e x cos x dx. Use the table to express e x cos x dx in terms of the sum of functions and an...

For Exercises, use the integration feature on a graphing calculator and successively larger values of b to estimate 0 f(x) dx. b ex cos x dx

Suppose that a stress T zj applied (x o ) is applied on the face z = 0 of a half-infinite elastic body (one that fills the region z >0). Then by virtue of the linearity of the elastostatics equation...

(a) Let f(x) be an even function of x so that f(x) = f (-x). Show that a-a (x) dx = 2 a0 (x) dx. (Hint: Write the integral from -a to a as the sum of the integral from -a to 0 and the integral...

The Maclaurin series expansion for cos x is Starting with the simplest version, cos x = 1, add terms one at a time to estimate cos (?/3). After each new term is added, compute the-true and...

Distribution Solutions, Inc., (DSI) is a regional distributor providing logistical support for merchandisers over a tristate area. Its distribution centers have been profitable until recently. DSI...

Prompt 1 You have probably had experience working in collaborative teams in previous courses. Describe some of your best and worst experiences. What did you learn that would help in future...

40 kN R1-5: A circular plate of diameter 120 mm and thickness 10 mm is placed over a circular hole of diameter 60 mm in the ground. A circular shaft of diameter 50 mm sits on top of the plate and has...

If the tape timer creates dots at a rate of 28Hz, what time interval in seconds does 3 successive dotsrepresent?

Perceived environmental uncertainty is a function of environmental complexity and: receptivity productivity change contingency Question 8 14 points

Aguila Corporation produces a single product. Variable manufacturing overhead is applied to products on the basis of direct labor-hours. The standard costs for one unit of product are as follows:...

Schedule of Cost of Goods Manufactured and Sold At December 31, 2019, the end of its fiscal year, Lederman Manufacturing Corporation collected the following data for 2019: BusinessCourse Return to...

Thanks in advance. I'm having trouble inputting the following code into my compiler and need help with this assignment. The material is confusing for me. I had my professor try to explain it but it...

Can partitioned join be used for r r.A s? Explain your answer

Triangular Determinants Show that the determinant of an upper triangular matrix is the product of its diagonal elements. The diagonal matrix is a special case. The statement for a lower triangular...

Two chemicals A and B react to form the substance X according to the law dx /dt = k(100 - x) (50- x), where k is a positive constant and x is the amount of substance X. Describe the amount of...

If the number of bacteria in a culture is 5 million at the end of 6 hours and 8 million at the end of 9 hours, how many were present initially?

A few years ago, the Toronto plant of a multinational soup producer was in competition with its U.S. sister plant to supply the Northeastern U.S. market. However, its cost of production was not...

What are the main advantages of a product layout? The main disadvantages?

A company plans to begin production of a new small appliance. The manager must decide whether to purchase the motors for die appliance from a vendor at $7 each or to produce them in-house. Either of...