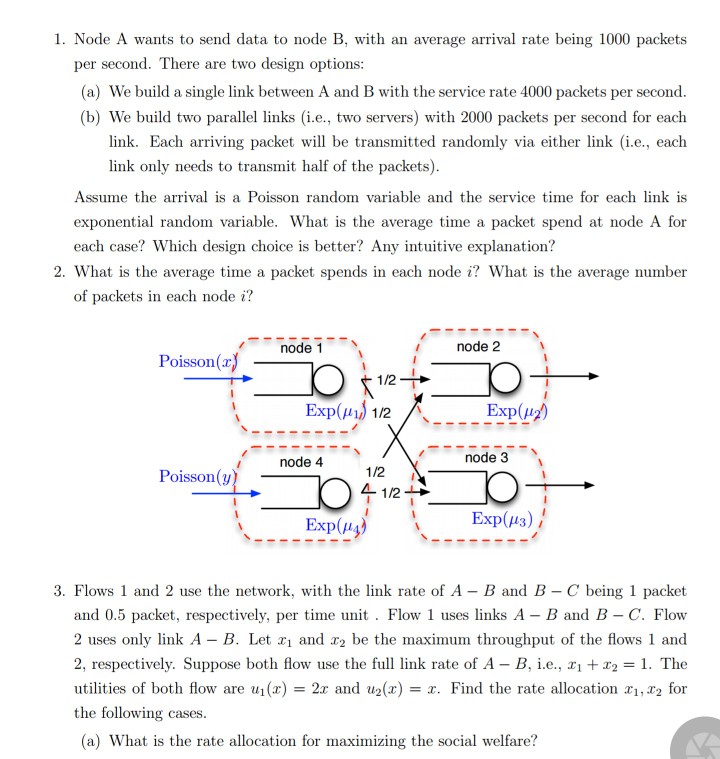
for question number 2

1. Node A wants to send data to node B, with an average arrival rate being 1000 packets per second. There are two design options (a) We build a single link between A and B with the service rate 4000 packets per second (b) We build two parallel links i.e., two servers) with 2000 packets per second for each link. Each arriving packetwl be transmitted randomly via either link (i.e., each link only needs to transmit half of the packets) Assume the arrival is a Poisson random variable and the service time for each link is exponential random variable. What is the average time a packet spend at node A for each case? Which design choice is better? Any intuitive explanation? 2. What is the average time a packet spends in each node i? What is the average number of packets in each nodei? node 1 node 2 Poisson(a) 1/2T Exp H /2- Exp(2) node 3 node 4 1/2 Poisson ( Exp (u3) Exp(3) 3. Flows 1 and 2 use the network, with the link rate of A B and B-C being 1 packet and 0.5 packet, respectively, per time unit. Flow 1 uses links A - B and B - C. Flow 2 uses only link A - B. Let i and r2 be the maximum throughput of the flows 1 and 2, respectively. Suppose both flow use the full link rate of A-B. i.e., x1 +T2 = 1 . The utilities of both flow are ul(x)-2x and u2(x) = x. Find the rate allocation x1,x2 for the following cases (a) What is the rate allocation for maximizing the social welfare? 1. Node A wants to send data to node B, with an average arrival rate being 1000 packets per second. There are two design options (a) We build a single link between A and B with the service rate 4000 packets per second (b) We build two parallel links i.e., two servers) with 2000 packets per second for each link. Each arriving packetwl be transmitted randomly via either link (i.e., each link only needs to transmit half of the packets) Assume the arrival is a Poisson random variable and the service time for each link is exponential random variable. What is the average time a packet spend at node A for each case? Which design choice is better? Any intuitive explanation? 2. What is the average time a packet spends in each node i? What is the average number of packets in each nodei? node 1 node 2 Poisson(a) 1/2T Exp H /2- Exp(2) node 3 node 4 1/2 Poisson ( Exp (u3) Exp(3) 3. Flows 1 and 2 use the network, with the link rate of A B and B-C being 1 packet and 0.5 packet, respectively, per time unit. Flow 1 uses links A - B and B - C. Flow 2 uses only link A - B. Let i and r2 be the maximum throughput of the flows 1 and 2, respectively. Suppose both flow use the full link rate of A-B. i.e., x1 +T2 = 1 . The utilities of both flow are ul(x)-2x and u2(x) = x. Find the rate allocation x1,x2 for the following cases (a) What is the rate allocation for maximizing the social welfare