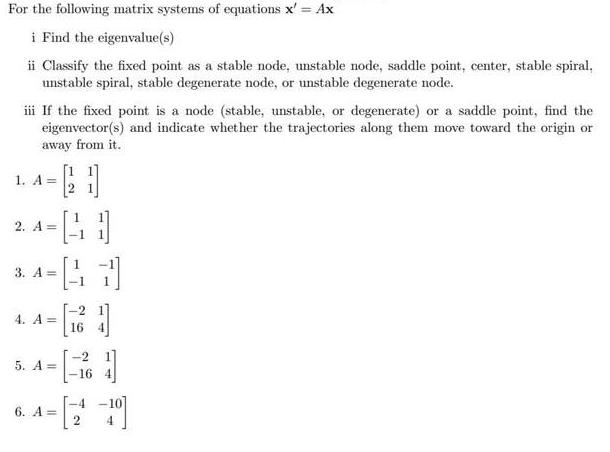
For the following matrix systems of equations x' = Ax i Find the eigenvalue(s) ii Classify the fixed point as a stable node, unstable node, saddle point, center, stable spiral, unstable spiral, stable degenerate node, or unstable degenerate node. iii If the fixed point is a node (stable, unstable, or degenerate) or a saddle point, find the eigenvector(s) and indicate whether the trajectories along them move toward the origin or away from it. 1. A= 2. A= 3. A= 4. A = 5. A = 6. A= 12 41 41 -2 16 -2 -16 2 4