Formal Logic
Here are some example questions and explanations
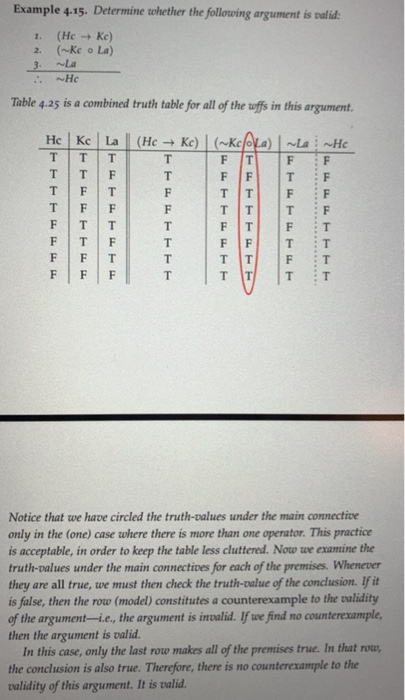
For each argument below, make a complete truth table (circling the truth-values under the main connectives) and use the completed table to determine whether the argument is valid. If it is not valid, place an x' at the end of each row that constitutes a counterexample to validity a) {(Hd Jc), -Jc} = (Jc Hd) b) {[(Hg + Kd) + Fa], -Hg} = (Kd ~Fa) 1. 2. Example 4.17. Determine whether the following argument is valid: (Gd o He) (Jk +Hc) (Jk Gd) Table 4.27 (next page) is a combined truth table for all of the wffs in this argument. In this case, the premises are all true in rows 2, 3, 4, 7, and 8. In rows 2, 3, 4, and 8, the conclusion is also true. However, in row 7, the con- clusion is false. The model of row 7 therefore constitutes a counterexample to the validity of this argument--that model shows that it is possible to make the premises all true, but the conclusion false. Notice that the truth table tells us exactly how to make all of the premises true, but the conclusion false. We do so by following what the model of row 7 says, namely: make Gd and Hc false, and Jk true. Doing so makes the premises true, but the conclusion false, and therefore, this argument is invalid. (Ga_Hc) (kA ~HC) (lk + Ga) Gd T T T T F F F F Hc JK T T T F F T F F T T T F F T F F TF TF FF FF T T F F TF TT FF T T T T F T F F TIT 1. Example 4.15. Determine whether the following argument is valid: (Heke) (Ke La) 3. LA . Hc 2. Table 4.25 is a combined truth table for all of the toffs in this argument. F Hc T T T T F F F F T F F T T F F La T F T F T F T F (HG 4 Kc) | (Kcola) | La He T F T F T F F T F F T T F F F T T T F T F T F T T F F T T T T F T T T T T Notice that we have circled the truth-values under the main connective only in the (one) case where there is more than one operator. This practice is acceptable, in order to keep the table less cluttered. Now we examine the truth-values under the main connectives for each of the premises. Whenever they are all true, we must then check the truth-value of the conclusion. If it is false, then the row (model) constitutes a counterexample to the validity of the argument-.e., the argument is invalid. If we find no counterexample, then the argument is valid. In this case, only the last row makes all of the premises true. In that row, the conclusion is also true. Therefore, there is no counterexample to the validity of this argument. It is valid. For each argument below, make a complete truth table (circling the truth-values under the main connectives) and use the completed table to determine whether the argument is valid. If it is not valid, place an x' at the end of each row that constitutes a counterexample to validity a) {(Hd Jc), -Jc} = (Jc Hd) b) {[(Hg + Kd) + Fa], -Hg} = (Kd ~Fa) 1. 2. Example 4.17. Determine whether the following argument is valid: (Gd o He) (Jk +Hc) (Jk Gd) Table 4.27 (next page) is a combined truth table for all of the wffs in this argument. In this case, the premises are all true in rows 2, 3, 4, 7, and 8. In rows 2, 3, 4, and 8, the conclusion is also true. However, in row 7, the con- clusion is false. The model of row 7 therefore constitutes a counterexample to the validity of this argument--that model shows that it is possible to make the premises all true, but the conclusion false. Notice that the truth table tells us exactly how to make all of the premises true, but the conclusion false. We do so by following what the model of row 7 says, namely: make Gd and Hc false, and Jk true. Doing so makes the premises true, but the conclusion false, and therefore, this argument is invalid. (Ga_Hc) (kA ~HC) (lk + Ga) Gd T T T T F F F F Hc JK T T T F F T F F T T T F F T F F TF TF FF FF T T F F TF TT FF T T T T F T F F TIT 1. Example 4.15. Determine whether the following argument is valid: (Heke) (Ke La) 3. LA . Hc 2. Table 4.25 is a combined truth table for all of the toffs in this argument. F Hc T T T T F F F F T F F T T F F La T F T F T F T F (HG 4 Kc) | (Kcola) | La He T F T F T F F T F F T T F F F T T T F T F T F T T F F T T T T F T T T T T Notice that we have circled the truth-values under the main connective only in the (one) case where there is more than one operator. This practice is acceptable, in order to keep the table less cluttered. Now we examine the truth-values under the main connectives for each of the premises. Whenever they are all true, we must then check the truth-value of the conclusion. If it is false, then the row (model) constitutes a counterexample to the validity of the argument-.e., the argument is invalid. If we find no counterexample, then the argument is valid. In this case, only the last row makes all of the premises true. In that row, the conclusion is also true. Therefore, there is no counterexample to the validity of this argument. It is valid