FORMULA FROM d) GIVEN HERE:

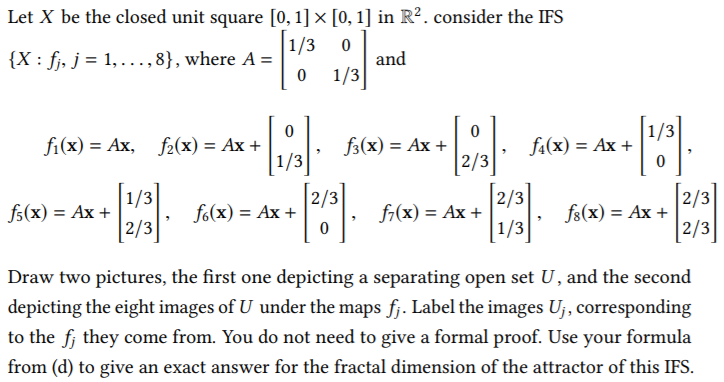
Let X be the closed unit square [0, 1] x [0, 1] in R. consider the IFS 1/3 0 {X: fj, j = 1,...,8}, where A = and 0 1/3 0 0 1/3 fi(x) = Ax, f(x) = Ax + Los f3(x) = Ax + f(x) = Ax + 1/3 2/3 0 2/3 f(x) = Ax + 1/3 (2/3) f(x) = Ax + f (x) = Ax+ 2/3 1/3 f(x) = Ax + [2/3] (2/3 0 N Draw two pictures, the first one depicting a separating open set U, and the second depicting the eight images of U under the maps f;. Label the images U, corresponding to the f; they come from. You do not need to give a formal proof. Use your formula from (d) to give an exact answer for the fractal dimension of the attractor of this IFS. am IFS, Let { I ;w, wz,..., WN} be where I c Rm is closed and each wi is a similitude I I with scaling factor si Let W denote the Hutchinson operator associated to this IFS. Suppose that there exists an open set us I such that exists (A) W(U) SU and wilu) nw; (u) = 0, i7j Then the fractal dimension of the IFS's attractor A. and satisfies the equation. (!) E Isil 1 N TEL (!) E lsil? = 1 EL Remarks: When all the similitudes have the Same scaling fact sis, fact s;=s, isl,...,N, then (!) is easy to solve: D = ln (N) ln (5) Let X be the closed unit square [0, 1] x [0, 1] in R. consider the IFS 1/3 0 {X: fj, j = 1,...,8}, where A = and 0 1/3 0 0 1/3 fi(x) = Ax, f(x) = Ax + Los f3(x) = Ax + f(x) = Ax + 1/3 2/3 0 2/3 f(x) = Ax + 1/3 (2/3) f(x) = Ax + f (x) = Ax+ 2/3 1/3 f(x) = Ax + [2/3] (2/3 0 N Draw two pictures, the first one depicting a separating open set U, and the second depicting the eight images of U under the maps f;. Label the images U, corresponding to the f; they come from. You do not need to give a formal proof. Use your formula from (d) to give an exact answer for the fractal dimension of the attractor of this IFS. am IFS, Let { I ;w, wz,..., WN} be where I c Rm is closed and each wi is a similitude I I with scaling factor si Let W denote the Hutchinson operator associated to this IFS. Suppose that there exists an open set us I such that exists (A) W(U) SU and wilu) nw; (u) = 0, i7j Then the fractal dimension of the IFS's attractor A. and satisfies the equation. (!) E Isil 1 N TEL (!) E lsil? = 1 EL Remarks: When all the similitudes have the Same scaling fact sis, fact s;=s, isl,...,N, then (!) is easy to solve: D = ln (N) ln