From the following figure: Develop the following items: 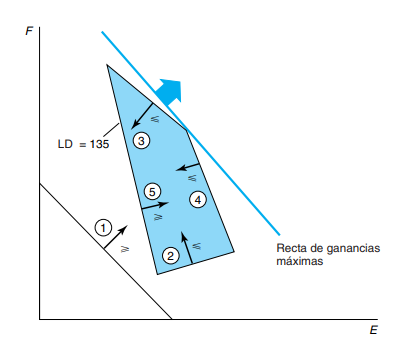(the image says: Maximum profit line) a. Consider Figure and suppose that the simplex algorithm was run at the vertex formed by the intersection of constraints 2 and 5. Demonstrate how a bottom-up algorithm eventually arrives safely at the optimal solution. b. On a piece of paper, draw a diagram like the one shown in Figure with a different feasible region for which a bottom-up algorithm cannot guarantee an optimal or even satisfactory solution. Would it be possible for a feasible region like the one you have created to exist in a linear programming model? That is, could you create a similar region using GLP (Graphic linear programming)? c. On a piece of paper, draw a diagram like Figure to show a situation in which a bottom-up algorithm might require many steps, or only a few, on its path to the optimal solution. d. Now consider a new diagram corresponding to a general (i.e., nonlinear) model with a single decision variable. Plotting the value of the decision variable on the x-axis and the value of the objective function on the y-axis, use this diagram to illustrate the fact that an optimal solution is not always obtained with a general promotion algorithm like the one described above. Answer step by step very well explained. If you do not know the answer, do not answer. If you are not going to answer all the items, do not answer.