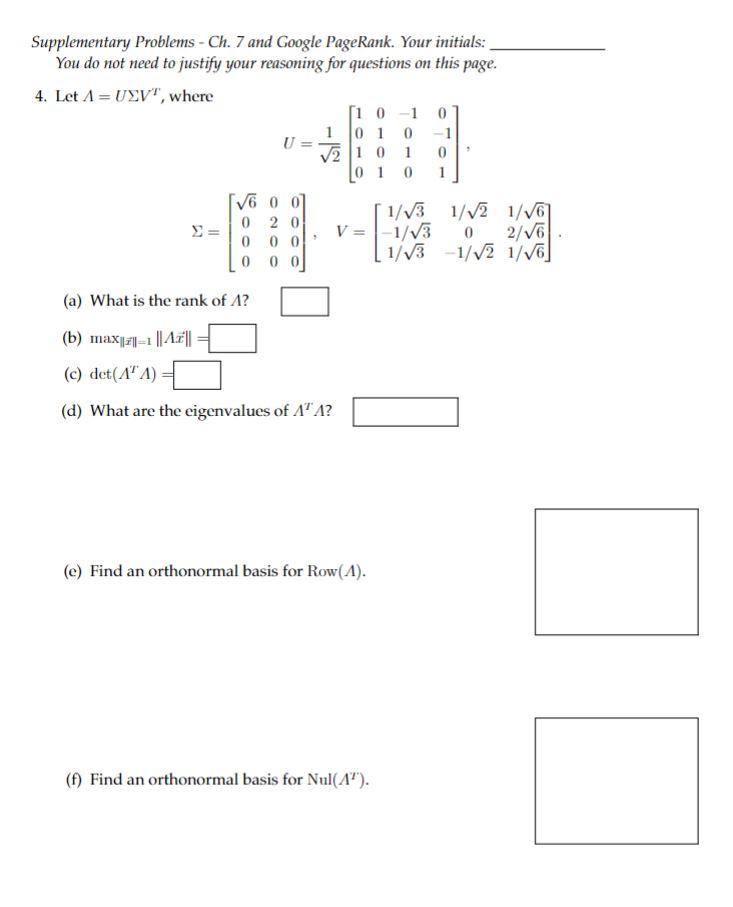
Give an example of a symmetric 3 x 3 matrix A such that: the corresponding quadratic form Q(z) = A is negative semi-definite and has no cross product terms; the range of the corresponding linear transformation T(7) = Az is 2-dimensional; and one of the eigenvalues of A has algebraic multiplicity equal to 2. A Find the minimum value of Q(z) = 4, on the unit sphere in R. Supplementary Problems - Ch. 7 and Google PageRank. Your initials: You do not need to justify your reasoning for questions on this page. 4. Let A = UEV, where = U 60 0 020 000 00 0 [10-1 0 01 21 0 1 01 0 (a) What is the rank of A? (b) max||||1||AZ|| (c) det(AA) (d) What are the eigenvalues of A A? V = -1/3 (e) Find an orthonormal basis for Row(4). 1/3 1/2 1/6] 0 2/6. 1/3 -1/2 1/6] (f) Find an orthonormal basis for Nul(A).