
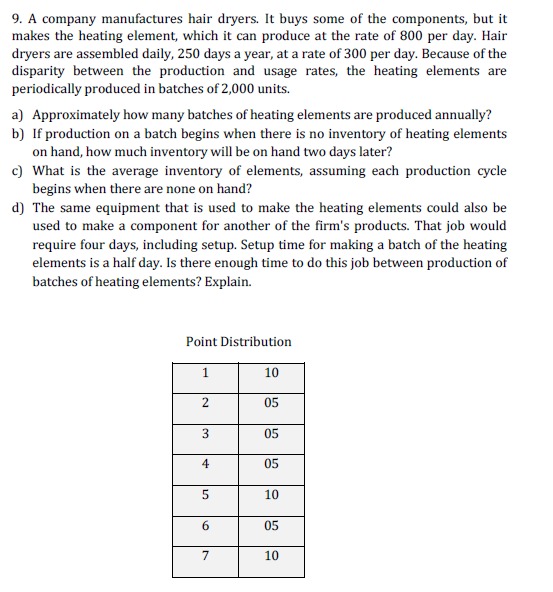


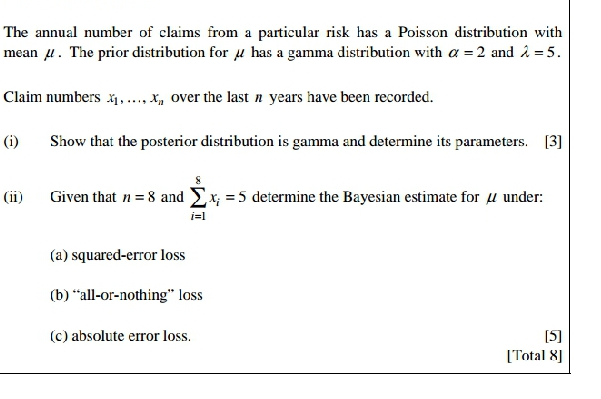
Give clear answers.
6. Write down consumption per worker, G, as a function of k, and the savings rate. Determine the Golden Rule level of the savings rate in this economy. (6 points) Solution. C = (1-8) kp. At the steady state, we have c* = (1 -8) 8 + gN Taking log: In c' = -7 1 - a - In (8 + gN ) + In (1 - s) + 1-a In s. Differentiating it w.r.t. s gives the following first order condition, 1 0 = - So s = 0. 7. Using a Solow diagram, show how the accumulation of capital per worker would change if there were an increase in the population growth rate, g. Provide the intuition. In another diagram, draw the evolution of consumption per worker over time after this change. (8 points) Solution. The increase in gy would shift up the required investment curve, resulting in a lower level of ke and ye in the steady state. Intuitively, the original level of investment is no longer sufficient to keep up with the growing population so capital per worker would fall. Since a = (1 - s) ye, it suffices to study the evolution of y. y = ky was at its steady state level and will change to its new steady state level which is lower. Hence, the evolution of consumption per worker decreases from (1 - s) (k*)" to (1 - s) (k*)". Make sure that the rate of change becomes slower and slower in your graph.9. A company manufactures hair dryers. It buys some of the components, but it makes the heating element, which it can produce at the rate of 800 per day. Hair dryers are assembled daily, 250 days a year, at a rate of 300 per day. Because of the disparity between the production and usage rates, the heating elements are periodically produced in batches of 2,000 units. a) Approximately how many batches of heating elements are produced annually? b) If production on a batch begins when there is no inventory of heating elements on hand, how much inventory will be on hand two days later? c) What is the average inventory of elements, assuming each production cycle begins when there are none on hand? d) The same equipment that is used to make the heating elements could also be used to make a component for another of the firm's products. That job would require four days, including setup. Setup time for making a batch of the heating elements is a half day. Is there enough time to do this job between production of batches of heating elements? Explain. Point Distribution 1 10 2 05 3 05 4 05 5 10 6 05 7 104) What makes a secondary source "academic" or "scholarly" such that you could actually use it in a research essay? See pp. 1956-1957. 5) Whenever you want to summarize, paraphrase, or quote from a secondary source, you must introduce that source. Apply the methods discussed on pp. 1960-1961 to the following and introduce this as a source in an imaginary essay. Author of Article: Keith Byerman Title of Article: "Anger in a Small Place: Jamaica Kincaid's Cultural Critique of Antigua" Argument of Article: In exploring Jamaica Kincaid's corpus of works, it is quite obvious that she confronts the problems that colonialism has created on the island of Antigua. What may be less apparent is that her writing also challenges traditional Antiguan culture and does so in gendered terms: she examines and critiques the social effects of sharply divided gender roles.There are two friend, Quick and Slow, taking tough Econ 301 course. Both need to decide whether to do assignment themselves or wait until just it is due and the copy what the other has done. Quick finds the material easy and will get 8 out of 10 questions correct if he does the work while Slow will get only 5 out of 10. If both wait, hoping to copy the other's work, they will both get zero. (a) Write out this game in its strategic form. (b) Do either have the dominant strategy, and if so, what they are? (c) What outcome would you expect. Explain.The annual number of claims from a particular risk has a Poisson distribution with mean /. The prior distribution for / has a gamma distribution with o =2 and 1 =5. Claim numbers M. .... x, over the last n years have been recorded. (i) Show that the posterior distribution is gamma and determine its parameters. [3] (ii) Given that n = 8 and ) x; =5 determine the Bayesian estimate for // under: i=1 (a) squared-error loss (b) "all-or-nothing" loss (c) absolute error loss. [5] [Total X]The annual number of claims from a particular risk has a Poisson distribution with mean /. The prior distribution for / has a gamma distribution with o =2 and 1 =5. Claim numbers M. .... x, over the last n years have been recorded. (i) Show that the posterior distribution is gamma and determine its parameters. [3] (ii) Given that n = 8 and ) x; =5 determine the Bayesian estimate for // under: i=1 (a) squared-error loss (b) "all-or-nothing" loss (c) absolute error loss. [5] [Total X]
















