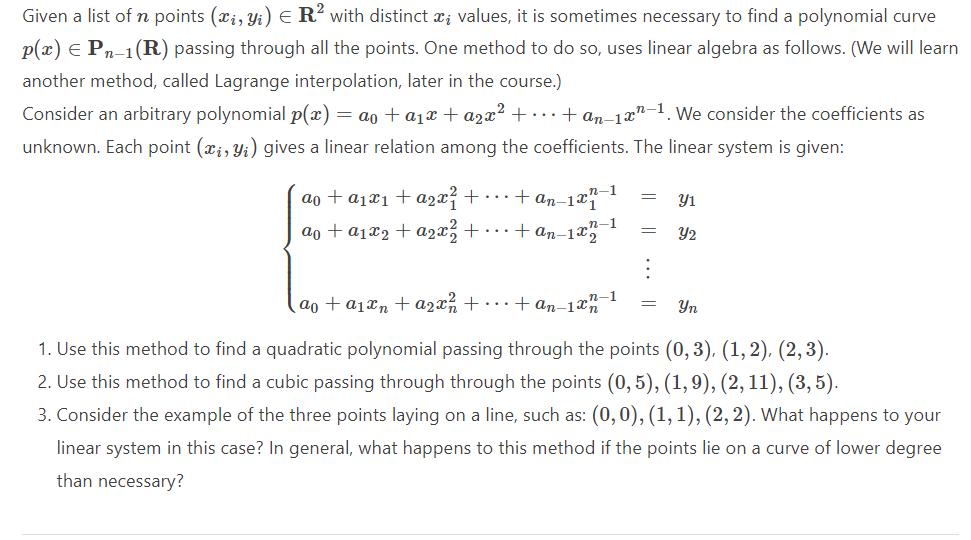
Given a list of n points (xi, yi) ER with distinct a values, it is sometimes necessary to find a polynomial curve p(x) Pn-1(R) passing through all the points. One method to do so, uses linear algebra as follows. (We will learn another method, called Lagrange interpolation, later in the course.) Consider an arbitrary polynomial p(x) = a + a1x+a2x + ... + an-1x-1. We consider the coefficients as unknown. Each point (xi, yi) gives a linear relation among the coefficients. The linear system is given: ao+a1x1 + a2x + + an- n-1 = Y1 90+ a1x2+2x+ ... +an-1x n-1 = Y2 ao+axn+a2x + + an-1xn1 Yn 1. Use this method to find a quadratic polynomial passing through the points (0,3), (1, 2), (2, 3). 2. Use this method to find a cubic passing through through the points (0,5), (1, 9), (2, 11), (3, 5). 3. Consider the example of the three points laying on a line, such as: (0,0), (1, 1), (2, 2). What happens to your linear system in this case? In general, what happens to this method if the points lie on a curve of lower degree than necessary?