Answered step by step
Verified Expert Solution
Question
1 Approved Answer
Given the following information: Fill in the yellow blanks below: begin{tabular}{|c|c|c|c|c|c|c|c|c|c|c|c|c|c|} hline multirow{2}{*}{begin{tabular}{l} 1 2 end{tabular}} & multicolumn{3}{|l|}{ Hedging DGAP and EVE } &
Given the following information:
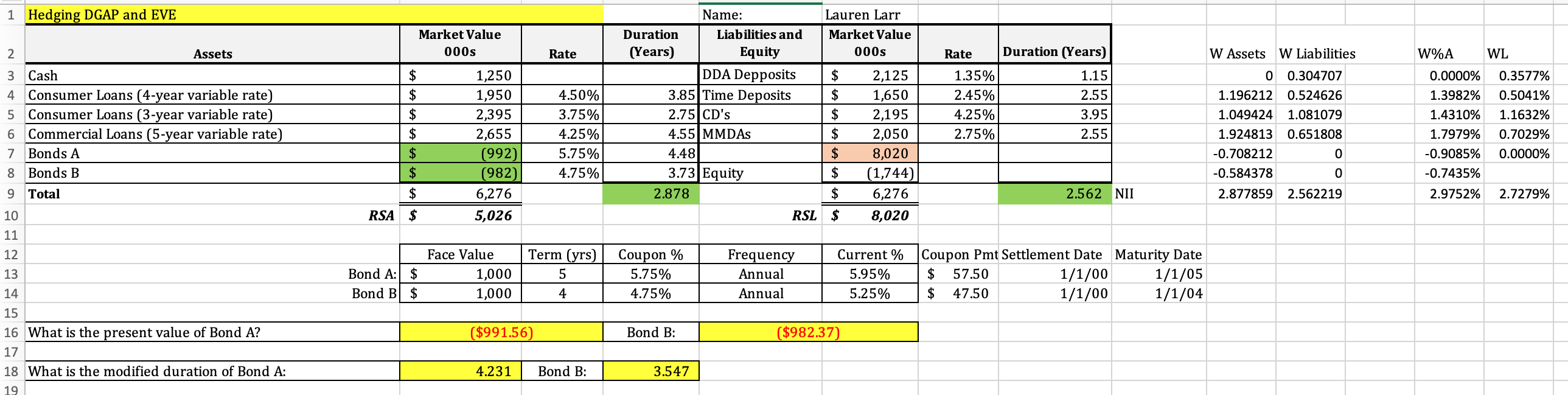
Fill in the yellow blanks below:
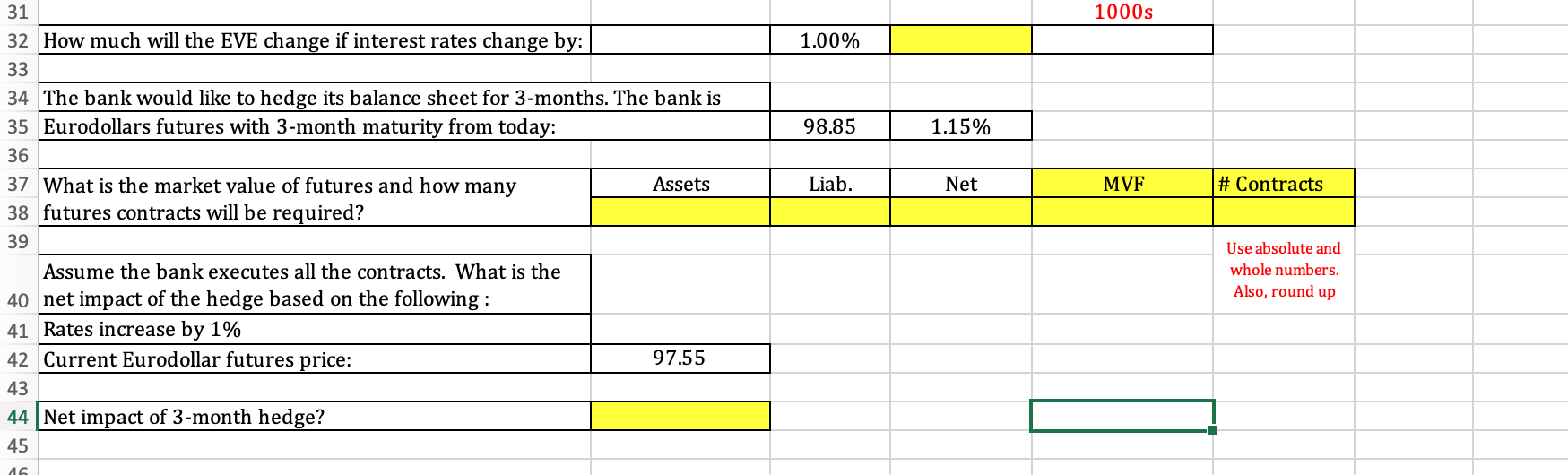 \begin{tabular}{|c|c|c|c|c|c|c|c|c|c|c|c|c|c|} \hline \multirow{2}{*}{\begin{tabular}{l} 1 \\ 2 \end{tabular}} & \multicolumn{3}{|l|}{ Hedging DGAP and EVE } & \multicolumn{2}{|r|}{ Name: } & Lauren Larr & \multirow[b]{2}{*}{ Rate } & \multirow[b]{2}{*}{ Duration (Years) } & & \multirow[b]{2}{*}{ W Assets } & \multirow[b]{2}{*}{ W Liabilities } & \multirow[b]{2}{*}{ W\%A } & \multirow[b]{2}{*}{ WL } \\ \hline & Assets & \begin{tabular}{c} Market Value \\ 000s \end{tabular} & Rate & \begin{tabular}{c} Duration \\ (Years) \end{tabular} & \begin{tabular}{c} Liabilities and \\ Equity \end{tabular} & \begin{tabular}{|c|} Market Value \\ 000s \\ \end{tabular} & & & & & & & \\ \hline 3 & Cash & 1,250 & & & DDA Depposits & 2,125 & 1.35% & 1.15 & & 0 & 0.304707 & 0.0000% & 0.3577% \\ \hline 4 & Consumer Loans (4-year variable rate) & 1,950 & 4.50% & 3.85 & Time Deposits & 1,650 & 2.45% & 2.55 & & 1.196212 & 0.524626 & 1.3982% & 0.5041% \\ \hline 5 & Consumer Loans (3-year variable rate) & 2,395 & 3.75% & 2.75 & CD's & 2,195 & 4.25% & 3.95 & & 1.049424 & 1.081079 & 1.4310% & 1.1632% \\ \hline 6 & Commercial Loans (5-year variable rate) & 2,655 & 4.25% & 4.55 & MMDAs & 2,050 & 2.75% & 2.55 & & 1.924813 & 0.651808 & 1.7979% & 0.7029% \\ \hline 7 & Bonds A & (992) & 5.75% & 4.48 & & 8,020 & & & & -0.708212 & 0 & 0.9085% & 0.0000% \\ \hline 8 & Bonds B & (982) & 4.75% & 3.73 & Equity & (1,744) & & & & -0.584378 & 0 & 0.7435% & \\ \hline 9 & Total & 6,276 & & 2.878 & & 6,276 & & 2.562 & NII & 2.877859 & 2.562219 & 2.9752% & 2.7279% \\ \hline 10 & RSA & 5,026 & & & RSL & 8,020 & & & & & & & \\ \hline 11 & & & & & & & & & & & & & \\ \hline 12 & & Face Value & Term (yrs) & Coupon \% & Frequency & Current \% & Coupon Pmt & Settlement Date & Maturity Date & & & & \\ \hline 13 & Bond A: & 1,000 & 5 & 5.75% & Annual & 5.95% & $57.50 & 1/1/00 & 1/1/05 & & & & \\ \hline 14 & Bond B & 1,000 & 4 & 4.75% & Annual & 5.25% & $47.50 & 1/1/00 & 1/1/04 & & & & \\ \hline 15 & & & & & & & & & & & & & \\ \hline 16 & What is the present value of Bond A? & ($991.56 & & Bond B: & ($982.3 & & & & & & & & \\ \hline 17 & & & & & & & & & & & & & \\ \hline 18 & What is the modified duration of Bond A: & 4.231 & Bond B: & 3.547 & & & & & & & & & \\ \hline \end{tabular} \begin{tabular}{|c|c|c|c|c|c|c|} \hline 31 & & & & & 1000s & \\ \hline 32 & How much will the EVE change if interest rates change by: & & 1.00% & & & \\ \hline 33 & & & & & & \\ \hline 34 & \multicolumn{2}{|c|}{ The bank would like to hedge its balance sheet for 3 -months. The bank is } & & & & \\ \hline 35 & \multicolumn{2}{|l|}{ Eurodollars futures with 3-month maturity from today: } & 98.85 & 1.15% & & \\ \hline 36 & & & & & & \\ \hline 37 & \multirow{2}{*}{\begin{tabular}{l} What is the market value of futures and how many \\ futures contracts will be required? \end{tabular}} & Assets & Liab. & Net & MVF & \# Contracts \\ \hline 38 & & & & & & \\ \hline 39 & & & & & & \multirow{2}{*}{\begin{tabular}{l} Use absolute and \\ whole numbers. \\ Also, round up \end{tabular}} \\ \hline 40 & \begin{tabular}{l} Assume the bank executes all the contracts. What is the \\ net impact of the hedge based on the following: \end{tabular} & & & & & \\ \hline 41 & Rates increase by 1% & & & & & \\ \hline 42 & Current Eurodollar futures price: & 97.55 & & & & \\ \hline \multirow{2}{*}{\begin{tabular}{l} 43 \\ 44 \end{tabular}} & & & & & & \\ \hline & Net impact of 3-month hedge? & & & & & \\ \hline 45 & & & & & & \\ \hline \end{tabular} \begin{tabular}{|c|c|c|c|c|c|c|c|c|c|c|c|c|c|} \hline \multirow{2}{*}{\begin{tabular}{l} 1 \\ 2 \end{tabular}} & \multicolumn{3}{|l|}{ Hedging DGAP and EVE } & \multicolumn{2}{|r|}{ Name: } & Lauren Larr & \multirow[b]{2}{*}{ Rate } & \multirow[b]{2}{*}{ Duration (Years) } & & \multirow[b]{2}{*}{ W Assets } & \multirow[b]{2}{*}{ W Liabilities } & \multirow[b]{2}{*}{ W\%A } & \multirow[b]{2}{*}{ WL } \\ \hline & Assets & \begin{tabular}{c} Market Value \\ 000s \end{tabular} & Rate & \begin{tabular}{c} Duration \\ (Years) \end{tabular} & \begin{tabular}{c} Liabilities and \\ Equity \end{tabular} & \begin{tabular}{|c|} Market Value \\ 000s \\ \end{tabular} & & & & & & & \\ \hline 3 & Cash & 1,250 & & & DDA Depposits & 2,125 & 1.35% & 1.15 & & 0 & 0.304707 & 0.0000% & 0.3577% \\ \hline 4 & Consumer Loans (4-year variable rate) & 1,950 & 4.50% & 3.85 & Time Deposits & 1,650 & 2.45% & 2.55 & & 1.196212 & 0.524626 & 1.3982% & 0.5041% \\ \hline 5 & Consumer Loans (3-year variable rate) & 2,395 & 3.75% & 2.75 & CD's & 2,195 & 4.25% & 3.95 & & 1.049424 & 1.081079 & 1.4310% & 1.1632% \\ \hline 6 & Commercial Loans (5-year variable rate) & 2,655 & 4.25% & 4.55 & MMDAs & 2,050 & 2.75% & 2.55 & & 1.924813 & 0.651808 & 1.7979% & 0.7029% \\ \hline 7 & Bonds A & (992) & 5.75% & 4.48 & & 8,020 & & & & -0.708212 & 0 & 0.9085% & 0.0000% \\ \hline 8 & Bonds B & (982) & 4.75% & 3.73 & Equity & (1,744) & & & & -0.584378 & 0 & 0.7435% & \\ \hline 9 & Total & 6,276 & & 2.878 & & 6,276 & & 2.562 & NII & 2.877859 & 2.562219 & 2.9752% & 2.7279% \\ \hline 10 & RSA & 5,026 & & & RSL & 8,020 & & & & & & & \\ \hline 11 & & & & & & & & & & & & & \\ \hline 12 & & Face Value & Term (yrs) & Coupon \% & Frequency & Current \% & Coupon Pmt & Settlement Date & Maturity Date & & & & \\ \hline 13 & Bond A: & 1,000 & 5 & 5.75% & Annual & 5.95% & $57.50 & 1/1/00 & 1/1/05 & & & & \\ \hline 14 & Bond B & 1,000 & 4 & 4.75% & Annual & 5.25% & $47.50 & 1/1/00 & 1/1/04 & & & & \\ \hline 15 & & & & & & & & & & & & & \\ \hline 16 & What is the present value of Bond A? & ($991.56 & & Bond B: & ($982.3 & & & & & & & & \\ \hline 17 & & & & & & & & & & & & & \\ \hline 18 & What is the modified duration of Bond A: & 4.231 & Bond B: & 3.547 & & & & & & & & & \\ \hline \end{tabular} \begin{tabular}{|c|c|c|c|c|c|c|} \hline 31 & & & & & 1000s & \\ \hline 32 & How much will the EVE change if interest rates change by: & & 1.00% & & & \\ \hline 33 & & & & & & \\ \hline 34 & \multicolumn{2}{|c|}{ The bank would like to hedge its balance sheet for 3 -months. The bank is } & & & & \\ \hline 35 & \multicolumn{2}{|l|}{ Eurodollars futures with 3-month maturity from today: } & 98.85 & 1.15% & & \\ \hline 36 & & & & & & \\ \hline 37 & \multirow{2}{*}{\begin{tabular}{l} What is the market value of futures and how many \\ futures contracts will be required? \end{tabular}} & Assets & Liab. & Net & MVF & \# Contracts \\ \hline 38 & & & & & & \\ \hline 39 & & & & & & \multirow{2}{*}{\begin{tabular}{l} Use absolute and \\ whole numbers. \\ Also, round up \end{tabular}} \\ \hline 40 & \begin{tabular}{l} Assume the bank executes all the contracts. What is the \\ net impact of the hedge based on the following: \end{tabular} & & & & & \\ \hline 41 & Rates increase by 1% & & & & & \\ \hline 42 & Current Eurodollar futures price: & 97.55 & & & & \\ \hline \multirow{2}{*}{\begin{tabular}{l} 43 \\ 44 \end{tabular}} & & & & & & \\ \hline & Net impact of 3-month hedge? & & & & & \\ \hline 45 & & & & & & \\ \hline \end{tabular}
\begin{tabular}{|c|c|c|c|c|c|c|c|c|c|c|c|c|c|} \hline \multirow{2}{*}{\begin{tabular}{l} 1 \\ 2 \end{tabular}} & \multicolumn{3}{|l|}{ Hedging DGAP and EVE } & \multicolumn{2}{|r|}{ Name: } & Lauren Larr & \multirow[b]{2}{*}{ Rate } & \multirow[b]{2}{*}{ Duration (Years) } & & \multirow[b]{2}{*}{ W Assets } & \multirow[b]{2}{*}{ W Liabilities } & \multirow[b]{2}{*}{ W\%A } & \multirow[b]{2}{*}{ WL } \\ \hline & Assets & \begin{tabular}{c} Market Value \\ 000s \end{tabular} & Rate & \begin{tabular}{c} Duration \\ (Years) \end{tabular} & \begin{tabular}{c} Liabilities and \\ Equity \end{tabular} & \begin{tabular}{|c|} Market Value \\ 000s \\ \end{tabular} & & & & & & & \\ \hline 3 & Cash & 1,250 & & & DDA Depposits & 2,125 & 1.35% & 1.15 & & 0 & 0.304707 & 0.0000% & 0.3577% \\ \hline 4 & Consumer Loans (4-year variable rate) & 1,950 & 4.50% & 3.85 & Time Deposits & 1,650 & 2.45% & 2.55 & & 1.196212 & 0.524626 & 1.3982% & 0.5041% \\ \hline 5 & Consumer Loans (3-year variable rate) & 2,395 & 3.75% & 2.75 & CD's & 2,195 & 4.25% & 3.95 & & 1.049424 & 1.081079 & 1.4310% & 1.1632% \\ \hline 6 & Commercial Loans (5-year variable rate) & 2,655 & 4.25% & 4.55 & MMDAs & 2,050 & 2.75% & 2.55 & & 1.924813 & 0.651808 & 1.7979% & 0.7029% \\ \hline 7 & Bonds A & (992) & 5.75% & 4.48 & & 8,020 & & & & -0.708212 & 0 & 0.9085% & 0.0000% \\ \hline 8 & Bonds B & (982) & 4.75% & 3.73 & Equity & (1,744) & & & & -0.584378 & 0 & 0.7435% & \\ \hline 9 & Total & 6,276 & & 2.878 & & 6,276 & & 2.562 & NII & 2.877859 & 2.562219 & 2.9752% & 2.7279% \\ \hline 10 & RSA & 5,026 & & & RSL & 8,020 & & & & & & & \\ \hline 11 & & & & & & & & & & & & & \\ \hline 12 & & Face Value & Term (yrs) & Coupon \% & Frequency & Current \% & Coupon Pmt & Settlement Date & Maturity Date & & & & \\ \hline 13 & Bond A: & 1,000 & 5 & 5.75% & Annual & 5.95% & $57.50 & 1/1/00 & 1/1/05 & & & & \\ \hline 14 & Bond B & 1,000 & 4 & 4.75% & Annual & 5.25% & $47.50 & 1/1/00 & 1/1/04 & & & & \\ \hline 15 & & & & & & & & & & & & & \\ \hline 16 & What is the present value of Bond A? & ($991.56 & & Bond B: & ($982.3 & & & & & & & & \\ \hline 17 & & & & & & & & & & & & & \\ \hline 18 & What is the modified duration of Bond A: & 4.231 & Bond B: & 3.547 & & & & & & & & & \\ \hline \end{tabular} \begin{tabular}{|c|c|c|c|c|c|c|} \hline 31 & & & & & 1000s & \\ \hline 32 & How much will the EVE change if interest rates change by: & & 1.00% & & & \\ \hline 33 & & & & & & \\ \hline 34 & \multicolumn{2}{|c|}{ The bank would like to hedge its balance sheet for 3 -months. The bank is } & & & & \\ \hline 35 & \multicolumn{2}{|l|}{ Eurodollars futures with 3-month maturity from today: } & 98.85 & 1.15% & & \\ \hline 36 & & & & & & \\ \hline 37 & \multirow{2}{*}{\begin{tabular}{l} What is the market value of futures and how many \\ futures contracts will be required? \end{tabular}} & Assets & Liab. & Net & MVF & \# Contracts \\ \hline 38 & & & & & & \\ \hline 39 & & & & & & \multirow{2}{*}{\begin{tabular}{l} Use absolute and \\ whole numbers. \\ Also, round up \end{tabular}} \\ \hline 40 & \begin{tabular}{l} Assume the bank executes all the contracts. What is the \\ net impact of the hedge based on the following: \end{tabular} & & & & & \\ \hline 41 & Rates increase by 1% & & & & & \\ \hline 42 & Current Eurodollar futures price: & 97.55 & & & & \\ \hline \multirow{2}{*}{\begin{tabular}{l} 43 \\ 44 \end{tabular}} & & & & & & \\ \hline & Net impact of 3-month hedge? & & & & & \\ \hline 45 & & & & & & \\ \hline \end{tabular} \begin{tabular}{|c|c|c|c|c|c|c|c|c|c|c|c|c|c|} \hline \multirow{2}{*}{\begin{tabular}{l} 1 \\ 2 \end{tabular}} & \multicolumn{3}{|l|}{ Hedging DGAP and EVE } & \multicolumn{2}{|r|}{ Name: } & Lauren Larr & \multirow[b]{2}{*}{ Rate } & \multirow[b]{2}{*}{ Duration (Years) } & & \multirow[b]{2}{*}{ W Assets } & \multirow[b]{2}{*}{ W Liabilities } & \multirow[b]{2}{*}{ W\%A } & \multirow[b]{2}{*}{ WL } \\ \hline & Assets & \begin{tabular}{c} Market Value \\ 000s \end{tabular} & Rate & \begin{tabular}{c} Duration \\ (Years) \end{tabular} & \begin{tabular}{c} Liabilities and \\ Equity \end{tabular} & \begin{tabular}{|c|} Market Value \\ 000s \\ \end{tabular} & & & & & & & \\ \hline 3 & Cash & 1,250 & & & DDA Depposits & 2,125 & 1.35% & 1.15 & & 0 & 0.304707 & 0.0000% & 0.3577% \\ \hline 4 & Consumer Loans (4-year variable rate) & 1,950 & 4.50% & 3.85 & Time Deposits & 1,650 & 2.45% & 2.55 & & 1.196212 & 0.524626 & 1.3982% & 0.5041% \\ \hline 5 & Consumer Loans (3-year variable rate) & 2,395 & 3.75% & 2.75 & CD's & 2,195 & 4.25% & 3.95 & & 1.049424 & 1.081079 & 1.4310% & 1.1632% \\ \hline 6 & Commercial Loans (5-year variable rate) & 2,655 & 4.25% & 4.55 & MMDAs & 2,050 & 2.75% & 2.55 & & 1.924813 & 0.651808 & 1.7979% & 0.7029% \\ \hline 7 & Bonds A & (992) & 5.75% & 4.48 & & 8,020 & & & & -0.708212 & 0 & 0.9085% & 0.0000% \\ \hline 8 & Bonds B & (982) & 4.75% & 3.73 & Equity & (1,744) & & & & -0.584378 & 0 & 0.7435% & \\ \hline 9 & Total & 6,276 & & 2.878 & & 6,276 & & 2.562 & NII & 2.877859 & 2.562219 & 2.9752% & 2.7279% \\ \hline 10 & RSA & 5,026 & & & RSL & 8,020 & & & & & & & \\ \hline 11 & & & & & & & & & & & & & \\ \hline 12 & & Face Value & Term (yrs) & Coupon \% & Frequency & Current \% & Coupon Pmt & Settlement Date & Maturity Date & & & & \\ \hline 13 & Bond A: & 1,000 & 5 & 5.75% & Annual & 5.95% & $57.50 & 1/1/00 & 1/1/05 & & & & \\ \hline 14 & Bond B & 1,000 & 4 & 4.75% & Annual & 5.25% & $47.50 & 1/1/00 & 1/1/04 & & & & \\ \hline 15 & & & & & & & & & & & & & \\ \hline 16 & What is the present value of Bond A? & ($991.56 & & Bond B: & ($982.3 & & & & & & & & \\ \hline 17 & & & & & & & & & & & & & \\ \hline 18 & What is the modified duration of Bond A: & 4.231 & Bond B: & 3.547 & & & & & & & & & \\ \hline \end{tabular} \begin{tabular}{|c|c|c|c|c|c|c|} \hline 31 & & & & & 1000s & \\ \hline 32 & How much will the EVE change if interest rates change by: & & 1.00% & & & \\ \hline 33 & & & & & & \\ \hline 34 & \multicolumn{2}{|c|}{ The bank would like to hedge its balance sheet for 3 -months. The bank is } & & & & \\ \hline 35 & \multicolumn{2}{|l|}{ Eurodollars futures with 3-month maturity from today: } & 98.85 & 1.15% & & \\ \hline 36 & & & & & & \\ \hline 37 & \multirow{2}{*}{\begin{tabular}{l} What is the market value of futures and how many \\ futures contracts will be required? \end{tabular}} & Assets & Liab. & Net & MVF & \# Contracts \\ \hline 38 & & & & & & \\ \hline 39 & & & & & & \multirow{2}{*}{\begin{tabular}{l} Use absolute and \\ whole numbers. \\ Also, round up \end{tabular}} \\ \hline 40 & \begin{tabular}{l} Assume the bank executes all the contracts. What is the \\ net impact of the hedge based on the following: \end{tabular} & & & & & \\ \hline 41 & Rates increase by 1% & & & & & \\ \hline 42 & Current Eurodollar futures price: & 97.55 & & & & \\ \hline \multirow{2}{*}{\begin{tabular}{l} 43 \\ 44 \end{tabular}} & & & & & & \\ \hline & Net impact of 3-month hedge? & & & & & \\ \hline 45 & & & & & & \\ \hline \end{tabular} Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


