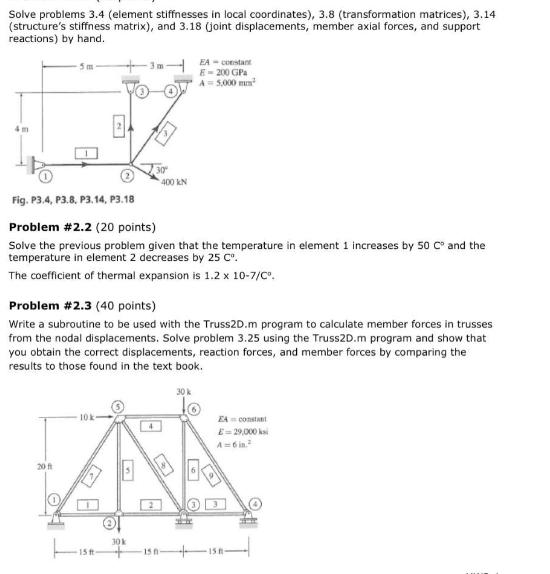
Solve problems 3.4 (element stiffnesses in local coordinates), 3.8 (transformation matrices), 3.14 (structure's stiffness matrix), and 3.18 (joint displacements, member axial forces, and support reactions) by hand. 4m Fig. P3.4, P3.8, P3.14, P3.18 Problem #2.2 (20 points) Solve the previous problem given that the temperature in element 1 increases by 50 C and the temperature in element 2 decreases by 25 C. The coefficient of thermal expansion is 1.2 x 10-7/C. 30 400 KN Problem #2.3 (40 points) Write a subroutine to be used with the Truss2D.m program to calculate member forces in trusses from the nodal displacements. Solve problem 3.25 using the Truss2D.m program and show that you obtain the correct displacements, reaction forces, and member forces by comparing the results to those found in the text book. 20 ft EA- constant E-200 GPa A=5,000 m 30 k AN -15 n +15- 15 ft 30k EA consta E=29,000 k