Hart Manufacturing possesses three plants and is considering four possible new products. Each new product can be produced at each plant. The labor-hour requirement per unit of each new product at each plant (hours per unit) is provided in the following table:

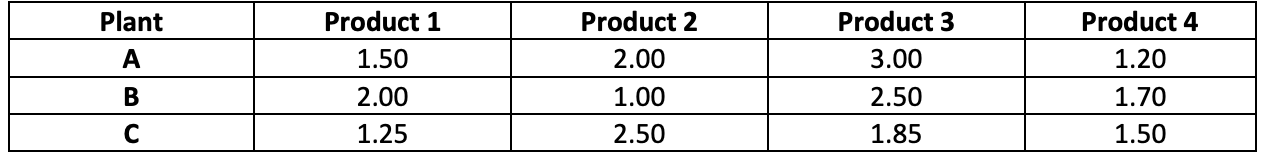During the next production period, the labor hours available are 450 hours at Plant A, 350 hours at Plant B, and 500 hours at Plant C. The fixed setup costs are $400 for Plant A, $550 for Plant B, and $600 for Plant C. For administrative reasons, only one plant should be chosen to be the sole producer of the new products.

At most three new products should be selected to be produced. In addition, Hart Manufacturing doesnt want to produce any units of each product unless its minimum production requirement is satisfied. The profit contribution per unit and the minimum production requirement of each product are provided in the following table:

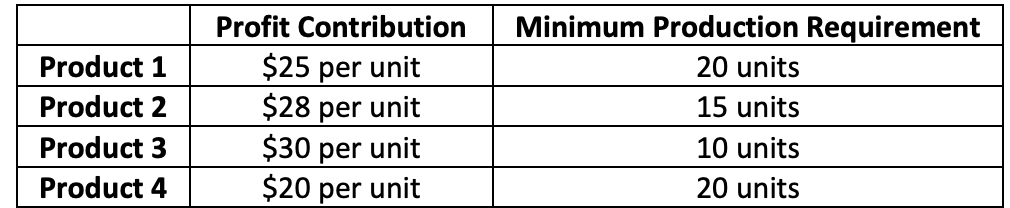
Please formulate a mixed-integer linear program that maximizes the total profit and takes setup costs into account.

If arbitrarily large positive values (s) are in the model formulation, please specify how to determine these values. Please remember that:

The value should be large enough to avoid eliminating any feasible solutions.

It should also be as small as possible, in order to avoid unduly enlarging the feasible region.

[The explicit model formulation is fine; it is also fine if you prefer the generic model formulation. But please provide clear definitions of all the notations in the model. NO need to solve the model.]

Plant Product 2 2.00 A Product 1 1.50 2.00 1.25 Product 3 3.00 2.50 1.85 Product 4 1.20 1.70 B 1.00 2.50 C 1.50 Product 1 Product 2 Product 3 Product 4 Profit Contribution $25 per unit $28 per unit $30 per unit $20 per unit Minimum Production Requirement 20 units 15 units 10 units 20 units Plant Product 2 2.00 A Product 1 1.50 2.00 1.25 Product 3 3.00 2.50 1.85 Product 4 1.20 1.70 B 1.00 2.50 C 1.50 Product 1 Product 2 Product 3 Product 4 Profit Contribution $25 per unit $28 per unit $30 per unit $20 per unit Minimum Production Requirement 20 units 15 units 10 units 20 units