Haskell GHCI: Tail recursion and Datatypes

PLEASE TRY TO ANSWER IT THIS TIME AS BEST POSSIBLE.

Example for BINARY TREE DEFINITION from lecture

Recursive Structures (Example)

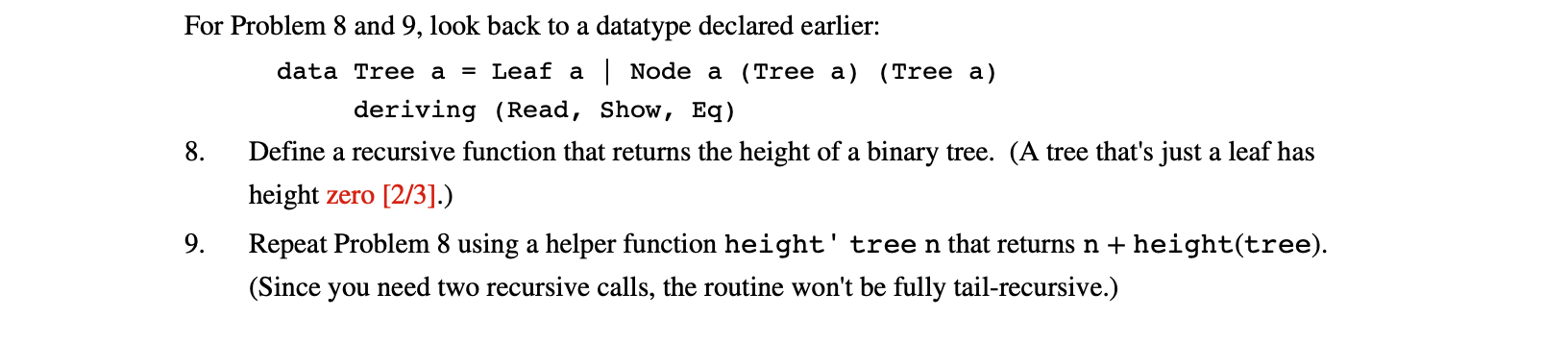
For Problems 3 and 4, use the following binary tree definition modified from lecture: It takes two type arguments, so nodes and leafs can contain different types of values. data Tree a b = Leaf b | Node a (Tree a b) (Tree a b) deriving (Read, Show, Eq) (12 points) For this problem, let's call an expression tree a Tree String b tree where all of the node data are strings from the set "+", "-", "*", and "/", and the leafs hold numbers. Write an eval function routine that evaluates an expression tree. Division requires fractional numbers, so the type of eval is Fractional t => Tree String b-> b. Examples: Let el = Node "+" (Leaf 2) (Leaf 4), e2 = Node "-" (Leaf 11) (Leaf 8), and e3 = Node "/" (Node "*" el e2) (Leaf 36). Then eval el = 6.0; eval e2 = 3.0; and eval e3 = 0.5. For Problem 8 and 9, look back to a datatype declared earlier: data Tree a = Leaf a | Node a (Tree a) (Tree a) deriving (Read, Show, Eq) 8. Define a recursive function that returns the height of a binary tree. (A tree that's just a leaf has height zero [2/3].) 9. Repeat Problem 8 using a helper function height' tree n that returns n + height(tree). (Since you need two recursive calls, the routine won't be fully tail-recursive.) For Problems 8 and 9, we have data Tree a = Leaf a | Node a (Tree a) (Tree a) deriving (Read, Show, Eq) I'll name the functions height1 and height2 just to make them different 8. heighti (Leaf _) = 0 -- leafs have height 0 not i heightl (Node left right) = 1 + max (height left) (heightl right) 9. height2 tree = height' tree o height' (Leaf) h = h -- height' tree n = height of tree + n height' (Node _ left right) h = max (height' left (h+1)) (height' right (h+1)) (There isn't much difference between the two definitions, since we need two recursive calls and we're doing work after the calls.) H. Recursive Structures If the body of a data declaration uses the type being defined, we get a recursive datatype. :{ | data List a = Node a (List a) | Nil deriving (Show, Read, Eq) | null Nil = True | null (Node _ _) = False | len Nil = 0 | len (Node_ x) = 1 + len x | lenl x = lenl' 0 x | lenl' res Nil = res | lenl' res (Node - t) = lenl' (res+1) t :} > x = Node 1 (Node 2 (Node 3 (Node 4 (Node 5 Nil)))) > len x > lenl x For another example of a recursive datatype, here's a simple binary tree type, where the nodes and leafs are labeled by values. > data Tree a = Leaf a Node a (Tree a) (Tree a) deriving (Read, Show, Eq) > t1 = Leaf "abc". > t2 = Node "ab" ti (Leaf "de") -- using ti, a leaf >:t ti ti :: Tree (Char] > it t2 t2 :: Tree (Char] Here's a function that checks to see if a given value is every value in the tree. (Note we can only run this function on trees where the values are of a type that supports Ord.) :{ | ge_tree :: Ord t => t -> Tree t -> Bool | ge_tree val (Leaf val') = val >= val' | ge_tree val (Node val' ti t2) = val >= val' && ge tree val tl && ge tree val t2 > t2 Node "ab" (Leaf "abc") (Leaf "de") For Problems 3 and 4, use the following binary tree definition modified from lecture: It takes two type arguments, so nodes and leafs can contain different types of values. data Tree a b = Leaf b | Node a (Tree a b) (Tree a b) deriving (Read, Show, Eq) (12 points) For this problem, let's call an expression tree a Tree String b tree where all of the node data are strings from the set "+", "-", "*", and "/", and the leafs hold numbers. Write an eval function routine that evaluates an expression tree. Division requires fractional numbers, so the type of eval is Fractional t => Tree String b-> b. Examples: Let el = Node "+" (Leaf 2) (Leaf 4), e2 = Node "-" (Leaf 11) (Leaf 8), and e3 = Node "/" (Node "*" el e2) (Leaf 36). Then eval el = 6.0; eval e2 = 3.0; and eval e3 = 0.5. For Problem 8 and 9, look back to a datatype declared earlier: data Tree a = Leaf a | Node a (Tree a) (Tree a) deriving (Read, Show, Eq) 8. Define a recursive function that returns the height of a binary tree. (A tree that's just a leaf has height zero [2/3].) 9. Repeat Problem 8 using a helper function height' tree n that returns n + height(tree). (Since you need two recursive calls, the routine won't be fully tail-recursive.) For Problems 8 and 9, we have data Tree a = Leaf a | Node a (Tree a) (Tree a) deriving (Read, Show, Eq) I'll name the functions height1 and height2 just to make them different 8. heighti (Leaf _) = 0 -- leafs have height 0 not i heightl (Node left right) = 1 + max (height left) (heightl right) 9. height2 tree = height' tree o height' (Leaf) h = h -- height' tree n = height of tree + n height' (Node _ left right) h = max (height' left (h+1)) (height' right (h+1)) (There isn't much difference between the two definitions, since we need two recursive calls and we're doing work after the calls.) H. Recursive Structures If the body of a data declaration uses the type being defined, we get a recursive datatype. :{ | data List a = Node a (List a) | Nil deriving (Show, Read, Eq) | null Nil = True | null (Node _ _) = False | len Nil = 0 | len (Node_ x) = 1 + len x | lenl x = lenl' 0 x | lenl' res Nil = res | lenl' res (Node - t) = lenl' (res+1) t :} > x = Node 1 (Node 2 (Node 3 (Node 4 (Node 5 Nil)))) > len x > lenl x For another example of a recursive datatype, here's a simple binary tree type, where the nodes and leafs are labeled by values. > data Tree a = Leaf a Node a (Tree a) (Tree a) deriving (Read, Show, Eq) > t1 = Leaf "abc". > t2 = Node "ab" ti (Leaf "de") -- using ti, a leaf >:t ti ti :: Tree (Char] > it t2 t2 :: Tree (Char] Here's a function that checks to see if a given value is every value in the tree. (Note we can only run this function on trees where the values are of a type that supports Ord.) :{ | ge_tree :: Ord t => t -> Tree t -> Bool | ge_tree val (Leaf val') = val >= val' | ge_tree val (Node val' ti t2) = val >= val' && ge tree val tl && ge tree val t2 > t2 Node "ab" (Leaf "abc") (Leaf "de")