Hello dear tutors,

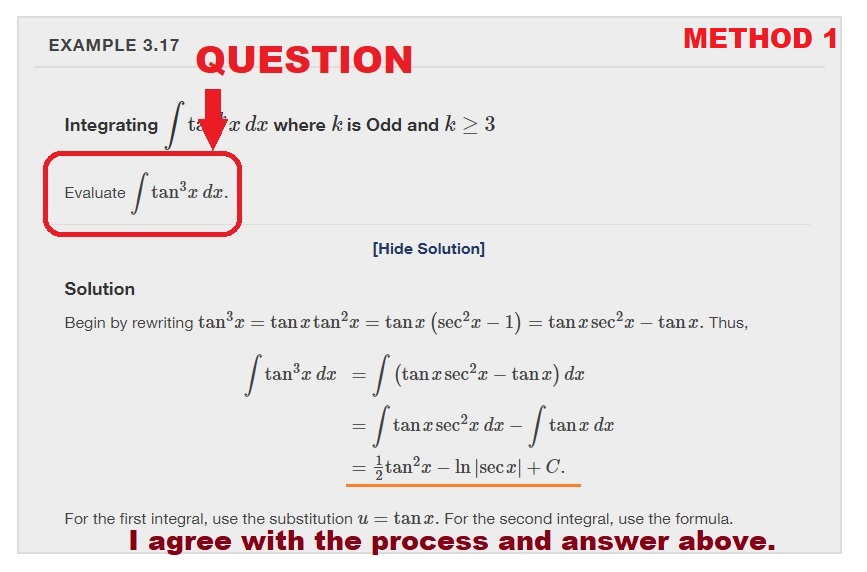
Good afternoon. If you see this post, could you please kindly review the two methods of calculations below to explain why their answers are different? Method 1 is from the Calculus 2 textbook, and Method 2 is from the computer calculator.

Moreover, I agree with both methods of calculations because there is nothing wrong with both approaches. Method 1 is to substitute u=tan(x), and Method 2 (Computer's Method) is to substitute u=sec(x).

Although their approaches are both correct, their answers are different! We all know that sec(x) is not equal to tan(x). Could you please kindly help me to check why their answers are different?

Please see the following two attached pictures. Thanks a lot! I am looking forward to your reply.

EXAMPLE 3.17 METHOD 1 QUESTION Integrating ta 'x da where k is Odd and k > 3 Evaluate / tan x dx. [Hide Solution] Solution Begin by rewriting tan'x = tantan'x = tana (sec2x - 1) = tanxsec'x - tanx. Thus, tan'x da = (tanasecar - tanx) dx = 1 tanxsec x dx - tanx dx = -tan x - In |secx] + C. For the first integral, use the substitution u = tan. For the second integral, use the formula. I agree with the process and answer above.calculator's Hide steps METHOD 2 Problem: method tan'(z) dr Rewrite: = tan'(z) tan(z) dr Rewrite/simplify using trigonometric/hyperbolic identities: tan (r) = sec?() - 1 (see ' (z) - 1) tan(z) de du 1 Substitute u = sec(I) - -= sec(x) tan(I) (steps) -> di = du sec(I) tan(I) w du .. or choose an alternative: Substitute cos(I) I also agree Expand: = (u-2)du However, the with the Apply linearity: answer from method from - Juda - /= du calculator is the computer Now solving: different from calculator. udu the textbook Apply power rule: (Method 1). with n = 1: Now solving: But we know du that This is a standard integral: = In(u) tan(x) * sec(x) Plug in solved integrals: udu - - du = 2 - In(2) Undo substitution u = sec(I): sec'(I) 2 -In(sec(I)) The problem is solved. Apply the absolute value function to arguments of logarithm functions in order to extend the antiderivative's domain: Final Answer tan' (z) dr sec' (I) = 2 - In(|sec(x)[) + C