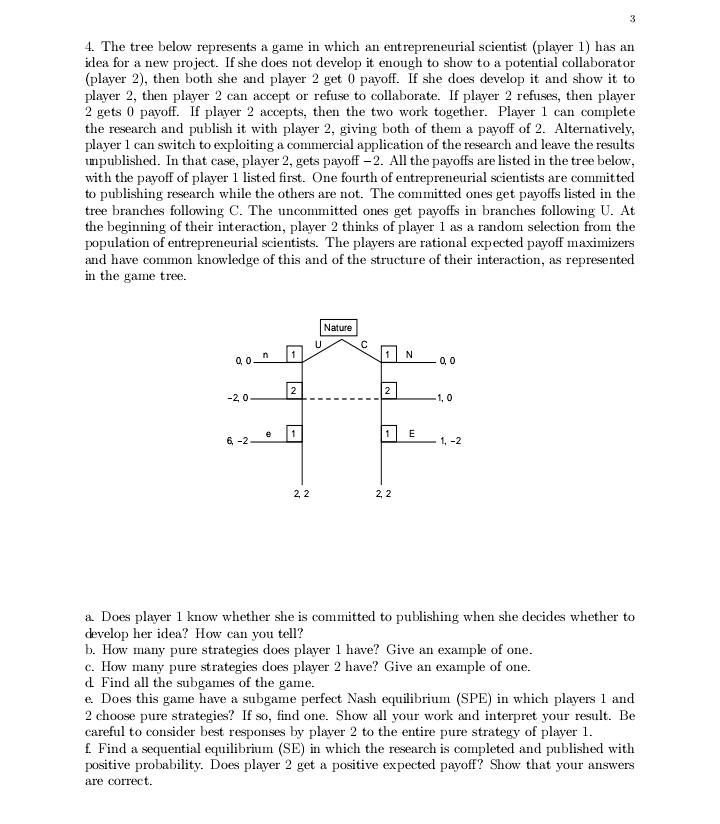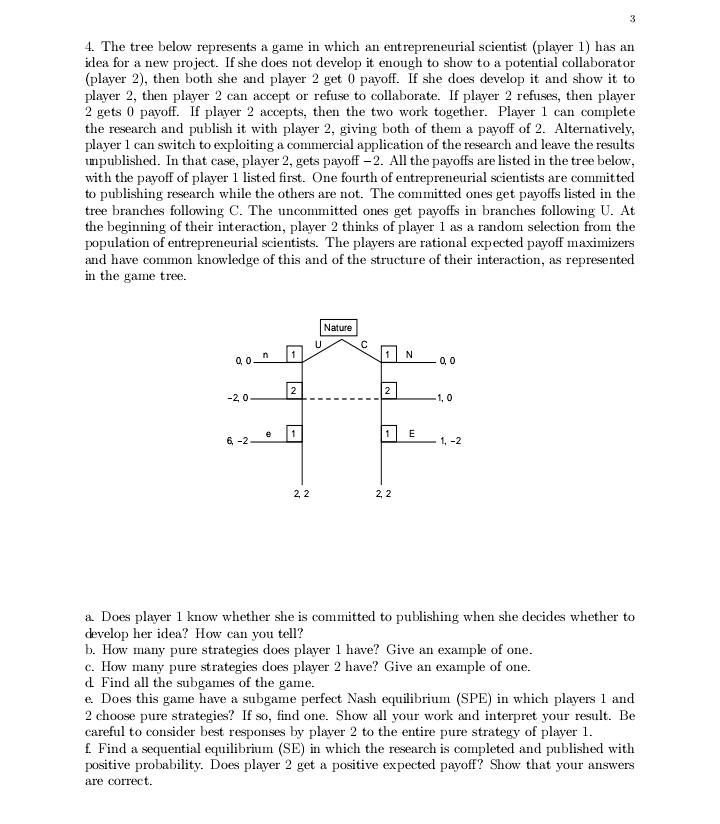
Help in solving this problem.k

6. (10) Consider the discrete time monetary-search model we saw in class. As in the baseline model, in the day time trade takes place in a decentralized market characterized by anonymity and bilateral meetings (call it the DM), and at night trade takes place in a Walrasian or centralized market (call it the CM). There are two types of agents, buyers and sellers, and the measure of both is normalized to the unit. The per period utility is u(q) + U(X) - H, for buyers, and -q + U(X) - H, for sellers; q is consumption of the DM good, X is consumption of the CM good (the numeraire), and H is hours worked in the CM. In the CM, one hour of work delivers one unit of the numeraire. The functions u, U satisfy standard properties. What is important here is that there exists X* > 0 such that U'(X*) = 1. Goods are non storable, but there exits a storable and recognizable object, called fiat money, that can serve as a means of payment. The supply of money, controlled by the monetary authority, follows the process Mitt = (1 + #)My, and new money is introduced via lump-sum transfers to buyers in the CM. So far, this is just a description of the model we saw in class. What is different here is that only a fraction of buyers turn out to have a desire to consume the DM good in the current period; let us refer to these buyers as C-types (for consumption) and to the remaining 1- a buyers as N-types (for no-consumption). The shock that determines each buyer's type in every period is did A buyer learns her type after all CM trade has concluded but before the DM opens. To make things interesting we will assume that between the CM and the DM there is a third market, where C-types and N-types can meet and trade "liquidity", i.e., money. Let us refer to this market as the loan market (LM). The LM is a bilateral market for loans, where N-types, who may carry some money that they do not need, meet C-types, who may need additional liquidity. A CRS matching function f (x, 1 - x) brings the two types together. Importantly, the LM is not anonymous, so that agents can make credible (and enforceable) promises. Hence, when an N-type and a C-type meet, they mutually benefit from a contract specifying that the N-type will give i units of money to the C-type right away, and the C-type will repay d (for debt) units of the numeraire good in the forthcoming CM. After the LM trades have concluded (for the agents who matched with someone), C-types proceed to the DM, where they use money to purchase goods from sellers. Assume that all C-type buyers match with a seller. Notice that I have not said anything about the splitting of the various surpluses (i.e., bargaining), because this information will not be necessary for what I am asking here. Let W( ) be the CM value function of a buyer, and V(.) the DM value function of a C-type buyer (since only these buyers visit the DM). Also, let ?'(.) be the LM value function of a type-i buyer, be {C, N). Your task in this question is to describe these value functions. I am not asking you to analyze them. I recommend that you draw a graph summarizing the timing of the model. (a) Describe the function W(.), and show that it is linear in all its arguments/state variables (what these arguments are, however, is for you to determine). (b) Let (q. p) be the quantity of good and the units of money exchanged in a typical DM meeting. Let (1, d) be the size of the loan (in dollars) and the promised repayment (in terms of the numeraire) specified in a typical LM meeting. What variables do the terms q, p, I, d depend on? Hint: Provide quick answers of the form "q is a function of the money holdings of the (C-type) buyer". (c) Describe the function V(.), where, again, determining the state variables is your task. (d) Describe the functions ?'(m), ie {C, N), for a buyer who enters the LM with m units of money. Hint: Recall that some buyers (of either type) will match in the LM and some will not, and the outcome of the matching process will critically affect a buyer's continuation value. Make sure that this is reflected in the expression you provide.4. The tree below represents a game in which an entrepreneurial scientist (player 1) has an idea for a new project. If she does not develop it enough to show to a potential collaborator (player 2), then both she and player 2 get 0 payoff. If she does develop it and show it to player 2, then player 2 can accept or refuse to collaborate. If player 2 refuses, then player 2 gets 0 payoff. If player 2 accepts, then the two work together. Player 1 can complete the research and publish it with player 2, giving both of them a payoff of 2. Alternatively, player I can switch to exploiting a commercial application of the research and leave the results unpublished. In that case, player 2, gets payoff -2. All the payoffs are listed in the tree below, with the payoff of player 1 listed first. One fourth of entrepreneurial scientists are committed to publishing research while the others are not. The committed ones get payoffs listed in the tree branches following C. The uncommitted ones get payoffs in branches following U. At the beginning of their interaction, player 2 thinks of player 1 as a random selection from the population of entrepreneurial scientists. The players are rational expected payoff maximizers and have common knowledge of this and of the structure of their interaction, as represented in the game tree. Nature L C n 1 - 0, 0 2 .2, 0 2 1, 0 6 -2. 1 1 | E - 1, -2 2 2 2, 2 a. Does player I know whether she is committed to publishing when she decides whether to develop her idea? How can you tell? b. How many pure strategies does player I have? Give an example of one. c. How many pure strategies does player 2 have? Give an example of one. d Find all the subgames of the game. e. Does this game have a subgame perfect Nash equilibrium (SPE) in which players 1 and 2 choose pure strategies? If so, find one. Show all your work and interpret your result. Be careful to consider best responses by player 2 to the entire pure strategy of player 1. f. Find a sequential equilibrium (SE) in which the research is completed and published with positive probability. Does player 2 get a positive expected payoff? Show that your answers are correct.UNIVERSITY AT ALBANY, STATE UNIVERSITY OF NEW YORK DEPARTMENT OF ECONOMICS Ph.D. Preliminary Examination in Microeconomics September 2, 2011 Instructions: Answer any three of the four problems below. Write your answer to each problem in a separate bluebook. Write the number of the problem on the cover of the bluebook. DO NOT WRITE YOUR NAME OR STUDENT ID NUMBER on the bluebooks. The exam lasts 4 hours. 1. Consider an L-good private ownership economy in which every consumer has a strictly positive endowment vector e, > 0 in R' and a utility function , : R4 - R with the following properties: (a) u, is strictly quasiconcave; and (b) the preferences determined by u, are locally nonsatiated. In addition, every consumer entirely owns its own firm and every firm has exactly one owner. The firms have the same convex production set Y e R', which has the following property: (c) yEY = ty EY for all te R+. A feasible allocation in this economy is one in which each consumer i gets a consumption vector r, E R4 and its firm chooses production vector y; ( Y such that ),(r - e, - y:) 5 0. a. Give a mathematical definition of strict quasiconcavity for a function u;- b. Use the notation above to define a competitive (Walrasian) equilibrium (without disposal) for this economy. c. Interpret property (c). 1. What can be said about the profit of firm i (owned by consumer i) in a competitive equilibrium of this economy? Be as specific as possible and show that your answer is correct. e. We call a competitive equilibrium in this economy self-sufficient if each consumer i gets a consumption vector 7, with 2 - e, C Y. Explain why the term "self-sufficient" applies to such an equilibrium. f. Is a self-sufficient competitive equilibrium allocation in this economy necessarily Pareto efficient? Suppose now that this economy has a self-sufficient competitive equilibrium in which each consumer i gets consumption vector Z,. Consider another competitive equilibrium with price vector p, consumption vector r, and production vector y; for each consumer i. g. Explain why pi, 0 who purchases commodities r and y at market prices p, > 0 and py > 0 respectively. However, in addition to incurring the direct cost associated with such expenditures, the consumer is also required to pay a transaction fee of 0.5% of the value of its purchases. (i) Write the budget equation for the consumer. (ii) How does this differ from the standard budget equation? b. In contrast, suppose the consumer was endowed with the bundle of goods w = (w,,wy) $ 0 rather than with income. In this case, the transaction cost is incurred as a result of changes from w and is paid in units of r, the numeraire. (That is, the transaction cost is incurred whether the consumer is a net buyer or a net seller of z.) Such costs are increasing in the size of the transaction. (i) Graphically depict the consumer's budget constraint which takes into consideration the transaction cost. Also, depict a typical consumer's equilibrium. (ii) Is it possible that a consumer would be a net buyer of r without the transaction cost, but would choose to be a net seller of r with the cost? (iii) Suppose the transaction cost is 0.5%% of the amount of a traded. Write an expression for the consumer's budget constraint and draw the consumer's budget set. c. Discuss the difference between the formulations in parts a and b. d Finally, consider an exchange economy with two consumers, 1 and 2, both of whom are is described in part b. Let w' = (wj, w;) denote the endowment of consumer z. (i) Assume that the consumers incur the cost of their own transactions. Set up the social planner's problem for determining the Pareto efficient allocations. (ii) Derive and interpret/explain the (necessary) first order conditions for efficiency at a solution to the planner's problem where consumer 1 is a net buyer of r