Help me with this

Grade 8

Everything must have a beginning . To understand the axiomatic development of geometry.

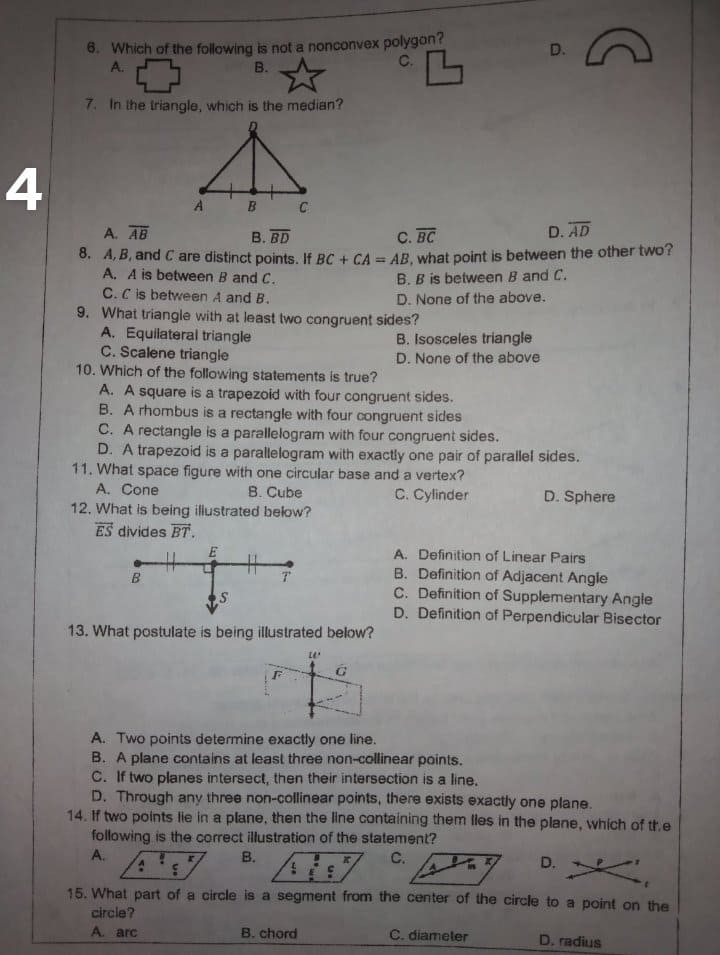
District: READINGS AND DISCUSSION Everything must have a beginning. To understand the axiomatic development of Geometry, bases must be established. Postulates, assumptions and theorems are considered as foundations of geometry, or any mathematical system for that matter. A mathematical mathematics system has following four parts, namely: undefined terms, defined terms, axioms and postulates, and theorems. Undefined Terms In a mathematical system, we come across many terms that cannot be precisely defined. In modern mathematics, we accept certain undefined terms. The choice of the undefined terms is completely arbitrary and generally to facilitate the development of the structure. The examples are points, lines, and planes. Point A location in space or a pinpoint suggests the idea of a point. A point has no dimension, no width, no length a no thickness. It is represented by a dot and is named by capital letters like A and B below. A. me Examples: 1. Tip of a pen 2. Corner of a paper 3. The intersection of two stringsB. Coplanar but not intersecting La 1 I F. d / are parallel lines, coplanar, and nonintersecting. C. Noncoplanar and nonintersecting Is and l are skew lines. They are noncoplanar and nonintersecting. Definition of Transversal A transversal is a line that Intersects two or more coplanar lines at two or more distinct points. Definitions of Angles Formed bylines and Transversal Alternate interior angles are two nonadjacent interior angles on opposite sides of the transversal. Alternate exterior angles are two nonadjacent exterior angles on opposite sides of the transversal. Corresponding angles are two nonadjacent angles, one interior, and one exterior on the same side of the transversal. Definition of Congruent Triangles Two triangles are congruent if their corresponding parts are congruent. Postulates Early Greeks considered postulates as general truths common to all studies and axioms as the truths relating to the special study at hand. A postulate is a statement that is accepted as true without proof 1. Two points determine exactly one line. 2. Three collinear points are contained in at least one plane and three noncollinear points are contained in exactly one plane. 3. If two distinct planes intersect, then their intersection is a line. 4. If two points of a line are in a plane, then the line is in the plane. 5. There is a one-to-one correspondence between the points of a line and the set of real numbers such that the distance between any two points of the line is the absolute value of the difference between the corresponding numbers. 6. Given two points P and S on a line, a coordinate system can be chosen 17 such a way that the coordinate of P is 0 and the coordinate of S is greater than 07. To every angle. there corresponds a unique real number r where 0