Hey there, answer all questions using strategies taught in grade 12 calculus. Thanks! (Full solutions too)

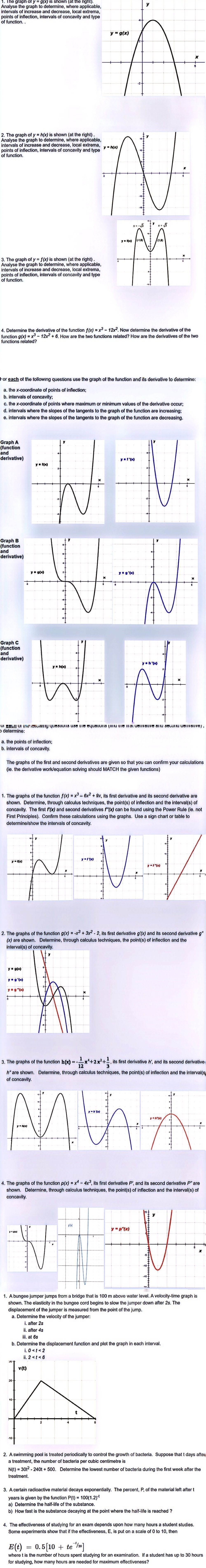
1. 1 ne grapn OT y = g(x) s (at tne ngnt) Analyse the graph to determine, where applicable, intervals of increase and decrease, local extrema, points of inflection, intervals of concavity and type of function.. Y g(x) 2. The graph of y = h(x) is shown (at the right) . Analyse the graph to determine, where applicable, intervals of increase and decrease, local extrema, points of inflection, intervals of concavity and type of function. au 3. The graph of y = f(x) is shown (at the right) . Analyse the graph to determine, where applicable, intervals of increase and decrease, local extrema, points of inflection, intervals of concavity and type of function. 4. Determine the derivative of the function f(x) = x3 12x2. Now determine the derivative of the function g(x) = x3 12x2 + 6. How are the two functions related? How are the derivatives of the two functions related? For each ot the following quesuons use the graph ot the function and its denvative to determine: a. the x-coordinate of points of inflection; b. intervals of concavity; c. the x-coordinate of points where maximum or minimum values of the derivative occur; d. intervals where the slopes of the tangents to the graph of the function are increasing; e. intervals where the slopes of the tangents to the graph of the function are decreasing. Graph A (function and derivative) Graph B (function and derivative) Graph C (function and derivative) ) determine: a. the points of inflection; b. intervals of concavity. The graphs of the first and second derivatives are given so that you can confirm your calculations (ie. the derivative worklequation solving should MATCH the given functions) 1. The graphs of the function f(x) = 6x2 + 9x, its first derivative and its second derivative are shown. Determine, through calculus techniques, the point(s) of inflection and the interval(s) of concavity. The first f'(x) and second derivatives can be found using the Power Rule (ie. not First Principles). Confirm these calculations using the graphs. Use a sign chart or table to determine/show the intervals of concavity. 2. The graphs of the function g(x) = -13 + 3x2 - 2, its first derivative g'(x) and its second derivative g" (x) are shown. Determine, through calculus techniques, the point(s) of inflection and the interval(s) of concavity. y gtx) y-t9 1 3. The graphs of the function h(x) = -x4+2 , its first derivative h', and its second derivative, 12 3 h" are shown. Determine, through calculus techniques, the point(s) of inflection and the interval(s of concavity. 4. The graphs of the function p(x) = x4 - 4x3, its first derivative P', and its second derivative P" are shown. Determine, through calculus techniques, the point(s) of inflection and the interval(s) of concavity. play l. A bungee jumper jumps from a bridge that is 100 m above water level. A velocity-time graph is shown. The elasticity in the bungee cord begins to slow the jumper down after 2s. The displacement of the jumper is measured from the point of the jump. a. Determine the velocity of the jumper: i. after 2s ii. after 4s iii. at 6s b. Determine the displacement function and plot the graph in each interval. 20 2 t 2. A swimming pool is treated periodically to control the growth of bacteria. Suppose that t days afteb a treatment, the number of bacteria per cubic centimetre is N(t) = 30t2 - 240t + 500. Determine the lowest number of bacteria during the first week after the treatment. 3. A certain radioactive material decays exponentially. The percent, P, of the material left after t years is given by the function P(t) = a) Determine the half-life of the substance. b) How fast is the substance decaying at the point where the half-life is reached ? 4. The effectiveness of studying for an exam depends upon how many hours a student studies. Some experiments show that if the effectiveness, E, is put on a scale of O to 10, then E(t) O. 5[10 + te-t/20] where t is the number of hours spent studying for an examination. If a student has up to 30 hours for studying, how many hours are needed for maximum effectiveness?