HHelp. me to solve the following attached questions.

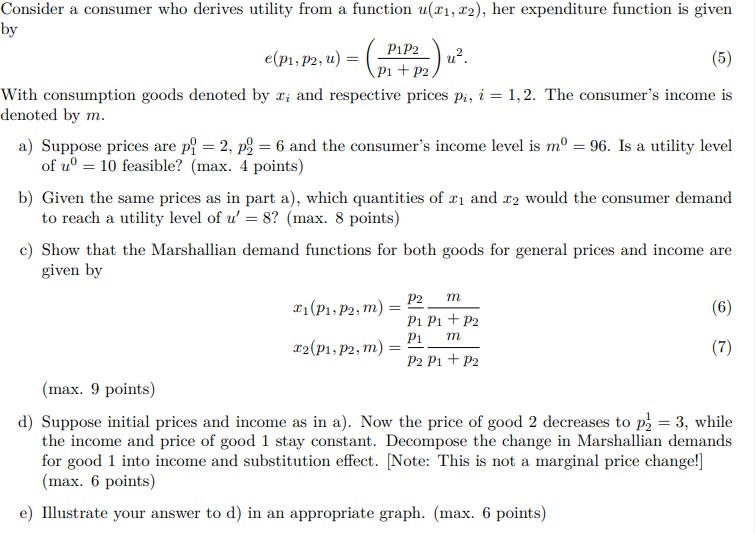
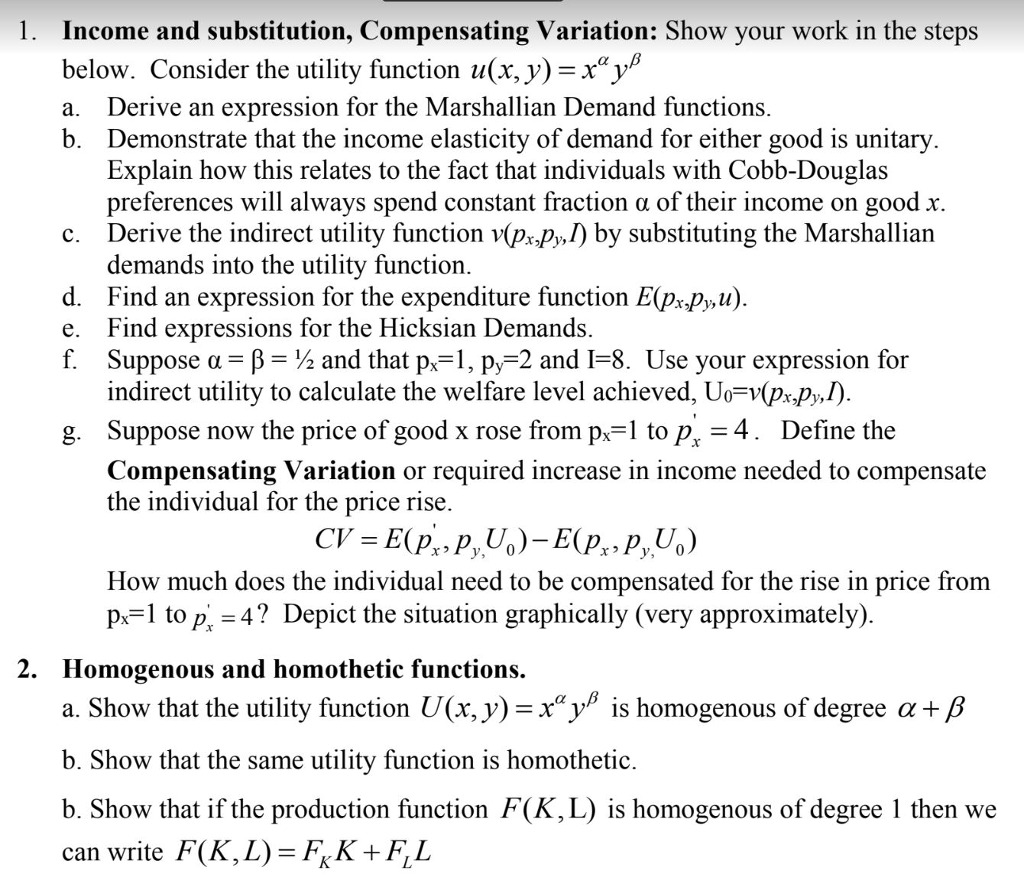
4 Labor market equilibrium with proportional taxation Consider a model in which a household derives utility from consumption c and leisure 1 according to the utility function u (c, l). The household has it hours to devote to either labor N or leisure i. The household owns xed capital stock K and rents it to the rm at rate r. The household owns shares in the rm and receives dividends in the form of rm prots 11'. The household takes prices and dividends as given. The rm maximizes prot and produces output Y according to the function F (K,N}, Where K is capital input and N is labor input. The rm takes prices as given. Consider a government that must nance a given level of spending G with a proportional tax on either labor income or output. Problem 4.1. Suppose the government imposes a proportional tax 1' on labor income so that after-tax labor income is (l T) wN, where w is the wage and N is the hours of labor worked. Write down the household's utility maximization problem. What is the household's rst order condition? Write down the rm's prot maximization problem. 1What is the rm's rst order condition? Show that the marginal rate of substitution M R8 is less than the marginal product of labor M PN . Use the Walrasian model of the labor market to argue, intuitively, about the effect of a proportional labor income tax on equilibrium wage and employment. Problem 4.2. Now suppose that the government imposes a proportional tax it on output so that the rm's after-tax revenue is (1 t)F (K,N). Again, write down the household's utility maximization problem. What is the household's rst order condition? Write down the rm's prot maximization problem. What is the rm's rst order condition. Show that M BS is less than M PN . Use the Walrasian model of the labor market to argue, intuitively, about the e'ect of a proportional labor income tax on equilibrium wage and employment. Problem 4.3. The government must now decide which taxation scheme to use. Under either the labor income tax or the output tax, tax revenue must equal government spending so that THIN = G and tY = G. Suppose that output is produced according to a constant returns to scale Cobb-Douglas production function so that Y = 2K \"N 1'\Consider a consumer who derives utility from a function mien, 1:2], her expenditure function is given by alpha-u) = (if???) 1.2. (a) With consumption goods denoted by .19.: and respective prices pi, i = 1, 2. The consumer's income is denoted by m. a] Suppose prices are p? = 2,. p3 = and the consumer's income level is m" = 96. Is a. utility level of n\" = If] feasible? (max. 4 points} h) Given the same prices as in part a}, which quantities of .191 and :35; would the consumer demand to reach a utility level of nF = 8? (max. 3 points} c) Show that the Marshallian demand functions for both goods for general prices and income are sirenbr pg m :r , ,m. = E 1(P1P2 } P1P1+ {l P1 are :r , ,m. = T 2(P1 P2 } 111+ f) (max. 9 points} d) Suppose initial prices and income as in a]. Now the price of good 2 decreases to p; = 3, while the income and price of good 1 stay constant. Deccmpcse the change in Marshallian demands for good 1 into income and substitution effect. [Note: This is not a marginal price changel] (max. '5 points} e) Illustrate your answer to d} in an appropriate graph. (max. [3 points} 1. Income and substitution, Compensating Variation: Show your work in the steps below. Consider the utility function u(x, y) = x y a. Derive an expression for the Marshallian Demand functions. b. Demonstrate that the income elasticity of demand for either good is unitary. Explain how this relates to the fact that individuals with Cobb-Douglas preferences will always spend constant fraction a of their income on good x. c. Derive the indirect utility function v(px,py,/) by substituting the Marshallian demands into the utility function. d. Find an expression for the expenditure function E(px,P,, u). e. Find expressions for the Hicksian Demands. f. Suppose a = = 1/2 and that px=1, py=2 and I=8. Use your expression for indirect utility to calculate the welfare level achieved, Uo=v(px,py,D). g. Suppose now the price of good x rose from px=1 to p. =4. Define the Compensating Variation or required increase in income needed to compensate the individual for the price rise. CV = E(Px, P,,U.) - E(Px, P,,U.) How much does the individual need to be compensated for the rise in price from Px=1 to p, = 4? Depict the situation graphically (very approximately). 2. Homogenous and homothetic functions. a. Show that the utility function U(x, y) = xay is homogenous of degree a + B b. Show that the same utility function is homothetic. b. Show that if the production function F(K, L) is homogenous of degree 1 then we can write F(K, L) = FKK + F,LMicroeconomic Theory - Problem Set. 3 16th November 2020 The solutions to these exercises will be made available on Monday November 23rd. You can submit your own solutions by 23:59 (CET) on Sunday November 22nd. 1, Let u : R2 - R where: with a, Be [0, 1). Assume pi py > 0 and that the consumer's income is y. Recall that, in Problem Set. 2 we derived the following Marshallian demand functions: (pipzy) = (a + ? ) PI By (a 1 9)P2 (a) Compute the indirect utility function v(P1, P2; #). (b) Show that the indirect utility function is homogeneous of degree 0 in (p, y). (c) Use Roy's identity to derive the Marshallian demand function for 21 using the indirect utility function. 2. A consumer has preferences over the single good, a and "all other goods", m represented by the utility function, u(2, m) = In(@) + m. Let the price of a be p, the price of me be 1 and let income be y. (a) Derive the Marshallian demands for a and m. (b) Derive the indirect utility function, v(p, y). (e) Use the Slutsky equation to decompose the effect of an own-price change on the demand for & into an income and substitution effect. Interpret you result briefly