Hi I am confuse on how to get to the result can you show work on how to get to the answer please. I need help with understanding it.

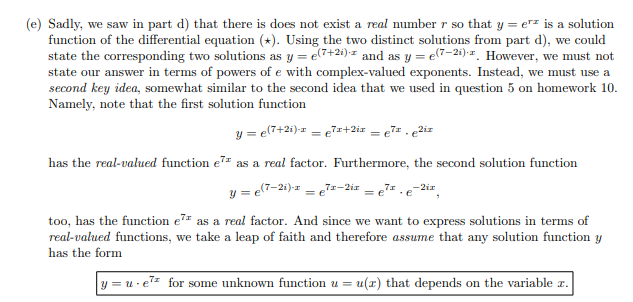
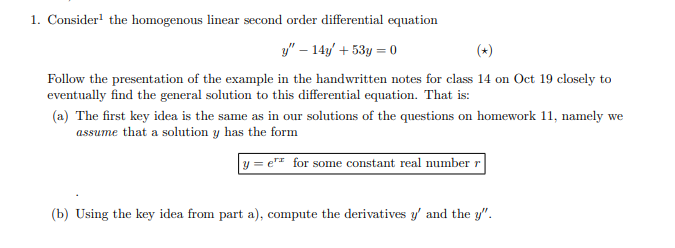
2. Use the same method as in question 1 to nd the general solution for the following differential equation: y\"+y'+25y= in Tb answer this question1 follow the exact same method as in question 1: that is' follow each step as in question la}l}. You must state your assumptions and your conclusions at each step clearly. Feel free to be hriefin your answers, but please do not skip any of the important steps? [c] Substitute the expressions for guy" and 3;" that you obtained in parts a] and b} into the dienential equation {1:}: this leads to an equation that must necessarily hold for the real number 1'. Dividing this equation by the expression e" 1which can never be equal to zero, 1will then lead to a. quadratic equation that must necessarily hold for the constant number 1'. Find this quadratic equation for the number 1'. Remark. This quadratic equation for the variable 1' is called the characteristic equation of the diHeDential equation it} (d) Solve the characteristic equation from part c) in order to find the two complex solutions for r. Hint. Use either the completing the square method, or the quadratic formula.(e) Sadly, we saw in part d) that there is does not exist a real number r so that y = ers is a solution function of the differential equation (*). Using the two distinct solutions from part d), we could state the corresponding two solutions as y = e(7+2:) and as y = e(7-21).3. However, we must not state our answer in terms of powers of e with complex-valued exponents. Instead, we must use a second key idea, somewhat similar to the second idea that we used in question 5 on homework 10. Namely, note that the first solution function y = e(7+20)-1 - 7x+2ix _ 7 . 21x has the real-valued function e' as a real factor. Furthermore, the second solution function y = (7-21)-1 = 0' 7x-211 - TI . E -2ir too, has the function ed as a real factor. And since we want to express solutions in terms of real-valued functions, we take a leap of faith and therefore assume that any solution function y has the form 1=u- elf for some unknown function a = a(x) that depends on the variable c.(f) Use the product rules and the assumption from part e) to carefully compute the derivatives y' and the y". In doing so, make sure to factor the expression e" in each expression for the derivatives.(g) Substitute the expressions for y, y' and y" that you obtained in parts e) and f) into the differential equation (*); this will lead to a second order differential equation that must necessarily hold for the function u = u(x). Dividing this equation by the expression e' which can never be equal to zero, this will to lead to a homogenous second order differential equation for u. Find this differential equation for the function u.(h) Find the general solution to the differential equation" that you obtained in part g). Remark. Follow the technique' explained in the handwritten notes of Class 14 from Oct 21. You will see that the general solution will involve two arbitrary constants-let's agree to call these constants C' and D. (i) Using the assumption from part e) and your solution from h) state your solution function y to the differential equation (#). (j) Use two different suitable pairs of constants C' and D to find two different solutions /(r) and g(x) with the goal of showing that these two solution functions are not constant multiples of each other. That is, find: . one solution function /(x) corresponding to the pair C =0 and D = 1: . one solution function g(x) corresponding to the pair C = 1 and D = 0: (k) Show that the two solutions that you obtained in part j) are not constant multiples of each other. (1) Use your work in parts a)- k) to find the general solution to the differential equation (*). Express your answer using constants c and d.1. Consider1 the hemogenorus linear seoond order differential equation 3;" - 1419' + 53y = {*1 FDIIDW the presentation of the example in the handwritten notes for clam 14 on Get 19 closely.r to eventuallgj.r nd the general solution to this differential equation. That is: [a] The first key idea is the same as in our solutions of the questions on homework 11, namely.r we assume that a solution y has the form 3; = e\" for son1e constant real number I" [b] Using the he}:r idea from part a}, compute the derivatives y\" and the y\