Hi! Is it possible to explain in detail with step-by-step instructions in accordance with the software (Show), Thank you!
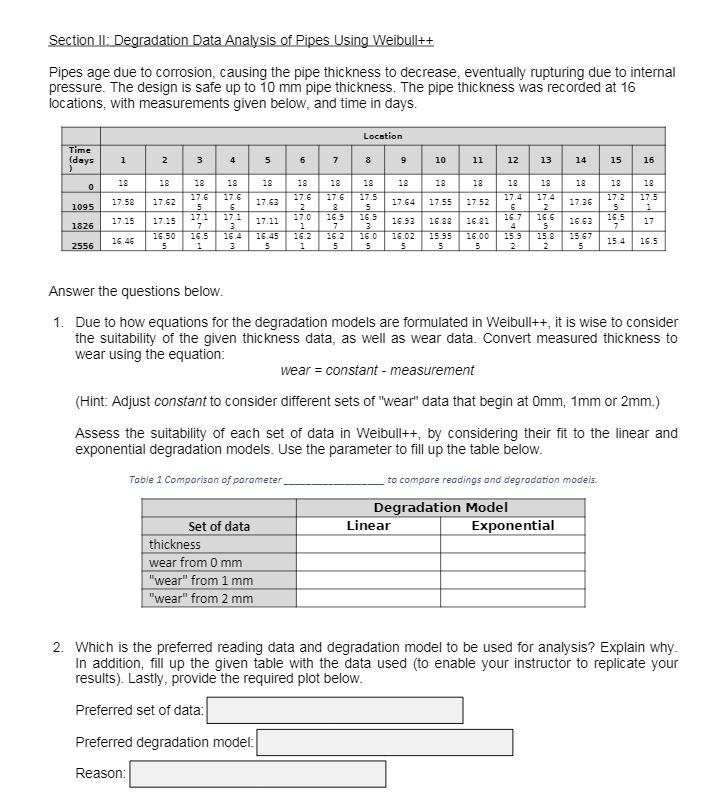
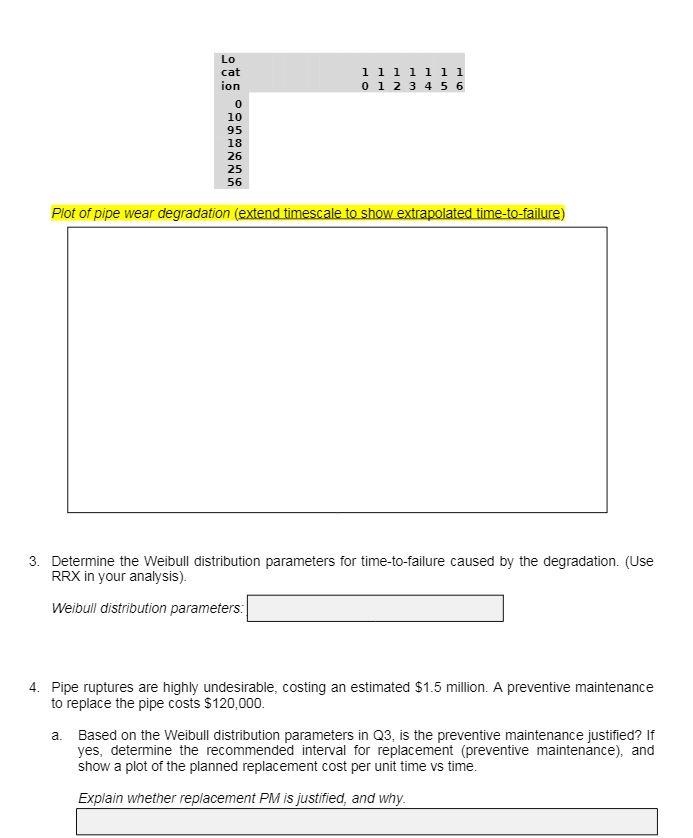

Section II: Degradation Data Analysis of Pipes Using Weibull++ Pipes age due to corrosion, causing the pipe thickness to decrease, eventually rupturing due to internal pressure. The design is safe up to 10 mm pipe thickness. The pipe thickness was recorded at 16 locations, with measurements given below, and time in days. Location Time (days ) 1 2 3 4 5 6 6. 7 7 8 10 11 12 13 14 15 16 18 18 18 18 18 18 18 18 19 28 18 18 18 18 18 18 0 17:58 17.62 17.63 17.64 17.55 17.52 17.36 17.5 1 1095 17.6 5 17.1 7 16.5 1 17.15 17.6 6 17.1 3 16.4 3 17.15 17.6 2 17.0 1 162 1 17.6 8 169 7 162 5 17.4 2 16.6 S 15.8 17.11 17.5 5 16.5 3 16.0 5 17.2 5 16.5 7 16.93 17.4 6 16.7 4 15.9 2 16.88 16.81 16.63 17 1826 16.46 16.50 5 16.45 5 16.02 5 15.95 5 16.00 5 15.67 5 15.4 16.5 2556 Answer the questions below. 1. Due to how equations for the degradation models are formulated in Weibull++, it is wise to consider the suitability of the given thickness data, as well as wear data. Convert measured thickness to wear using the equation: wear = constant - measurement (Hint: Adjust constant to consider different sets of "wear' data that begin at Omm, 1mm or 2mm.) Assess the suitability of each set of data in Weibull++, by considering their fit to the linear and exponential degradation models. Use the parameter to fill up the table below. Toble 1 Comparison of porometer to compore readings and degradation models. Degradation Model Linear Exponential Set of data thickness wear from 0 mm "wear" from 1 mm "wear" from 2 mm 2. Which is the preferred reading data and degradation model to be used for analysis? Explain why. In addition, fill up the given table with the data used to enable your instructor to replicate your results). Lastly, provide the required plot below. Preferred set of data: Preferred degradation model: Reason: Lo cat ion 1111111 0 1 2 3 4 5 6 10 95 18 26 25 56 Plot of pipe wear degradation (extend timescale to show extrapolated time-to-failure) 3. Determine the Weibull distribution parameters for time-to-failure caused by the degradation. (Use RRX in your analysis). Weibull distribution parameters: 4. Pipe ruptures are highly undesirable, costing an estimated $1.5 million. A preventive maintenance to replace the pipe costs $120,000 a. Based on the Weibull distribution parameters in Q3, is the preventive maintenance justified? If yes, determine the recommended interval for replacement (preventive maintenance), and show a plot of the planned replacement cost per unit time vs time. Explain whether replacement PM is justified, and why. Optimum interval for replacement (PM): Plot of the planned replacement cost per unit time vs time. b. Determine percentage of pipes that would have reached 10 mm by the recommended interval for replacement 5. Besides being known to be safe to 10 mm thickness, the pipes are also known to have a high chance of failure at 6 mm thickness. Determine how long it will take for a pipe to reach 6 mm thickness. (Hint: Re-run degradation analysis to 6 mm critical thickness and show the degradation plot). Time to reach 6 mm thickness: Plot of pipe wear degradation Section II: Degradation Data Analysis of Pipes Using Weibull++ Pipes age due to corrosion, causing the pipe thickness to decrease, eventually rupturing due to internal pressure. The design is safe up to 10 mm pipe thickness. The pipe thickness was recorded at 16 locations, with measurements given below, and time in days. Location Time (days ) 1 2 3 4 5 6 6. 7 7 8 10 11 12 13 14 15 16 18 18 18 18 18 18 18 18 19 28 18 18 18 18 18 18 0 17:58 17.62 17.63 17.64 17.55 17.52 17.36 17.5 1 1095 17.6 5 17.1 7 16.5 1 17.15 17.6 6 17.1 3 16.4 3 17.15 17.6 2 17.0 1 162 1 17.6 8 169 7 162 5 17.4 2 16.6 S 15.8 17.11 17.5 5 16.5 3 16.0 5 17.2 5 16.5 7 16.93 17.4 6 16.7 4 15.9 2 16.88 16.81 16.63 17 1826 16.46 16.50 5 16.45 5 16.02 5 15.95 5 16.00 5 15.67 5 15.4 16.5 2556 Answer the questions below. 1. Due to how equations for the degradation models are formulated in Weibull++, it is wise to consider the suitability of the given thickness data, as well as wear data. Convert measured thickness to wear using the equation: wear = constant - measurement (Hint: Adjust constant to consider different sets of "wear' data that begin at Omm, 1mm or 2mm.) Assess the suitability of each set of data in Weibull++, by considering their fit to the linear and exponential degradation models. Use the parameter to fill up the table below. Toble 1 Comparison of porometer to compore readings and degradation models. Degradation Model Linear Exponential Set of data thickness wear from 0 mm "wear" from 1 mm "wear" from 2 mm 2. Which is the preferred reading data and degradation model to be used for analysis? Explain why. In addition, fill up the given table with the data used to enable your instructor to replicate your results). Lastly, provide the required plot below. Preferred set of data: Preferred degradation model: Reason: Lo cat ion 1111111 0 1 2 3 4 5 6 10 95 18 26 25 56 Plot of pipe wear degradation (extend timescale to show extrapolated time-to-failure) 3. Determine the Weibull distribution parameters for time-to-failure caused by the degradation. (Use RRX in your analysis). Weibull distribution parameters: 4. Pipe ruptures are highly undesirable, costing an estimated $1.5 million. A preventive maintenance to replace the pipe costs $120,000 a. Based on the Weibull distribution parameters in Q3, is the preventive maintenance justified? If yes, determine the recommended interval for replacement (preventive maintenance), and show a plot of the planned replacement cost per unit time vs time. Explain whether replacement PM is justified, and why. Optimum interval for replacement (PM): Plot of the planned replacement cost per unit time vs time. b. Determine percentage of pipes that would have reached 10 mm by the recommended interval for replacement 5. Besides being known to be safe to 10 mm thickness, the pipes are also known to have a high chance of failure at 6 mm thickness. Determine how long it will take for a pipe to reach 6 mm thickness. (Hint: Re-run degradation analysis to 6 mm critical thickness and show the degradation plot). Time to reach 6 mm thickness: Plot of pipe wear degradation









