Answered step by step
Verified Expert Solution
Question
1 Approved Answer
Hi My issue is primarily with questions c) and d). So if these could be the most thorough I would really appreciate it. Thank you
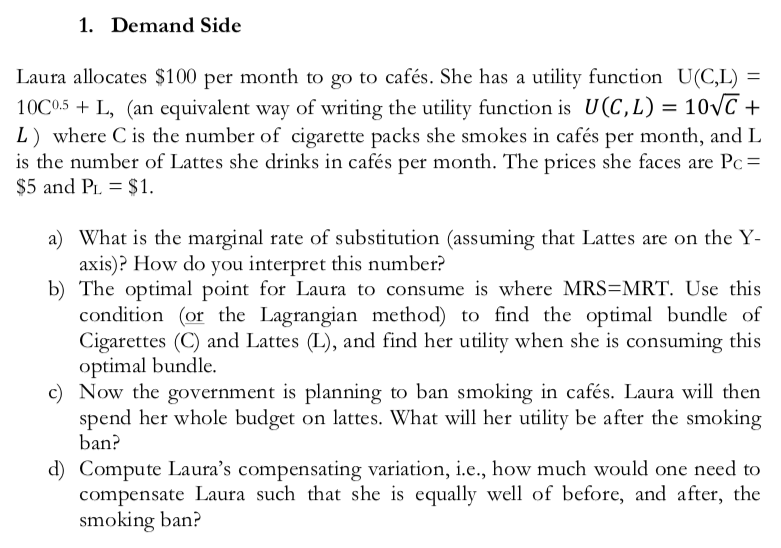
Hi
My issue is primarily with questions c) and d). So if these could be the most thorough I would really appreciate it.
Thank you in advance!
1. Demand Side = Laura allocates $100 per month to go to cafs. She has a utility function U(CL) = 10C0,5 + L, (an equivalent way of writing the utility function is U(C,L) = 10VC + L) where C is the number of cigarette packs she smokes in cafs per month, and L is the number of Lattes she drinks in cafs per month. The prices she faces are Pc = $5 and PL = $1. a) What is the marginal rate of substitution (assuming that Lattes are on the Y- axis)? How do you interpret this number? b) The optimal point for Laura to consume is where MRS=MRT. Use this condition (or the Lagrangian method) to find the optimal bundle of Cigarettes (C) and Lattes (L), and find her utility when she is consuming this optimal bundle. c) Now the government is planning to ban smoking in cafs. Laura will then spend her whole budget on lattes. What will her utility be after the smoking ban? d) Compute Laura's compensating variation, i.e., how much would one need to compensate Laura such that she is equally well of before, and after, the smoking ban? 1. Demand Side = Laura allocates $100 per month to go to cafs. She has a utility function U(CL) = 10C0,5 + L, (an equivalent way of writing the utility function is U(C,L) = 10VC + L) where C is the number of cigarette packs she smokes in cafs per month, and L is the number of Lattes she drinks in cafs per month. The prices she faces are Pc = $5 and PL = $1. a) What is the marginal rate of substitution (assuming that Lattes are on the Y- axis)? How do you interpret this number? b) The optimal point for Laura to consume is where MRS=MRT. Use this condition (or the Lagrangian method) to find the optimal bundle of Cigarettes (C) and Lattes (L), and find her utility when she is consuming this optimal bundle. c) Now the government is planning to ban smoking in cafs. Laura will then spend her whole budget on lattes. What will her utility be after the smoking ban? d) Compute Laura's compensating variation, i.e., how much would one need to compensate Laura such that she is equally well of before, and after, the smoking banStep by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


