HI, please assist me completing the assignment below. Thank you! I am NOT violating the honor code. This is just a school assingment that I need help with. I did not see any other tutor responding this assignment here on Course Hero. CAN SOMEONE PLEASE HELP ME.

The success average of a hockey player is the number of "points scored" divided by the number of "shots on goal." Recently, a certain professional league player's shots on goal and corresponding points scored were recorded for 400 consecutive games. The consecutive games span more than one season. Since each game is different, the number of shots and points scored both vary. For this particular player, there were from 0 to 15 shots. Thus, one can sort the more than 400 games into 16 categories:

0 shots

1 shot

2 shots

.

.

.

14 shots

15 shots

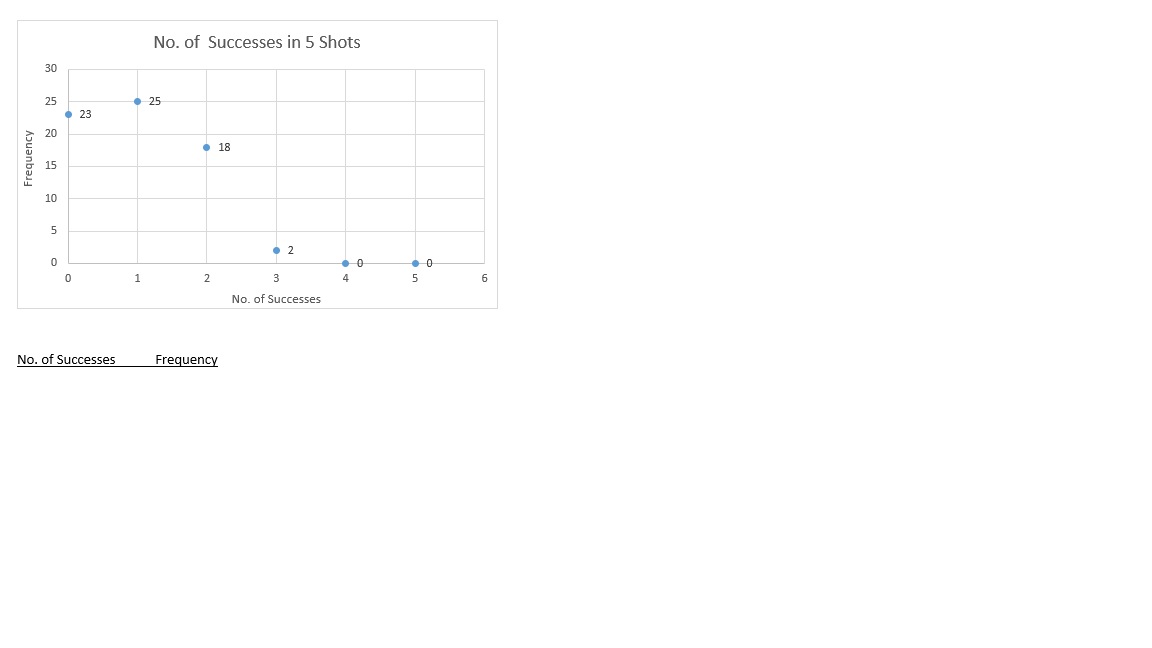
Consider the games where the player had exactly five shots on goal. A similar analysis can be done for each of the other shots category. Download the file titled Hockey. It contains a scatter plot of the Five Shots number of successes versus frequency. To compare the results to the Binomial Distribution, complete the following:

1. Explain why the five shots is a binomial experiment.
2. Using the Hockey scatter plot, construct a frequency distribution for the number of successes.
3. Compute the mean number of successes. The formula for the mean is.
4. Here, xirepresent no. of successes (0, 1, 2, 3, 4, 5) andfiis the corresponding frequency. Explain what the numerical result means.
5. From the frequency distribution, construct the corresponding probability distribution. Explain why it is a probability distribution. Then, use Excel to make a scatter plot of the probability distribution:
6. Select the two columns of the probability distribution. Click on INSERT, and then go to the Charts area and select Scatter. Then choose the first Scatter chart (the one without lines connecting).
7. Using the frequency distribution, what is the player's success average for five shots? In part 3, note that the numerator in the formula for the mean is the total number of successes. The total number of shots is the denominator of the formula for the mean multiplied by 5.
8. The Binomial Distribution is uniquely determined by n, the number of trials, and p, the probability of "success" on each trial. Using Excel, construct the Binomial Probability Distribution for five trials, n, and probability of success, p, as the success average in part 5. Here is an explanation of theBINOM.DIST function
9. (Links to an external site.)
11. Links to an external site.
12. in Excel
13. For example, In Excel
14. =BINOM.DIST(7,15,0.7,FALSE)
15. represents the probability of 7 successes out of 15 (n) trials. The 0.7 is the probability of success, p.
16. Using the formula for the mean of the binomial distribution, what is the mean number of successes in part 6 up above?
17. In Excel, make a scatter plot for the binomial distribution. The instructions for making one are in part 4 up above.
18. Use the results up above to compare the probability distribution of five shots and the Binomial Distribution. Compare the means in parts 4 and 6, too. If the probability distribution of five shots and the Binomial Distribution differ, explain why that is so.

No. of Successes in 5 Shots 30 25 25 23 Frequency 20 18 10 . 2 0 0 1 2 3 4 No. of Successes No. of Successes Frequency