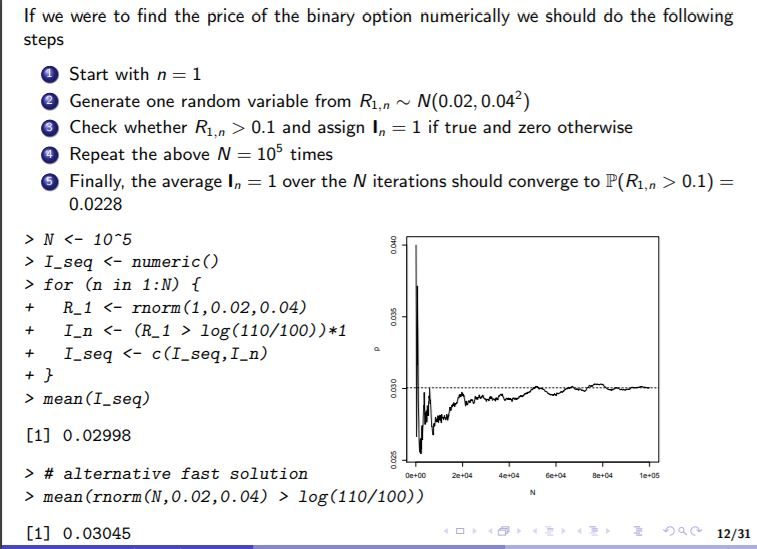
Hi - Please use R code to solve above. an example is above as well

3, Lucky Number : In this task, you need to demonstrate a way to numerically compute the irrational number . You will need to provide a plot demonstrating the approximation as a function of N. Make sure to add a line on the plot of the number T that your machine gives you, e.g. you can treat 3.14159265358979 as the "true" number Hint: while is considered an irrational number, it still has an important property when it comes to computing the circle area. Recall that the area of a given circle with radius r is given by Tr2. In order to compute , you need to numerically compute the area of a given circle. To do so, consider a circle of radius r being inscribed inside a square with side of length 2r. In order to compute the circle area, you need to generate random numbers for (x, y) coordinates Vx, y 0,, 2r. The proportion of these numbers that fall inside the circle will be proportional to the area of the circle. To determine whether a point falls inside the circle, recall that the circle equation is given by 3, Lucky Number : In this task, you need to demonstrate a way to numerically compute the irrational number . You will need to provide a plot demonstrating the approximation as a function of N. Make sure to add a line on the plot of the number T that your machine gives you, e.g. you can treat 3.14159265358979 as the "true" number Hint: while is considered an irrational number, it still has an important property when it comes to computing the circle area. Recall that the area of a given circle with radius r is given by Tr2. In order to compute , you need to numerically compute the area of a given circle. To do so, consider a circle of radius r being inscribed inside a square with side of length 2r. In order to compute the circle area, you need to generate random numbers for (x, y) coordinates Vx, y 0,, 2r. The proportion of these numbers that fall inside the circle will be proportional to the area of the circle. To determine whether a point falls inside the circle, recall that the circle equation is given by