How can I type up a program for this in MatLab?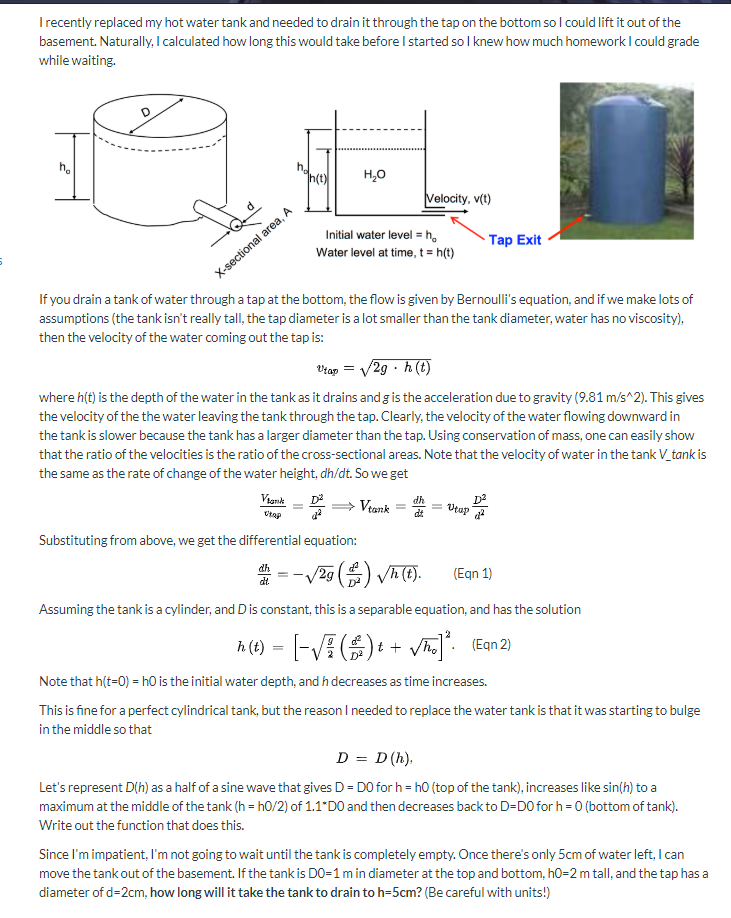

I recently replaced my hot water tank and needed to drain it through the tap on the bottom so I could lift it out of the basement. Naturally, I calculated how long this would take before I started so I knew how much homework I could grade while waiting "h(t) H,0 Velocity, v(t) Initial water level = h. Water level at time, t = h(t) Tap Exit X-sectional area, A If you drain a tank of water through a tap at the bottom, the flow is given by Bernoulli's equation, and if we make lots of assumptions (the tank isn't really tall, the tap diameter is a lot smaller than the tank diameter, water has no viscosity), then the velocity of the water coming out the tap is: Vtap = 29 - h (t) where h(t) is the depth of the water in the tank as it drains and g is the acceleration due to gravity (9.81 m/s^2). This gives the velocity of the the water leaving the tank through the tap. Clearly, the velocity of the water flowing downward in the tank is slower because the tank has a larger diameter than the tap. Using conservation of mass, one can easily show that the ratio of the velocities is the ratio of the cross-sectional areas. Note that the velocity of water in the tank V_tank is the same as the rate of change of the water height, dh/dt. So we get Substituting from above, we get the differential equation: le=-29 )h(t). (Egn 1) Assuming the tank is a cylinder, and Dis constant, this is a separable equation, and has the solution h(t) = (-VI )t + vhol (Egn 2) Note that h(t=0) = h0 is the initial water depth, and h decreases as time increases. This is fine for a perfect cylindrical tank, but the reason I needed to replace the water tank is that it was starting to bulge in the middle so that D = D(h), Let's represent D(h) as a half of a sine wave that gives D = DO for h = h0 (top of the tank), increases like sin(h) to a maximum at the middle of the tank (h=h0/2) of 1.1*DO and then decreases back to D=DO for h=0 (bottom of tank). Write out the function that does this. Since I'm impatient, I'm not going to wait until the tank is completely empty. Once there's only 5cm of water left, I can move the tank out of the basement. If the tank is DO=1 m in diameter at the top and bottom, h0=2 m tall, and the tap has a diameter of d=2cm, how long will it take the tank to drain to h=5cm? (Be careful with units!)