How do we we solve eq.(4) to be like eq.(5)

plz do it step by step

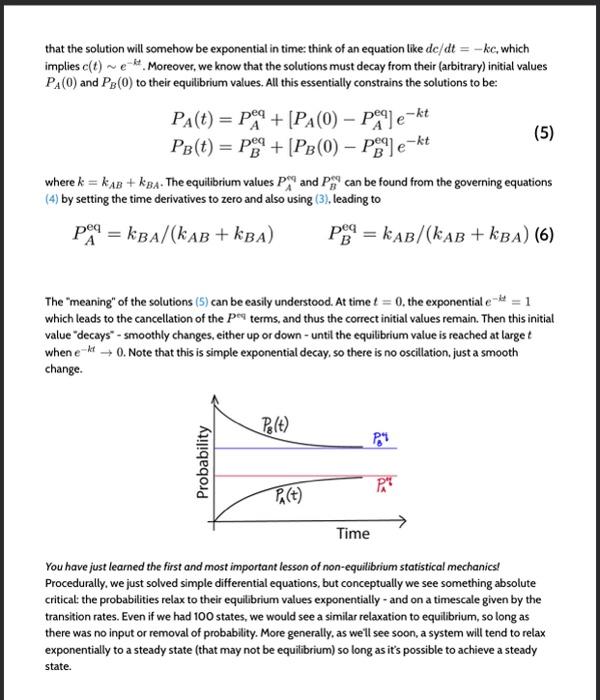
http://www.physicallensonthecell.org/discrete-state-kinetics-and-markov-models

where [X]=NX/V is the number of molecules in state X divided by the volume-i.e, the concentration of molecules in state X. These equations govern the behavior of the two concentrations as functions of time. For mathematical convenience, we will consider an equivalent set of equations written in terms of the state probabilities PX(t) which make it easier to indicate the dependence on time t explicitly. PX is simply the fraction NX/N of molecules in state X, where N=NA+NB is the total number of molecules, which in turn implies we are assuming every molecule is either in state A or B so that PA(t)+PB(t)=1 After multiplication by V/N, the governing differential equations (2) then become dtdPA=PA(t)kAB+PB(t)kBAdtdPB=PA(t)kABPB(t)kBA where the rate constants have the same meaning as above. Before we give the exact solution to the equations (4), there are a couple of things to observe about them. First, because they are equivalent to mass action equations, they implicitly assume the rate constants are independent of state concentrations. This is not necessarily true, however. We can imagine that in state A, for instance, the molecules tend to aggregate which might inhibit (slow down) the transition to state B at large [A] values. In our mathematical treatment, which is fully standard, we will ignore this possibility. Second, there is something funny about the set of equations (4) - which occurs for many such system of mass-action equations. Try summing the two equations, and you will find the right-hand sides sum to zero. This tells us that the sum of the left-hand sides must also vanish: d(PA+PB)/dt=0. Is this true? Yes!l In fact, in Eq. (3) we already saw that the sum of the concentrations was unchanging in time, which immediately implies that its time derivative vanishes. So there's no mistake here, but this strange property of the sum of the two differential equations is actually telling us that one of the equations is unnecessary. The two equations are not independent because of the constraint on the sum of PA and PB. There are indeed two unknown functions of time, PA(t) and PB(t), but the two equations determining the time dependence of the PX functions in fact are (3) and either one of equations (4). How do we get the solution? First notice that the system of equations (4) has first derivatives on the lefthand side and is linear in the probabilities on the right-hand side. Given that, we know (from experience) that the solution will somehow be exponential in time: think of an equation like dc/dt=kc, which implies c(t)ekt. Moreover, we know that the solutions must decay from their (arbitrary) initial values PA(0) and PB(0) to their equilibrium values. All this essentially constrains the solutions to be: PA(t)=PAeq+[PA(0)PAeq]ektPB(t)=PBeq+[PB(0)PBeq]ekt (4) by setting the time derivatives to zero and also using (3), leading to PAeq=kBA/(kAB+kBA)PBeq=kAB/(kAB+kBA)(6) The "meaning" of the solutions (5) can be easily understood. At time t=0, the exponential eit=1 which leads to the cancellation of the Peq terms, and thus the correct initial values remain. Then this initial value "decays" - smoothly changes, either up or down - until the equilibrium value is reached at large t when ekt0. Note that this is simple exponential decay, so there is no oscillation, just a smooth change. You have just leamed the first and most important lesson of non-equilibrium statistical mechanics! Procedurally, we just solved simple differential equations, but conceptually we see something absolute critical: the probabilities relax to their equilibrium values exponentially - and on a timescale given by the transition rates. Even if we had 100 states, we would see a similar relaxation to equilibrium, so long as there was no input or removal of probability. More generally, as well see soon, a system will tend to relax exponentially to a steady state (that may not be equilibrium) so long as it's possible to achieve a steady state