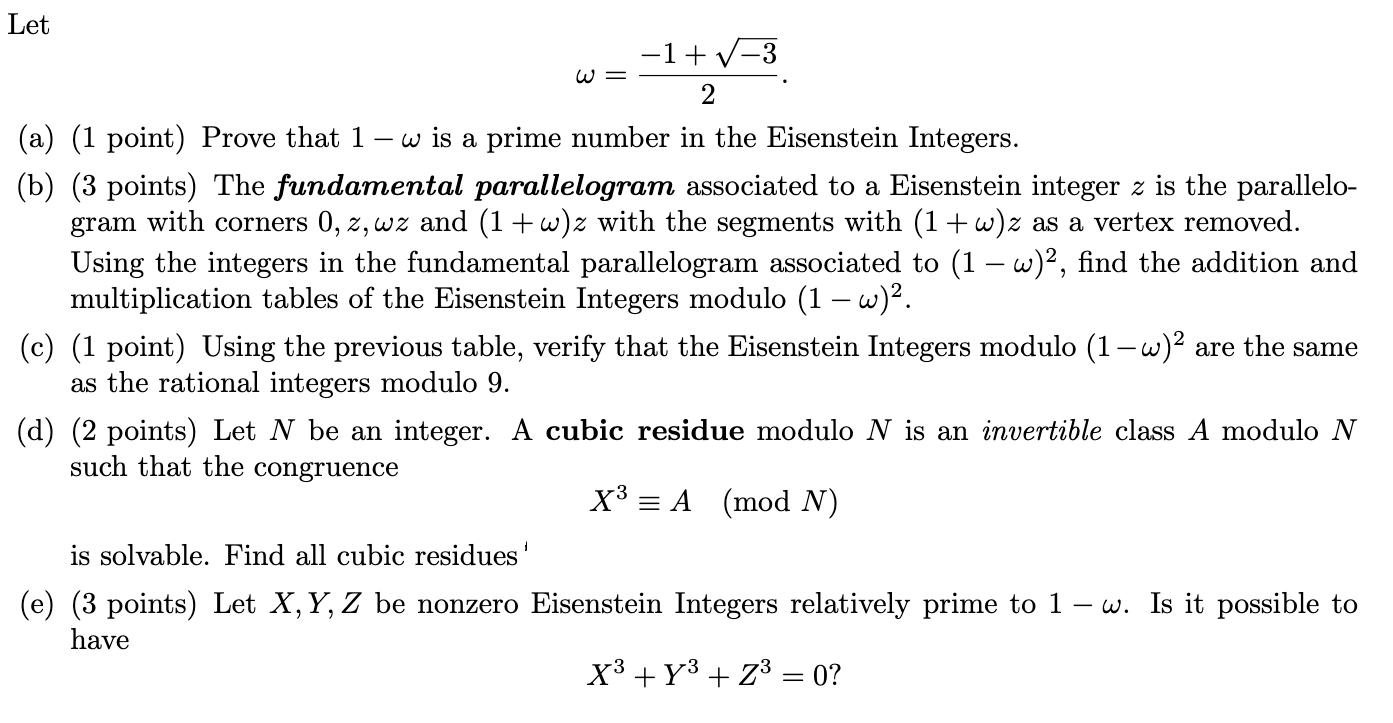
Let W= -1+-3 2 (a) (1 point) Prove that 1 - w is a prime number in the Eisenstein Integers. (b) (3 points) The fundamental parallelogram associated to a Eisenstein integer z is the parallelo- gram with corners 0, z, wz and (1+w)z with the segments with (1+w) z as a vertex removed. Using the integers in the fundamental parallelogram associated to (1 w), find the addition and multiplication tables of the Eisenstein Integers modulo (1 w). (c) (1 point) Using the previous table, verify that the Eisenstein Integers modulo (1-w) are the same as the rational integers modulo 9. (d) (2 points) Let N be an integer. A cubic residue modulo N is an invertible class A modulo N such that the congruence X = A (mod N) is solvable. Find all cubic residues' (e) (3 points) Let X, Y, Z be nonzero Eisenstein Integers relatively prime to 1 - w. Is it possible to have X + Y + Z = 0?