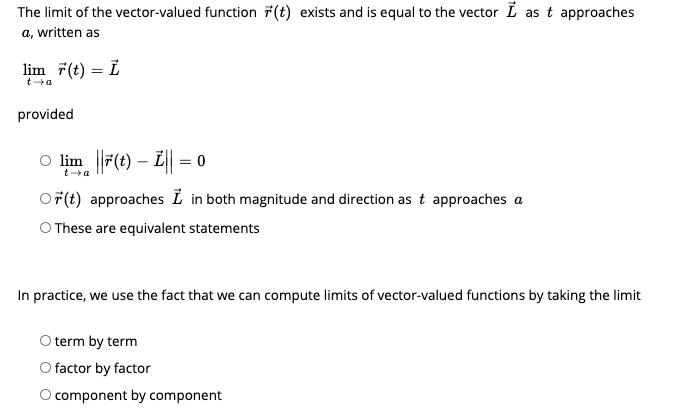
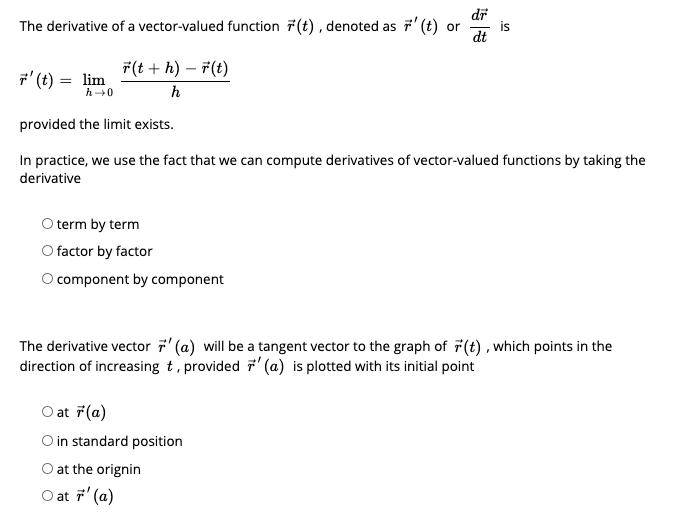
The limit of the vector-valued function 7(t) exists and is equal to the vector L as t approaches a, written as lim 7(t) = L ta provided O lim 7(t) L| = 0 ta O7(t) approaches L in both magnitude and direction as t approaches a O These are equivalent statements In practice, we use the fact that we can compute limits of vector-valued functions by taking the limit O term by term Ofactor by factor O component by component dr The derivative of a vector-valued function 7(t), denoted as 7'(t) or dt 7(t + h)- F(t) 7'(t) = lim h+0 h provided the limit exists. In practice, we use the fact that we can compute derivatives of vector-valued functions by taking the derivative O term by term factor by factor O component by component The derivative vector 7' (a) will be a tangent vector to the graph of 7(t), which points in the direction of increasing t, provided '(a) is plotted with its initial point O at 7(a) O in standard position at the orignin O at 7'(a)