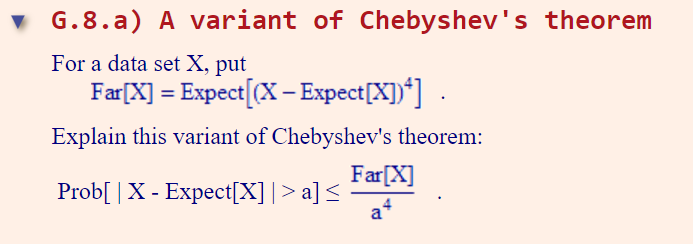
Question
I have been doing a unit in my probability theory and statistics course on Markov's inequality and Chebyshev's theorem. This below question is stumping me,
I have been doing a unit in my probability theory and statistics course on Markov's inequality and Chebyshev's theorem. This below question is stumping me, I'm not quite sure where to start. The only hint I have gotten is this: The key to explaining this is using Markov's inequality. Markov's inequality tells usk * Prob[Y > k]
If we carefully choose our k and Y we can solve the above problem. You setY =(X - Expect[X])^2 , but it may be easier to choose a Y such that Expect[Y] = Far[X].
Could I have some advice on how to tackle this problem? Thank you!
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


