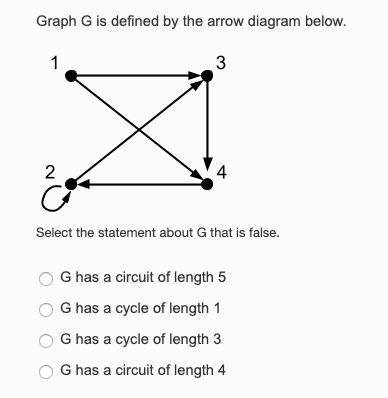
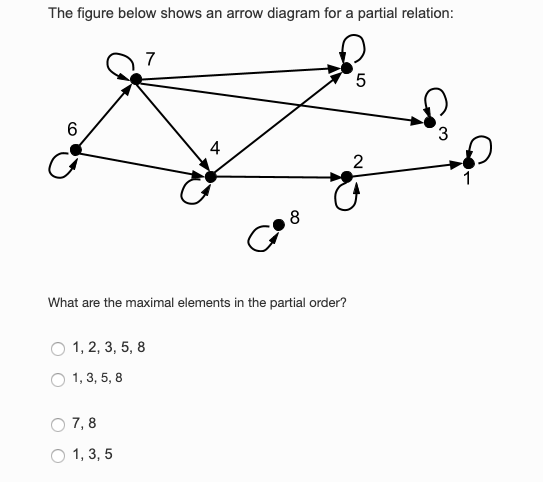
I just need answers to these question, work is not required

Select the correct rule to replace (?) in the proof segment below: 1. -pvq Hypothesis 2. -P Hypothesis 3. (?) Disjunctive syllogism Simplification Resolution O Hypothetical syllogismGraph G is defined by the arrow diagram below. 3 2 Select the statement about G that is false. O G has a circuit of length 5 G has a cycle of length 1 G has a cycle of length 3 O G has a circuit of length 4A donut store sells packages of 12 donuts. The store has made x donuts. How many complete packages does the store have for sale? O [12x1 O [x/12] O [x/121 O [ 12x]The domain of relation R is the set of all integers. is related to if |x-y|s1 . Which statement correctly characterizes the relation R? Q R is not an equivalence relation because R is not reexive. Q R is not an equivalence relation because R is not symmetric. Q R is not an equivalence relation because R is not transitive. Q R is an equivalence relation. Which rule is used in the argument below? Alice is a student in the class. Alice got an A on the test and did not study. Therefore, there is a student in the class who got an A on the test and did not study. O iversal instantiation Universal generalization O Existential generalization Existential instantiationDefine strings s = 101 and t = 10. Select the string that is equal to st. O 10 O 101 O 10110 101101The figure below shows an arrow diagram for a partial relation: 7 5 6 4 3 2 1 8 What are the maximal elements in the partial order? O 1, 2, 3, 5, 8 1, 3, 5, 8 0 7, 8 O 1, 3, 5Select the truth assignment that shows that the argument below is not valid: pv q OP =F q =T Op = F q = F OP =T q = F OP= T q =TA = {a, b, c, d} X = {1, 2, 3, 4) Select the definition for f that is a well-defined function. Of = {(a, 2), (b, 3), (c, 3), (d, 1)} Of = {(a, 2), (b, 3), (b, 3), (d, 1)} Of = {(a, 2), (b, 3), (d, 1)} Of = {(a, 2), (b, 3), (c, 3), (1, d)}4:10,1)3_+10,1)3 f (x) is obtained by removing the second bit from x and placing the bit at the end of the string. For example, f(101) = 110. Select the correct description of the function f. O One-to-one but not onto Neither one-to-one nor onto O One-to-one and onto O Onto but not one-to-one