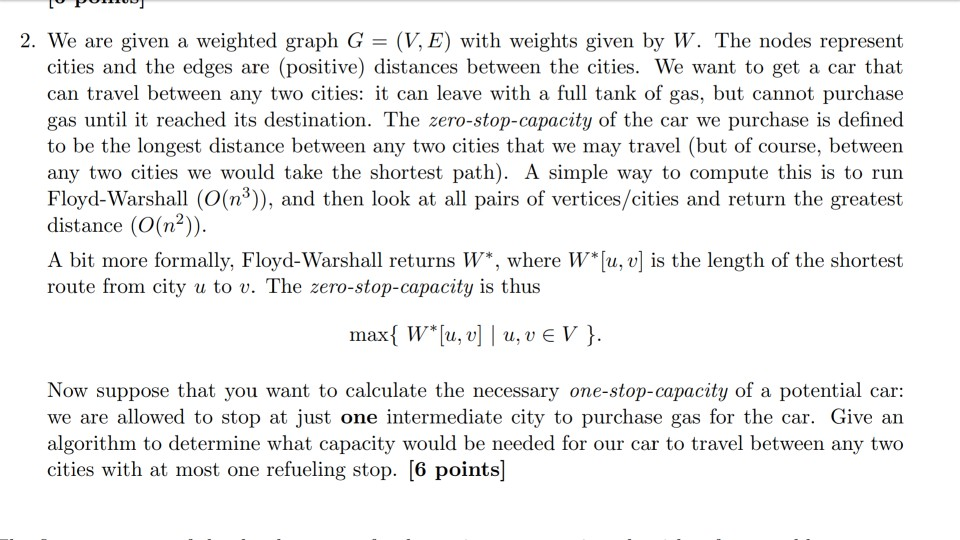
I just need q2 to be done. The question ends where it says (6points)

10 PomDJ 2. We are given a weighted graph G = (V, E) with weights given by W. The nodes represent cities and the edges are positive) distances between the cities. We want to get a car that can travel between any two cities: it can leave with a full tank of gas, but cannot purchase gas until it reached its destination. The zero-stop-capacity of the car we purchase is defined to be the longest distance between any two cities that we may travel (but of course, between any two cities we would take the shortest path). A simple way to compute this is to run Floyd-Warshall (O(n)), and then look at all pairs of vertices/cities and return the greatest distance (O(n?)). A bit more formally, Floyd-Warshall returns W*, where W* (u, v) is the length of the shortest route from city u to v. The zero-stop-capacity is thus max{ W* [u, v) | UV EV}. Now suppose that you want to calculate the necessary one-stop-capacity of a potential car: we are allowed to stop at just one intermediate city to purchase gas for the car. Give an algorithm to determine what capacity would be needed for our car to travel between any two cities with at most one refueling stop. [6 points] The first two steps of the development of a dynamic programming algorithm for a problem are step 1 describe the structure of the subproblem step 2 find a recurrence for the optimal value of the subproblem in terms of smaller subproblems Perform just these two steps for the two problems listed below. Do not write (pseudo) code - just the subproblem and recurrence structure. LO PO1100) 2. We are given a weighted graph G = (V, E) with weights given by W. The nodes represent cities and the edges are (positive) distances between the cities. We want to get a car that can travel between any two cities: it can leave with a full tank of gas, but cannot purchase gas until it reached its destination. The zero-stop-capacity of the car we purchase is defined to be the longest distance between any two cities that we may travel (but of course, between any two cities we would take the shortest path). A simple way to compute this is to run Floyd-Warshall (O(n)), and then look at all pairs of vertices cities and return the greatest distance (O(n?)). A bit more formally, Floyd-Warshall returns W*, where W*[u, v) is the length of the shortest route from city u to v. The zero-stop-capacity is thus max{ W*[u, v] | U, V EV}. Now suppose that you want to calculate the necessary one-stop-capacity of a potential car: we are allowed to stop at just one intermediate city to purchase gas for the car. Give an algorithm to determine what capacity would be needed for our car to travel between any two cities with at most one refueling stop. (6 points)