i just need to solve part 1 please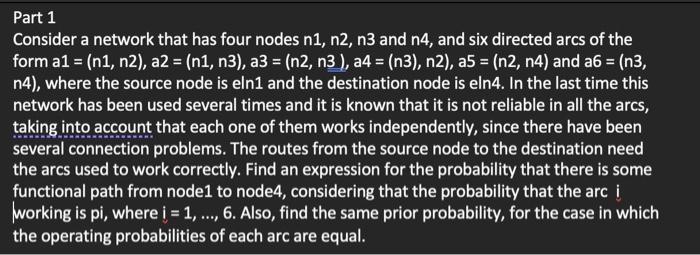

Part 1 Consider a network that has four nodes n1, n2, n3 and n4, and six directed arcs of the form a1 = (n1, n2), a2 = (n1, n3), a3 = (n2, n3 ), a4 = (n3), n2), a5 = (n2, n4) and a6 = (n3, n4), where the source node is eln1 and the destination node is eln4. In the last time this network has been used several times and it is known that it is not reliable in all the arcs, taking into account that each one of them works independently, since there have been several connection problems. The routes from the source node to the destination need the arcs used to work correctly. Find an expression for the probability that there is some functional path from node1 to node4, considering that the probability that the arc i working is pi, where i = 1, ..., 6. Also, find the same prior probability, for the case in which the operating probabilities of each arc are equal. Part 2 (a) Let X be a random variable, defined as X = Y Z, where Y and Z are independent random variables, taking values -1 or 1 with probability 0.5. Show that X is independent of YYZ, but not of Y + Z. (B) Let X be a continuous random variable with density function f (x). Obtain the expression for the expected value of X conditioned to Xs a and replace with the case where the variable X-Exp (). Part 3 You went to the supermarket and you already have all the products you want to buy, you go to the checkout and there are two people in front of you in line, one who is already paying and another who is waiting to be seen. Consider that the time for product scanning and payment (going to checkout) has an exponential distribution with an expected value of 10 minutes and that the person at the checkout has been there for 2 minutes. Find the probability that the person at the checkout will leave in less than 5 minutes and the probability that they will have to wait more than 15 minutes to leave. Part 1 Consider a network that has four nodes n1, n2, n3 and n4, and six directed arcs of the form a1 = (n1, n2), a2 = (n1, n3), a3 = (n2, n3 ), a4 = (n3), n2), a5 = (n2, n4) and a6 = (n3, n4), where the source node is eln1 and the destination node is eln4. In the last time this network has been used several times and it is known that it is not reliable in all the arcs, taking into account that each one of them works independently, since there have been several connection problems. The routes from the source node to the destination need the arcs used to work correctly. Find an expression for the probability that there is some functional path from node1 to node4, considering that the probability that the arc i working is pi, where i = 1, ..., 6. Also, find the same prior probability, for the case in which the operating probabilities of each arc are equal. Part 1 Consider a network that has four nodes n1, n2, n3 and n4, and six directed arcs of the form a1 = (n1, n2), a2 = (n1, n3), a3 = (n2, n3 ), a4 = (n3), n2), a5 = (n2, n4) and a6 = (n3, n4), where the source node is eln1 and the destination node is eln4. In the last time this network has been used several times and it is known that it is not reliable in all the arcs, taking into account that each one of them works independently, since there have been several connection problems. The routes from the source node to the destination need the arcs used to work correctly. Find an expression for the probability that there is some functional path from node1 to node4, considering that the probability that the arc i working is pi, where i = 1, ..., 6. Also, find the same prior probability, for the case in which the operating probabilities of each arc are equal. Part 2 (a) Let X be a random variable, defined as X = Y Z, where Y and Z are independent random variables, taking values -1 or 1 with probability 0.5. Show that X is independent of YYZ, but not of Y + Z. (B) Let X be a continuous random variable with density function f (x). Obtain the expression for the expected value of X conditioned to Xs a and replace with the case where the variable X-Exp (). Part 3 You went to the supermarket and you already have all the products you want to buy, you go to the checkout and there are two people in front of you in line, one who is already paying and another who is waiting to be seen. Consider that the time for product scanning and payment (going to checkout) has an exponential distribution with an expected value of 10 minutes and that the person at the checkout has been there for 2 minutes. Find the probability that the person at the checkout will leave in less than 5 minutes and the probability that they will have to wait more than 15 minutes to leave. Part 1 Consider a network that has four nodes n1, n2, n3 and n4, and six directed arcs of the form a1 = (n1, n2), a2 = (n1, n3), a3 = (n2, n3 ), a4 = (n3), n2), a5 = (n2, n4) and a6 = (n3, n4), where the source node is eln1 and the destination node is eln4. In the last time this network has been used several times and it is known that it is not reliable in all the arcs, taking into account that each one of them works independently, since there have been several connection problems. The routes from the source node to the destination need the arcs used to work correctly. Find an expression for the probability that there is some functional path from node1 to node4, considering that the probability that the arc i working is pi, where i = 1, ..., 6. Also, find the same prior probability, for the case in which the operating probabilities of each arc are equal