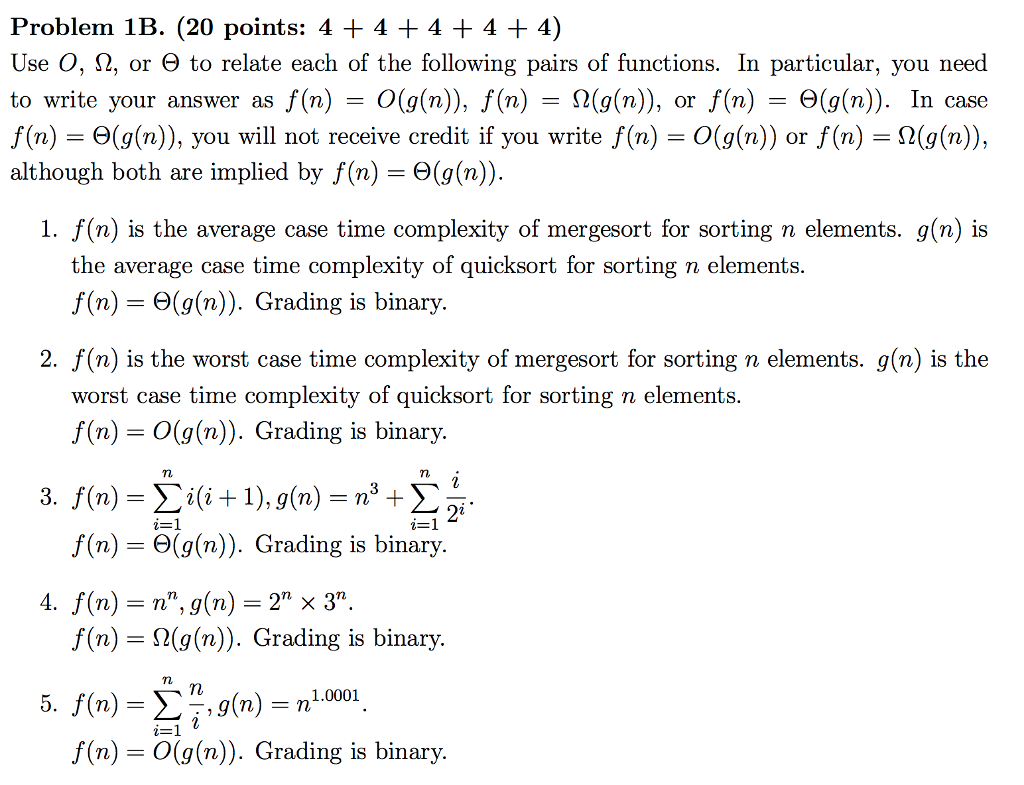
I need a detailed answer please, this is a review for my upcoming exam

Use O, Ohm, or Theta to relate each of the following pairs of functions. In particular, you need to write your answer as f(n) = 0(g(n)), f(n) = Ohm(g(n)), or f(n) = Theta(g(n)). In case f(n) = Ohm(g(n)), you will not receive credit if you write f(n) = O(g(n)) or f(n) = Ohm(g(n)), although both are implied by f(n) = Ohm(g(n)). f(n) is the average case time complexity of mergesort for sorting n elements. g(n) is the average case time complexity of quicksort for sorting n elements. f(n) = Theta(g(n)). Grading is binary. f(n) is the worst case time complexity of mergesort for sorting n elements. g(n) is the worst case time complexity of quicksort for sorting n elements. f(n) = Theta(g(n)). Grading is binary. f(n) is the worst case time complexity of mergesort for sorting n elements. g(n) is the worst case time complexity of quicksort for sorting n elements. f(n) = O(g(n)). Grading is binary. f(n) = sigma_i = 1^n i(i + 1), g(n) = n^3 + sigma_i = 1^n i/2^i. f(n) = Theta (g(nn). Grading is binary. f(n) = n^n g(n) = 2^n times 3^n. f(n) = Ohm (g(n)). grading is binary. f(n) = sigma_i = 1^n n/i, g(n) = n^1.001 f(n) = O(g(n)). Grading is binary