I need answers of these questions ASAP !! PLEASE. Note: this is complete question and i have attached references

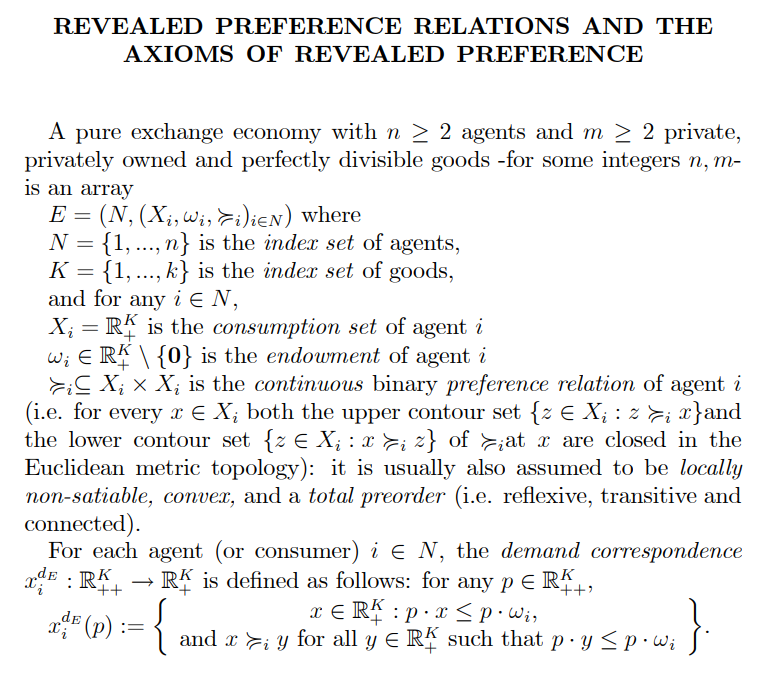
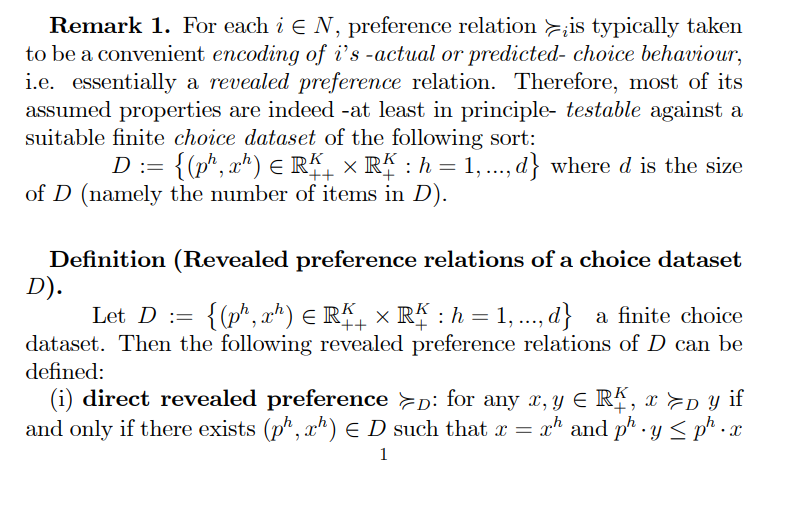
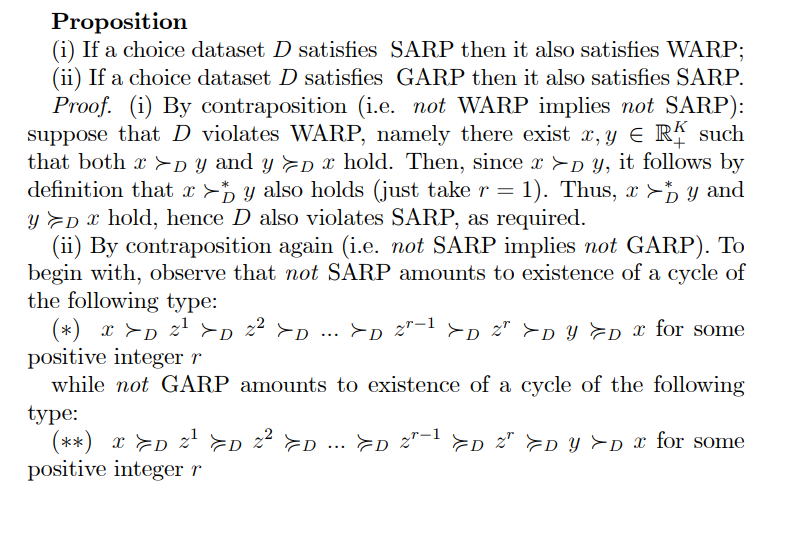
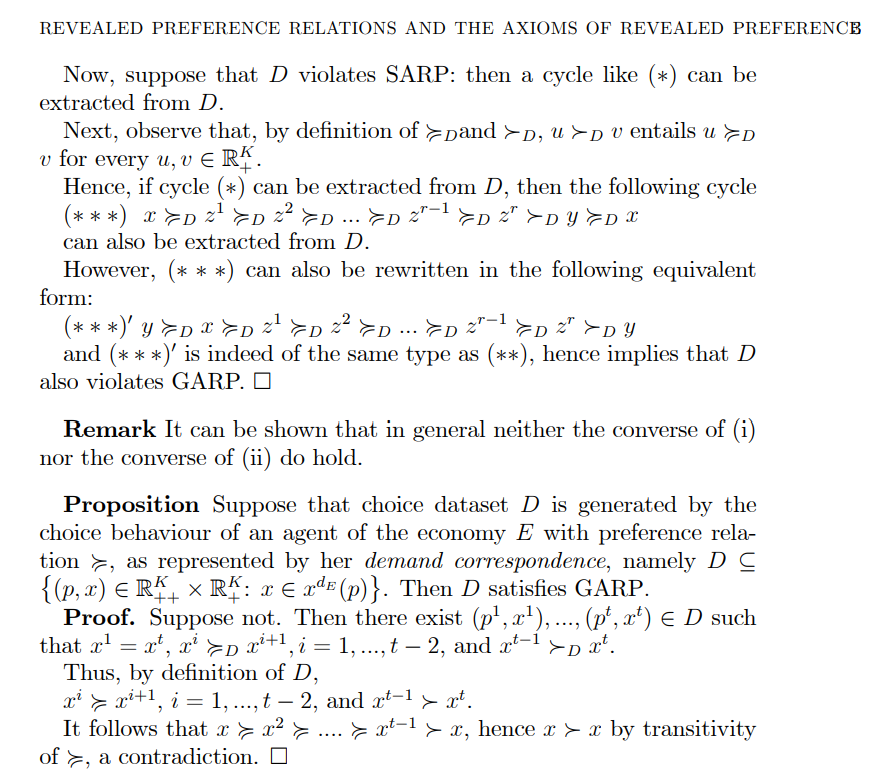
REVEALED PREFERENCE RELATIONS AND THE AXIOMS OF REVEALED PREFERENCE A pure exchange economy with n 2 2 agents and m 2 2 private, privately owned and perfectly divisible goods -for some integers n, m- is an array E : (N, (Xi: Maj, kihEN) where N = {1, ..., n} is the index set of agents, K : {1, ...,k} is the index set of goods, and for any i E N, X,- : Rf is the consumption set of agent i w,- E Rf \\ {U} is the endowment of agent i >,,-g X,- x X, is the continuous binary preference relation of agent i (i.e. for every :t: E X,- both the upper contour set {2 E X,- : z m :12}and the lower contour set {2: E X, : :1: r,- z} of mat 1: are closed in the Euclidean metric topology): it is usually also assumed to be locally nowsatinbie, convent, and a total pr'eorder (i.e. reexive, transitive and connected). For each agent (or consumer) i E N, the demand correspondence me : Rig ) Rf is defined as follows: for any 3:) E R541, (,3 __ mERfm-iESP'we, m,(p). and$%,yforallyERfsuchthatp-ySP'Wz' - Remark 1. For each i E N, preference relation > is typically taken to be a convenient encoding of i's -actual or predicted- choice behaviour, i.e. essentially a revealed preference relation. Therefore, most of its assumed properties are indeed -at least in principle- testable against a suitable finite choice dataset of the following sort: D := {(p, x) ERk X RA : h =1, ...,d} where d is the size of D (namely the number of items in D). Definition (Revealed preference relations of a choice dataset D). Let D := {(ph, xh) ERK, X RK : h =1, ...,d) a finite choice dataset. Then the following revealed preference relations of D can be defined: (i) direct revealed preference > p: for any x, y E RK, x / D y if and only if there exists (ph, x*) E D such that ax = x and phy p: for any x, y E RA, x > D y if and only if there exists (ph, ph) E D such that x = ah and ph . y > :for any x, y E RK, x > > y if and only if there exists a positive integer r and (pl, z! ), ..., (p", z") E D such that x 20 21, 21 20 24+1 for each i = 1, ..., r - 1, and 2" FD y (iv) indirect strict revealed preference > > :for any x, y E RA, x > b y if and only if there exists a positive integer r and (pl, z]), ..., (p", z") E D such that x > D 21, 2' >D 27+1 for each i = 1, ..., r - 1, and 2" > D y. The axioms of revealed preference Weak Axiom of Revealed Preference (WARP): A choice dataset D = {(ph, xh) ERK x RK : h =1, ...,d, satis- fies WARP if, for any x, y E RA, [x >D y entails not y ED x]. Strong Axiom of Revealed Preference (SARP): A choice dataset D = {(ph, xh) E RK x RK : h =1, ...,d, satis- fies SARP if, for any x, y ERA, [x >by entails not y zD x]. Generalized Axiom of Revealed Preference (GARP): A choice dataset D = {(ph, xh) E RK, x RK : h =1, ...,d, satis- fies GARP if, for any x, y E RA, [x >), y entails not y > D x].Proposition (i) If a choice dataset D satisfies SARP then it also satisfies WARP; (ii) If a choice dataset D satisfies GARP then it also satisfies SARP. Proof. (i) By contraposition (i.e. not WARP implies not SARP): suppose that D violates WARP, namely there exist x, y E R^ such that both x >Dy and y > p x hold. Then, since > >p y, it follows by definition that > >b y also holds (just take r = 1). Thus, x >b y and y > D x hold, hence D also violates SARP, as required. (ii) By contraposition again (i.e. not SARP implies not GARP). To begin with, observe that not SARP amounts to existence of a cycle of the following type: (*) >DZ DZ >D ... XD 2 XD 2 >D U ED * for some positive integer r while not GARP amounts to existence of a cycle of the following type: (* *) XD202 XD ...>D2 >D 2" ED y XD x for some positive integer rREVEALED PREFERENCE RELATIONS AND THE AXIOMS 0F REVEALED PREFERENCE Now, suppose that D violates SARP: then a cycle like (at) can be extracted from D. Next, observe that, by denition of kgand >13, n >1) 1.! entails in kg. 1: for every n, v 6 RE. Hence, if cycle (1:) can be extracted from D, then the following cycle (* * *) 37 %D 21 ho 2'2 kn kn Z151 kn Zr >13 3; ho 23 can also be extracted from D. However, (:1: a: *) can also be rewritten in the following equivalent form: (* * *)i 3; ho 11? kn 21 #D 32 ED kn 2'51 kn Zr >10 9! and (:1: a: as)' is indeed of the same type as (3101:), hence implies that D also violates CARP. III Remark It can be shown that in general neither the converse of (i) nor the converse of (ii) do hold. Proposition Suppose that choice dataset D is generated by the choice behaviour of an agent of the economy E with preference rela tion , as represented by her demand correspondence, namely D Q {(39, :5) E Rf; >13 mt. Thus, by definitiOn of D, of % zr+1,= 1, ...,t 2, and art1 > 37*. It follows that a: k 2:2 r % 12*'1 > 5?, hence :5 > a: by transitivity of %1 a cOntradictiOn. El