







I need help asap with these followed steps on this question. These are the examples that follow the questions which are 3 questions.
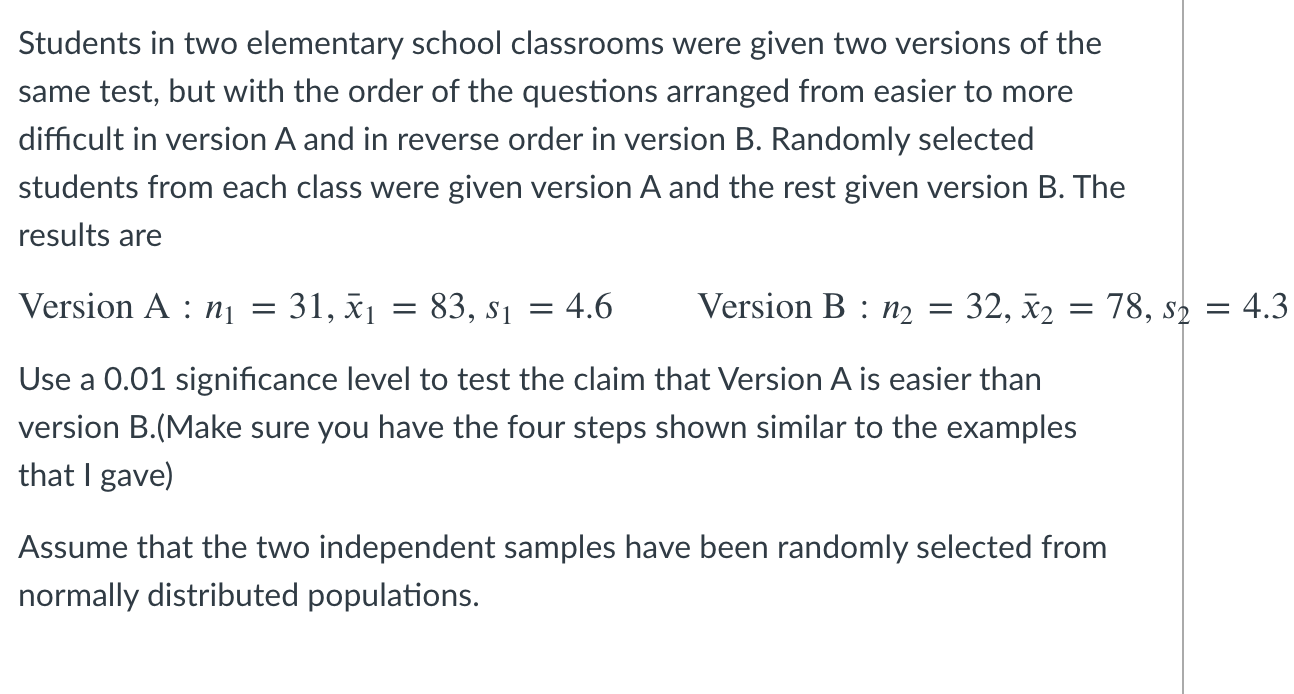
Five students took a math test before and after tutoring. Their scores were as followed: Subject A B C D E Before 71 66 75 78 66 After 75 75 73 81 78 Use a 0.01 level of significance to test the claim that the tutoring has an effect on increasing the math scores. (Make sure you have the four steps shown similar to the examples that I gave) Assume that two dependent samples have been randomly selected from normally distributed populations.lnstruction: 1) Must contains 4 steps as shown on the instructor's video. alternative hypothesis is the claim. 3) When doing the calculation, do not calculate standard error. from the textbook. A marketing survey involves product recognition in New York and California. Of 558 New Yorkers surveyed, 193 knew the product while 196 out of 614 Californians knew the product. At the 0.05 significance level, test the claim that the recognition rates are the same in both states. (Make sure you have the four steps shown similar to the examples that I gave)lnstruction: 1) Must contains 4 steps as shown on the instructor's video. alternative hypothesis is the claim. 3) When doing the calculation, do not calculate standard error. from the textbook. Students in two elementary school classrooms were given two versions of the same test, but with the order of the questions arranged from easier to more difficult in version A and in reverse order in version B. Randomly selected students from each class were given version A and the rest given version B. The results are Version A : n1 = 31, x1 = 83, $1 = 4.6 Version B : n2 = 32, x2 = 78, s = 4.3 Use a 0.01 significance level to test the claim that Version A is easier than version B.(Make sure you have the four steps shown similar to the examples that I gave) Assume that the two independent samples have been randomly selected from normally distributed populations.lnstruction: 1) Must contains 4 steps as shown on the instructor's video. alternative hypothesis is the claim. 3) When doing the calculation, do not calculate standard error. from the textbook. Wording the Final Conclusion Condition Conclusion Original claim does not include "There is sufficient evidence to equality, and you reject Ho- support the claim that ... (original claim) Original claim does not include There is not sufficient evidence equality, and you fail to reject Ho. to support the claim that ... (original claim)" Original claim includes equality, There is sufficient evidence to and you reject Ho- warrant rejection of the claim that ... (original claim)." Original claim includes equality, "There is not sufficient evidence and you fail to reject Ho- to warrant rejection of the claim that ... (original claim)."Given into Cliven confidence level. 95 %% = = 1- 95%= 5% Want : Confidence Interval of the proportion. sample Then the sample proportion F- 4 mean the 3 2618 . E statistic P = 2 13 = 420101 - 0. 0 003 262 furth decimal = 3.26/8 = 0 DJ0 32 6 7 8 critical The critical value is value Zo = Inv Norm ( 1 - 2 ) = Inv Norm ( 1 - 02 ) = Inv Norm ( 0.97 5 ) ~ 1.96 Heme the margin of error 0. 0 01 3 262 (1- 20003 262) E = 28 = 196 420 005 - 1.96 0. 070 3 26 2 . 0. 9 9 96738 420 005 - 1. 96 . 0 0 0 0 0 2786 - 0.01005461~ 1.76 Heme the margin of error margin of A A D. Dad 3 262 ( 1- 20003 262) E = 23 = 196 error n 420 DO 5 - 1.96 0. DDO 3 2 6 2 . 0. 9 9 9 67 38 420 005 - 1. 96 . 0. 0 0 0 0 2786 - 0 01005461 calculation The the confidence interval is ( a , b ) = ( 202716 %% 2038 8 % ) where a = P - E = 1000 } 262 - 0.0000 5 461 the confidence ~ 0.00 02716 = 0.02716 % internal b = P + E = 0 0 0 0 3 2 62 + 0.0010 5 461 ~ 0 00 0 38 08 = 0 03808 %Therefore , we are 95 % confident that the interval Interpretation from 0.027 16 1% to 0. 03808 % actually contains the true population proportion of the cell phone users who developed cancer of the brain or nervous system

























