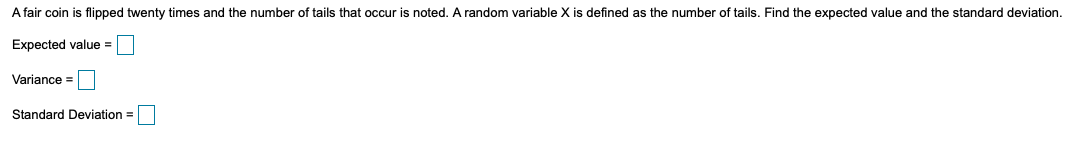I need help understanding these practice problems, please explain the steps.

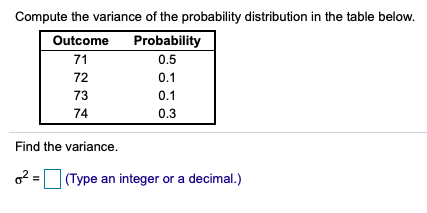
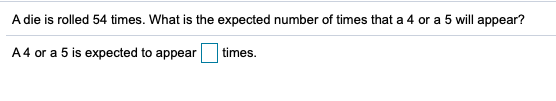
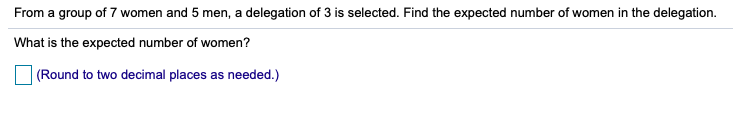
If the probability distribution for the random variable X is given in the table, what is the expected value of X? Xi - 3 1 5 P .3 .5 .2 E(X) (Type an integer or a decimal.)Compute the variance of the probability distribution in the table below. Outcome Probability 71 0.5 72 0.1 73 0.1 74 0.3 Find the variance. (Type an integer or a decimal.)One option in a roulette game is to bet $11 on red. (There are 18 red compartments, 18 black compartments, and 2 compartments that are neither red nor black.) If the ball lands on red, you get to keep the $11 you paid to play the game, and you are awarded $12. If the ball lands elsewhere, you are awarded nothing, and the $11 that you bet is collected. Write down the probability distribution for the earnings from this type of bet and find the expected value. Give the probability distribution. Earnings Probability 0 Type an exact answer in simplified form.)Five thousand tickets are sold at $1 each for a charity raffle. Tickets are to be drawn at random and monetary prizes awarded as follows: 1 prize of $700, 3 prizes of $300, 5 prizes of $10, and 20 prizes of $5. What is the expected value of this raffle if you buy 1 ticket? a. Let X be the random variable for the amount won on a single raffle ticket. Complete the payoff table for the random variable X. Xi (Enter the net amount won in decreasing order.) Pi (Type probabilities as decimals.)Several psychology students are unprepared for a surprise true/false test with 13 questions, and all of their answers are guesses. Find the mean and standard deviation for the number of correct answers for such students.A fair coin is flipped twenty times and the number of tails that occur is noted. A random variable X is defined as the number of tails. Find the expected value and the standard deviation. Expected value = Variance = Standard Deviation =A box of 10 flashbulbs contains 2 defective bulbs. A random sample of 2 is selected and tested. Let X be the random variable associated with the number of defective bulbs in the sample. a. Find the probability distribution of X. b. Find the expected number of defective bulbs in a sample. a. Complete the probability distribution for X. (Enter the number of defective bulbs in increasing order.) (Type probabilities as simplified fractions.)A die is rolled 54 times. What is the expected number of times that a 4 or a 5 will appear? A4 or a 5 is expected to appear times.Two candidates are running for president: John Democrat and Joe Republican. A recent survey concluded that Joe Republican had a probability of 0.56 to win the election. Two hundred people are interviewed at random about whom they would vote for. A random variable X is defined as the number of people who said they would vote for John Democrat. Find the expected value of the random variable Expected Value =Complete parts (a) and (b) below. The number of dogs per household in a small town Dogs 2 4 5 Probability 0.653 0.216 0.089 0.021 0.013 0.008 (a) Find the mean, variance, and standard deviation of the probability distribution. Find the mean of the probability distribution. (Round to one decimal place as needed.)From a group of 7 women and 5 men, a delegation of 3 is selected. Find the expected number of women in the delegation. What is the expected number of women? (Round to two decimal places as needed.)A manufacturer produces widgets that are packaged in boxes of 200. The probability of a widget being defective is 0.013. Find the mean and standard deviation for the number of defective widgets in a box The mean, J, is . (Round to the nearest hundredth as needed.)