I need help with number 3 number 2 is there for reference 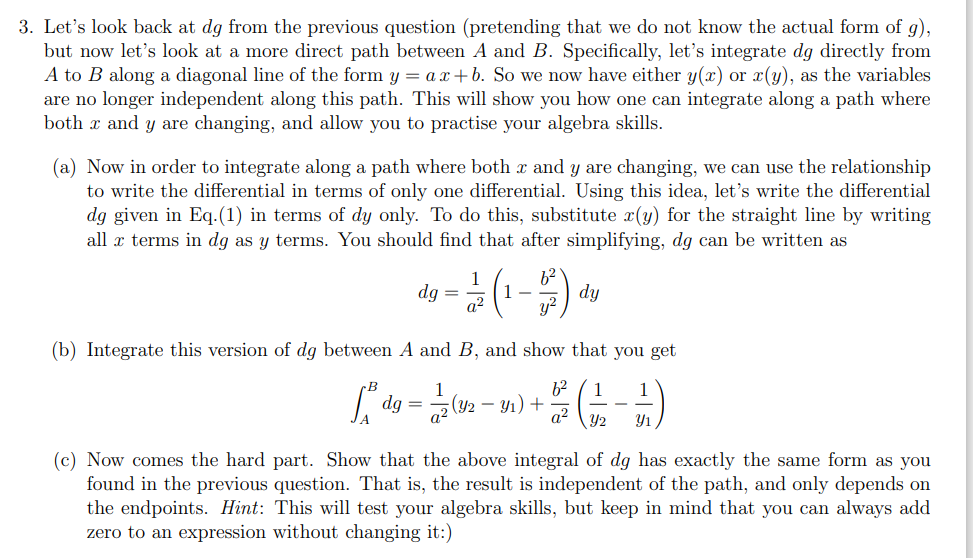
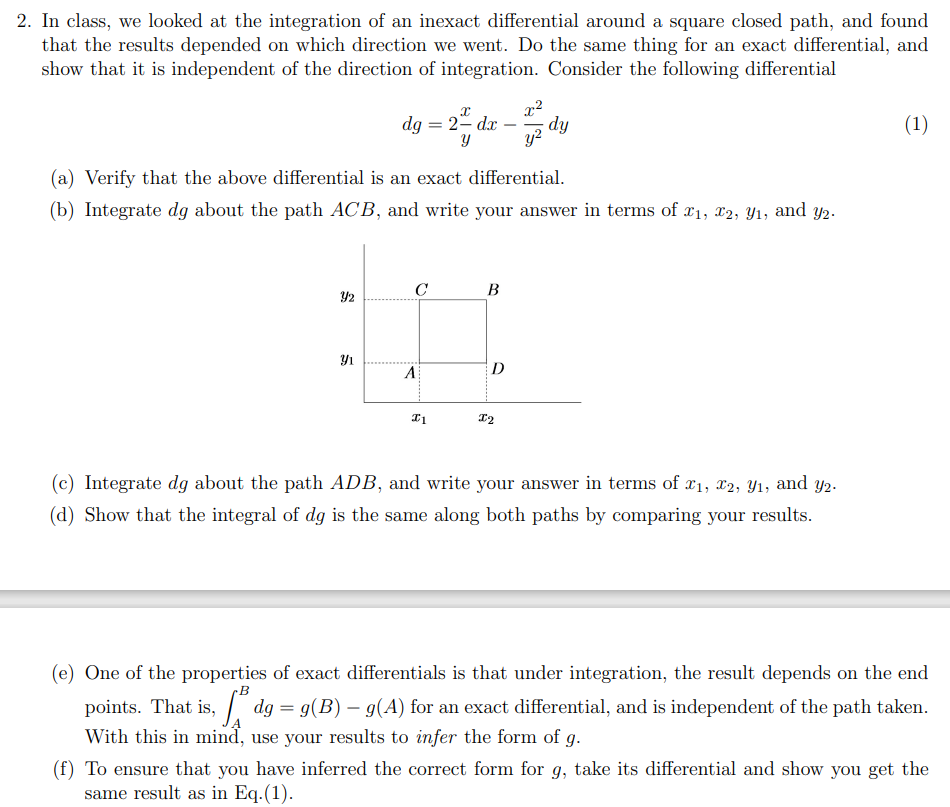
3. Let's look back at dg from the previous question (pretending that we do not know the actual form of g), but now let's look at a more direct path between A and B. Specifically, let's integrate dg directly from A to B along a diagonal line of the form y = ax+b. So we now have either y(x) or (y), as the variables are no longer independent along this path. This will show you how one can integrate along a path where both x and y are changing, and allow you to practise your algebra skills. (a) Now in order to integrate along a path where both x and y are changing, we can use the relationship y to write the differential in terms of only one differential. Using this idea, let's write the differential dg given in Eq.(1) in terms of dy only. To do this, substitute z(y) for the straight line by writing all x terms in dg as y terms. You should find that after simplifying, dg can be written as dg 1 a2 (-) 62 1 - y2 dy (b) Integrate this version of dg between A and B, and show that you get 1 62 / 1 1 al y S dg = (12 9)+ + Yi (c) Now comes the hard part. Show that the above integral of dg has exactly the same form as you found in the previous question. That is, the result is independent of the path, and only depends on the endpoints. Hint: This will test your algebra skills, but keep in mind that you can always add zero to an expression without changing it:) 2. In class, we looked at the integration of an inexact differential around a square closed path, and found that the results depended on which direction we went. Do the same thing for an exact differential, and show that it is independent of the direction of integration. Consider the following differential x2 C dg = 2- dx y dy y2 (1) (a) Verify that the above differential is an exact differential. (b) Integrate dg about the path ACB, and write your answer in terms of 21, 12, 41, and y2. Y2 B yi A D 11 12 (C) Integrate dg about the path ADB, and write your answer in terms of 21, 22, 41, and y2. (d) Show that the integral of dg is the same along both paths by comparing your results. (e) One of the properties of exact differentials is that under integration, the result depends on the end points. That is, dg=g(B) 9(A) for an exact differential, and is independent of the path taken. With this in mind, use your results to infer the form of g. (f) To ensure that you have inferred the correct form for g, take its differential and show you get the same result as in Eq.(1). 3. Let's look back at dg from the previous question (pretending that we do not know the actual form of g), but now let's look at a more direct path between A and B. Specifically, let's integrate dg directly from A to B along a diagonal line of the form y = ax+b. So we now have either y(x) or (y), as the variables are no longer independent along this path. This will show you how one can integrate along a path where both x and y are changing, and allow you to practise your algebra skills. (a) Now in order to integrate along a path where both x and y are changing, we can use the relationship y to write the differential in terms of only one differential. Using this idea, let's write the differential dg given in Eq.(1) in terms of dy only. To do this, substitute z(y) for the straight line by writing all x terms in dg as y terms. You should find that after simplifying, dg can be written as dg 1 a2 (-) 62 1 - y2 dy (b) Integrate this version of dg between A and B, and show that you get 1 62 / 1 1 al y S dg = (12 9)+ + Yi (c) Now comes the hard part. Show that the above integral of dg has exactly the same form as you found in the previous question. That is, the result is independent of the path, and only depends on the endpoints. Hint: This will test your algebra skills, but keep in mind that you can always add zero to an expression without changing it:) 2. In class, we looked at the integration of an inexact differential around a square closed path, and found that the results depended on which direction we went. Do the same thing for an exact differential, and show that it is independent of the direction of integration. Consider the following differential x2 C dg = 2- dx y dy y2 (1) (a) Verify that the above differential is an exact differential. (b) Integrate dg about the path ACB, and write your answer in terms of 21, 12, 41, and y2. Y2 B yi A D 11 12 (C) Integrate dg about the path ADB, and write your answer in terms of 21, 22, 41, and y2. (d) Show that the integral of dg is the same along both paths by comparing your results. (e) One of the properties of exact differentials is that under integration, the result depends on the end points. That is, dg=g(B) 9(A) for an exact differential, and is independent of the path taken. With this in mind, use your results to infer the form of g. (f) To ensure that you have inferred the correct form for g, take its differential and show you get the same result as in Eq.(1)








