Answered step by step
Verified Expert Solution
Question
1 Approved Answer
I need help with this question, particularly 1a. I have to answer this in Python coding format. This particular snip is of Python but in
I need help with this question, particularly 1a. I have to answer this in Python coding format. This particular snip is of Python but in Jupyter Notebook. Please explain the code you are using and what exactly you are doing and why so I can follow easily. Thank you for your help! It only allows me to pick one subject, but this class is called Environmental Modeling and Health and is essentially using mathematical models in Python to explain environmental behavior. 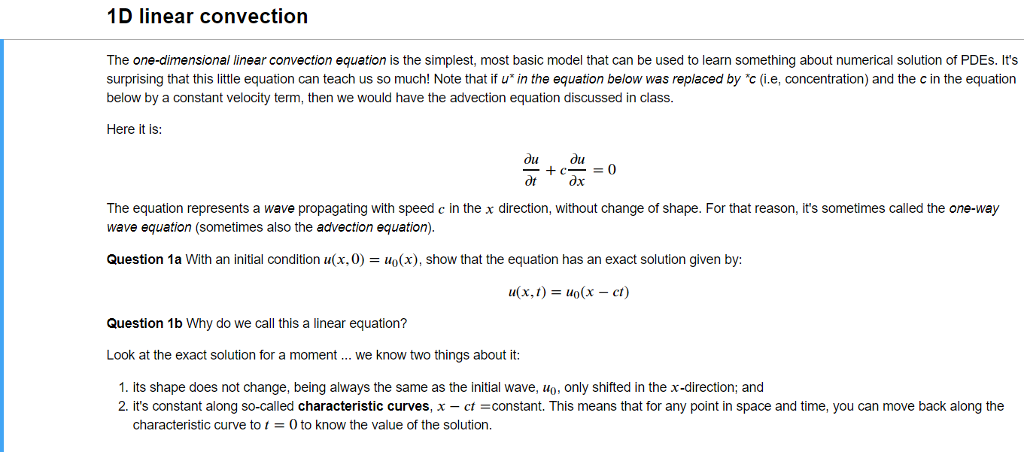
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


