Question: 2. Let X be a Poisson random variable with parameter > 0, probability function 1e-A x! and moment generating function M(t) = (-1) (a)

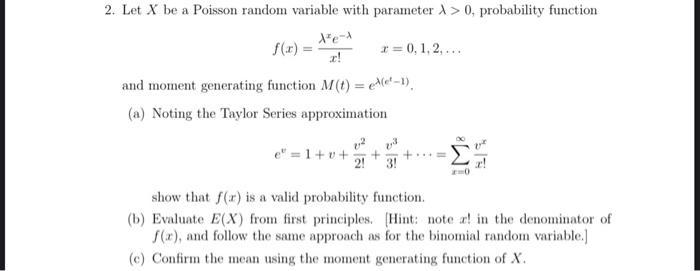
2. Let X be a Poisson random variable with parameter > 0,

2. Let X be a Poisson random variable with parameter > 0, probability function 1e-A x! and moment generating function M(t) = (-1) (a) Noting the Taylor Series approximation f(x) = e" =1+v+ x = 0, 1, 2,... 23 + 21 3! show that f(r) is a valid probability function. (b) Evaluate E(X) from first principles. (Hint: note a! in the denominator of f(x), and follow the same approach as for the binomial random variable.] (c) Confirm the mean using the moment generating function of X.

Step by Step Solution

★★★★★

3.41 Rating (151 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock

a In this exercise we need to know if fx satisfies img 1 The proof is in the image 2 b This on... View full answer

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Mathematics Questions!

In this assignment you need to write a program in MIPS assembly language. Your program should perform following tasks: It should prompt the user to enter five numbers one by one. Once user has...

Distinguish between an in-the-money call option and an in-the-money put option. In your response, you should identify specific options from the above XYZ option quote table that are in-the-money and...

Straightforward calculation of overhead variances: manufacturer The data below relate to Sporty Ltd, a manufacturer of exercise equipment, for August: Standard variable overhead rate $27 per machine...

If Xi, i = 1, . . . , n are independent normal random variables, with Xi having mean i and variance 1, then the random variable n i=1X2 i is said to be a noncentral chi-squared random variable. (a)...

42. If Xi , i = 1, . . . , n are independent normal random variables, with Xi having mean i and variance 1, then the random variable ni =1 X2 i is said to be a noncentral chi-squared random variable....

Consider an rv X with mean m and standard deviation s, and let g(X) be a specified function of X. The first-order Taylor series approximation to g(X) in the neighborhood of m is g(X) g() + g(m) (X...

Let N be a Poisson random variable with parameter ?. Write a one-line R command for simulating where X 1 ,X 2 , . . . are i.id. normal random variables with parameters ? and ? 2 . Now show how to...

See the previous exercise. Suppose ? = 10 and ? = ? = 1. It might be tempting to believe that the distribution of Z is normal with mean 10 and variance 20. (Why might someone make this mistake?) Give...

Recall the Coupon Collectors Problem described in Exercise 106 of Chapter 2. Let X = the number of cereal boxes purchased in order to obtain all 10 coupons. a. Use a simulation program to estimate...

151. Consider an rv X with mean m and standard deviation s, and let g(X) be a specified function of X. The first-order Taylor series approximation to g(X) in the neighborhood of m is The right-hand...

please answer all the questionss.,.within 30 minutes. make sure the explanation and calculations are explained in very detailed manner. else leave it for other tutor otherwise i will give negative...

You have $375,000 to invest in a share portfolio. Your choices are as follows: Invest in FTV with an expected return of 14.25%. Invest in FHL with an expected return of 8.7%. FTV has a beta of 1.58....

1-18 Find the volume of the solid obtained by rotating the region bounded by the given curves about the specified line. Sketch the region, the solid, and a typical disk or washer. 1. y=2x, y = 0, x =...

about the case study "The Westerville Physician Practice: Value-Stream Mapping" on Pages 445 - 448 of our textbook, total wait time is 111minutes, processing time is 67minutes. I have questions about...

1. Your friend asks you to invest $10,000 in a business venture. Based on your estimates, you would receive nothing for four years, at the end of year 5 you would receive interest on the investment...

1 ) A global contracting organisation suffers from different types of organisational conflicts internally and within its clients and supply chain. You are appointed as a consultant to advise senior...

Jane, a new partner in a CPA firm, borrows $40,000 from one of her firm's audit clients to upgrade her vacation home. She provides no services to the client, does not work in the same office as the...

Cable Corporation is 60% owned by Anna and 40% owned by Jim, who are unrelated. It has noncash assets, which it sells to an unrelated purchaser for $100,000 in cash and $900,000 in installment...

Glass viewing windows are to be installed in an aquarium. Each window is to be 0.6 m in diameter and to be centered 2 m below the surface level. Find the force and location of the force acting on the...

Water flows in the channels between two aluminum plates as shown in the sketch. The ribs that form the channels are also made of aluminum and are 8-mm thick. The effective surface coefficient between...

Determine the difference in pressure between the inside and outside of a soap film bubble at 20C if the diameter of the bubble is 4 mm.

A distributor orders four products from a supplier. The fixed cost to place an order for one SKU is $23, and cost of each additional SKU added to the order is $3. The carrying cost rate is 24 percent...

A manager wants to purchase a new piece of processing equipment and must decide on tire number of a spare part to order with the new equipment. The spares cost $200 each, and any unused spares will...

Hall mark sells personal expression cards and gifts wrorldwride through either its own stores or other retailers. Most products are single runs. Unsold products are either discounted and sold to...