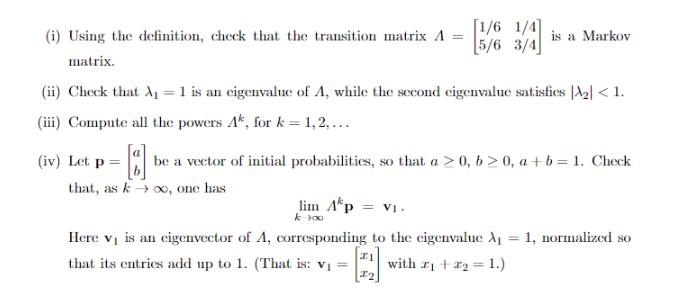
(i) Using the definition, check that the transition matrix A matrix. [1/6 1/4] 5/6 3/4 is a Markov (ii) Check that A = 1 is an eigenvalue of A, while the second eigenvalue satisfies |2| < 1. (iii) Compute all the powers A, for k = 1, 2,... (iv) Let p = be a vector of initial probabilities, so that a > 0, b0, a+b=1. Check that, as koo, one has lim Ap = VI. k 100 Here v is an eigenvector of A, corresponding to the eigenvalue A = 1, normalized so that its entries add up to 1. (That is: v = with 1+2=1.) 21 #2