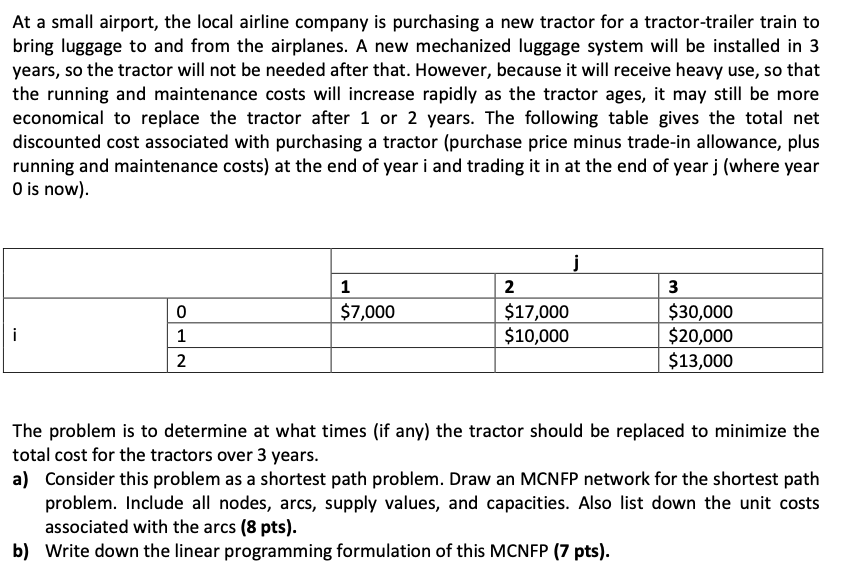
I will be very happy if you can solve it in an understandable way :) Thank you!!!

At a small airport, the local airline company is purchasing a new tractor for a tractor-trailer train to bring luggage to and from the airplanes. A new mechanized luggage system will be installed in 3 years, so the tractor will not be needed after that. However, because it will receive heavy use, so that the running and maintenance costs will increase rapidly as the tractor ages, it may still be more economical to replace the tractor after 1 or 2 years. The following table gives the total net discounted cost associated with purchasing a tractor (purchase price minus trade-in allowance, plus running and maintenance costs) at the end of year i and trading it in at the end of year j (where year 0 is now). The problem is to determine at what times (if any) the tractor should be replaced to minimize the total cost for the tractors over 3 years. a) Consider this problem as a shortest path problem. Draw an MCNFP network for the shortest path problem. Include all nodes, arcs, supply values, and capacities. Also list down the unit costs associated with the arcs (8 pts). b) Write down the linear programming formulation of this MCNFP (7 pts). At a small airport, the local airline company is purchasing a new tractor for a tractor-trailer train to bring luggage to and from the airplanes. A new mechanized luggage system will be installed in 3 years, so the tractor will not be needed after that. However, because it will receive heavy use, so that the running and maintenance costs will increase rapidly as the tractor ages, it may still be more economical to replace the tractor after 1 or 2 years. The following table gives the total net discounted cost associated with purchasing a tractor (purchase price minus trade-in allowance, plus running and maintenance costs) at the end of year i and trading it in at the end of year j (where year 0 is now). The problem is to determine at what times (if any) the tractor should be replaced to minimize the total cost for the tractors over 3 years. a) Consider this problem as a shortest path problem. Draw an MCNFP network for the shortest path problem. Include all nodes, arcs, supply values, and capacities. Also list down the unit costs associated with the arcs (8 pts). b) Write down the linear programming formulation of this MCNFP (7 pts)