I will use this as reviewer on our upcoming exam this week hope you can help me~
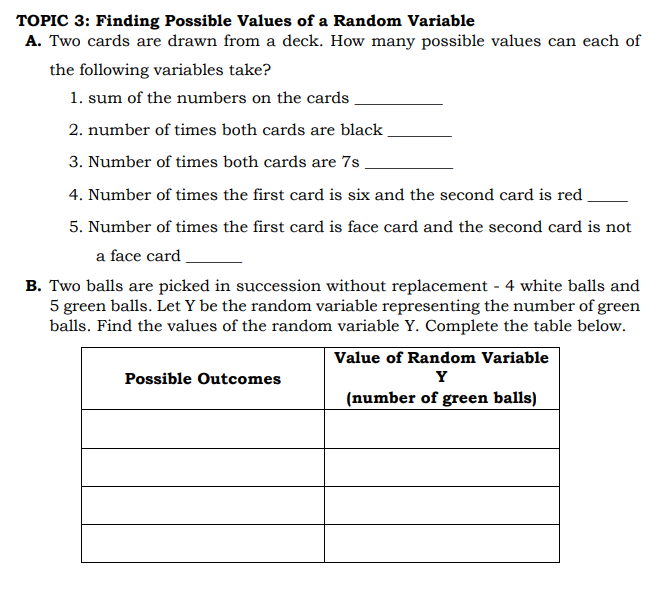
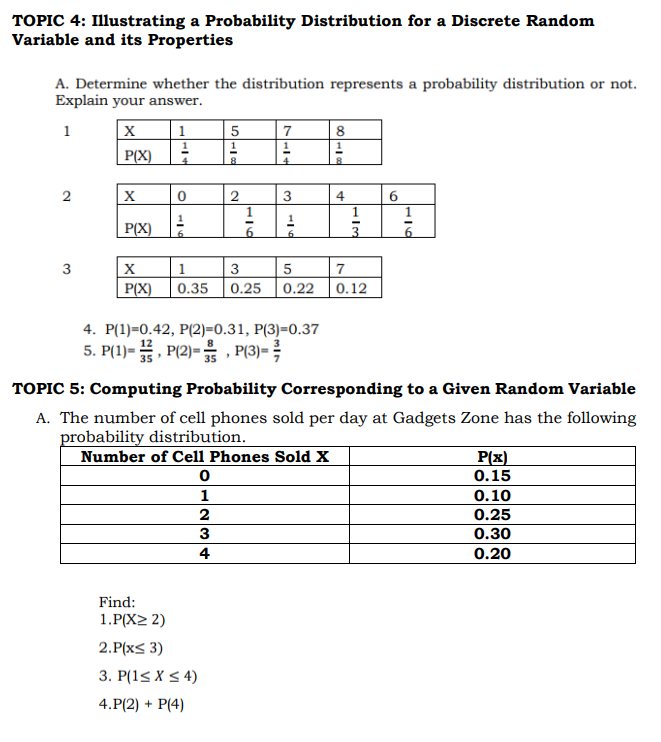
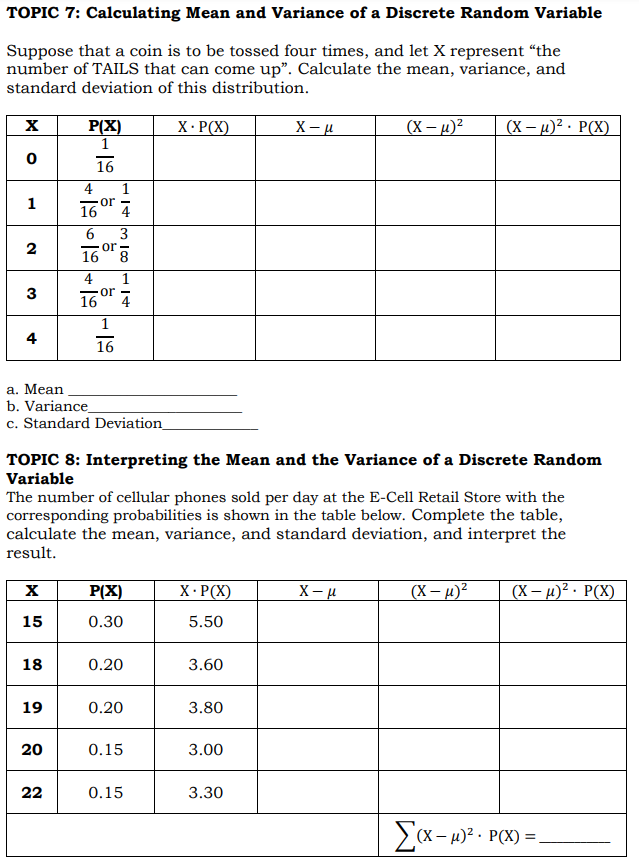
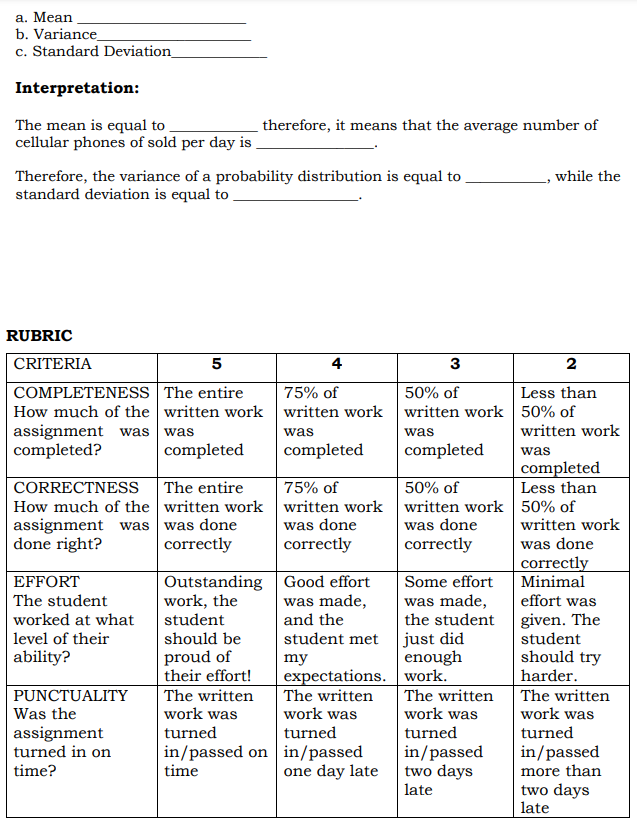
TOPIC 1: Illustrating a Random Variable (Discrete and Continuous) Complete the table below by identifying the number X or the random variable X and type of random variable. EXPERIMENT Number X or the Type of Random Random Variable X Variable 1. Number of rings before the phone is answered 2. Teacher ask the students to finish the test after an hour 3. Number of complaints per day 4. Height of the tallest building in Lucena City 5. Number of Mobile phones in a household TOPIC 2: Distinguishing Between a Discrete and a Continuous Random Variable Classify the following random variables as discrete or continuous. 1. the speed of a tricycle. 2. the number of female students. 3. the time needed to finish the module. 4. the amount of sugar in a cup of coffee. 5. The number of defective mobile phones produced by a manufacturer.TOPIC 3: Finding Possible Values of a Random Variable A. Two cards are drawn from a deck. How many possible values can each of the following variables take? 1. sum of the numbers on the cards 2. number of times both cards are black 3. Number of times both cards are 7s 4. Number of times the first card is six and the second card is red 5. Number of times the first card is face card and the second card is not a face card B. Two balls are picked in succession without replacement - 4 white balls and 5 green balls. Let Y be the random variable representing the number of green balls. Find the values of the random variable Y. Complete the table below. Value of Random Variable Possible Outcomes Y (number of green balls)TOPIC 4: Illustrating a Probability Distribution for a Discrete Random Variable and its Properties A. Determine whether the distribution represents a probability distribution or not. Explain your answer. 1 X 1 5 7 8 P(X) 2 X 0 2 3 4 6 P(X) 3 X 1 3 5 7 P(X) 0.35 0.25 0.22 0.12 4. P(1)=0.42, P(2)=0.31, P(3)=0.37 5. P(1)= =, P(2)=, P(3)= TOPIC 5: Computing Probability Corresponding to a Given Random Variable A. The number of cell phones sold per day at Gadgets Zone has the following probability distribution. Number of Cell Phones Sold X P(x) 0 0.15 0.10 2 0.25 0.30 4 0.20 Find: 1.P(X2 2) 2.P(xS 3) 3. P(1S X S 4) 4.P(2) + P(4)TDPID 7: Calculating Mean and Variance cf a Discrete Random Variable Suppose that a coin is to be tossed four times, and let X represent \"the number of TAILS that can come up\". Calculate the mean, variance, and standard deviation of this distribution. a. Mean. 1]. 1|I..I'a_tiance c. Standard Deviation TDPIC B: Interpreting the Mean and the Variance of a Discrete Random Variable The number of cellular phones sold per day at the ECell Retail Store with the corresponding probabilities is shown in the table below. Complete the table, calculate the mean, variance, and standard deviation, and interpret the result. a. Mean b. Variance c. Standard Deviation Interpretation: The mean is equal to therefore, it means that the average number of cellular phones of sold per day is Therefore, the variance of a probability distribution is equal to while the standard deviation is equal to RUBRIC CRITERIA 5 4 3 2 COMPLETENESS The entire 75% of 50% of Less than How much of the written work written work written work 50% of assignment was was was was written work completed? completed completed completed was completed CORRECTNESS The entire 75% of 50% of Less than How much of the written work written work written work 50% of assignment was was done was done was done written work done right? correctly correctly correctly was done correctly EFFORT Outstanding Good effort Some effort Minimal The student work, the was made, was made, effort was worked at what student and the the student given. The level of their should be student met just did student ability? proud of my enough should try their effort! expectations. work. harder. PUNCTUALITY The written The written The written The written Was the work was work was work was work was assignment turned turned turned turned turned in on in /passed on in /passed in / passed in / passed time? time one day late two days more than late two days late















