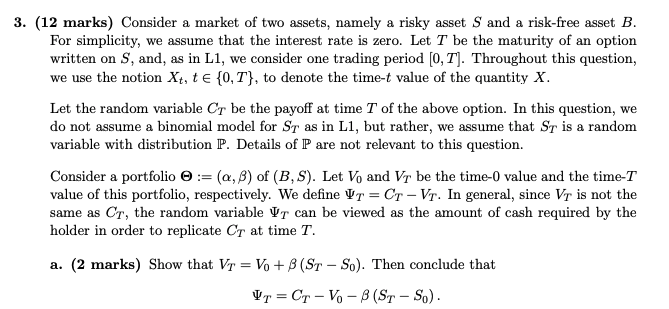
I will vote up quickly if the answer is correct. Thanks!

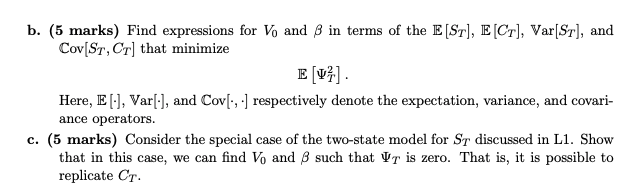
3. (12 marks) Consider a market of two assets, namely a risky asset S and a risk-free asset B. For simplicity, we assume that the interest rate is zero. Let T be the maturity of an option written on S, and, as in Li, we consider one trading period [0,T]. Throughout this question, we use the notion Xt, t {0, T}, to denote the time-t value of the quantity X. Let the random variable Cr be the payoff at time of the above option. In this question, we do not assume a binomial model for Sr as in L1, but rather, we assume that Sr is a random variable with distribution P. Details of P are not relevant to this question. Consider a portfolio @ :=(a,b) of (B, S). Let V, and V be the time-0 value and the time-T value of this portfolio, respectively. We define VT = Cr-Vr. In general, since Vr is not the same as Cr, the random variable Ut can be viewed as the amount of cash required by the holder in order to replicate CT at time T. a. (2 marks) Show that Vr = V0 + B (ST - S.). Then conclude that UT=Cr-V-B (ST-S.). b. (5 marks) Find expressions for Vo and 8 in terms of the E (ST), E (Cr), Var[ST], and Cov[St, Cr] that minimize E [v] Here, E[:], Var(-), and Cov , -] respectively denote the expectation, variance, and covari- ance operators. c. (5 marks) Consider the special case of the two-state model for Sy discussed in Li. Show that in this case, we can find V, and 8 such that U is zero. That is, it is possible to replicate Cr 3. (12 marks) Consider a market of two assets, namely a risky asset S and a risk-free asset B. For simplicity, we assume that the interest rate is zero. Let T be the maturity of an option written on S, and, as in Li, we consider one trading period [0,T]. Throughout this question, we use the notion Xt, t {0, T}, to denote the time-t value of the quantity X. Let the random variable Cr be the payoff at time of the above option. In this question, we do not assume a binomial model for Sr as in L1, but rather, we assume that Sr is a random variable with distribution P. Details of P are not relevant to this question. Consider a portfolio @ :=(a,b) of (B, S). Let V, and V be the time-0 value and the time-T value of this portfolio, respectively. We define VT = Cr-Vr. In general, since Vr is not the same as Cr, the random variable Ut can be viewed as the amount of cash required by the holder in order to replicate CT at time T. a. (2 marks) Show that Vr = V0 + B (ST - S.). Then conclude that UT=Cr-V-B (ST-S.). b. (5 marks) Find expressions for Vo and 8 in terms of the E (ST), E (Cr), Var[ST], and Cov[St, Cr] that minimize E [v] Here, E[:], Var(-), and Cov , -] respectively denote the expectation, variance, and covari- ance operators. c. (5 marks) Consider the special case of the two-state model for Sy discussed in Li. Show that in this case, we can find V, and 8 such that U is zero. That is, it is possible to replicate Cr