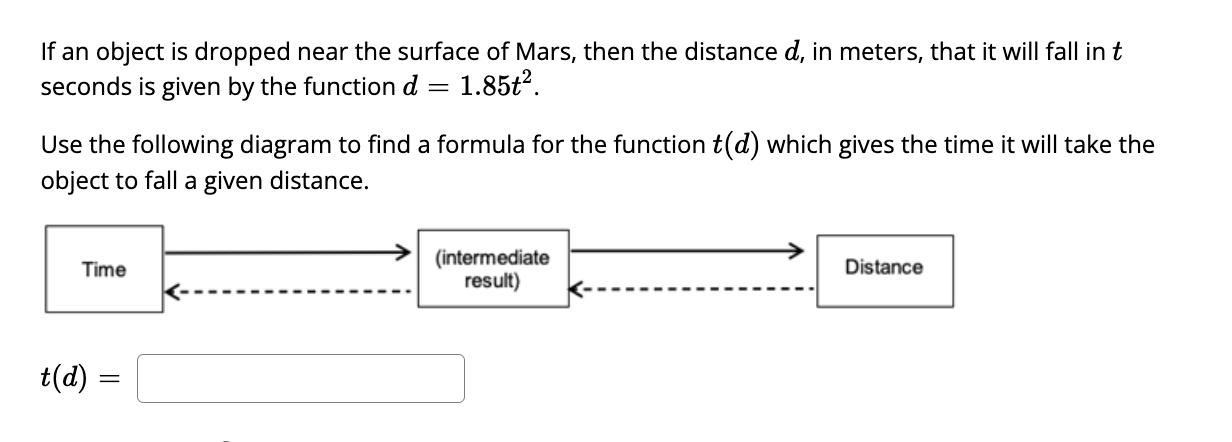
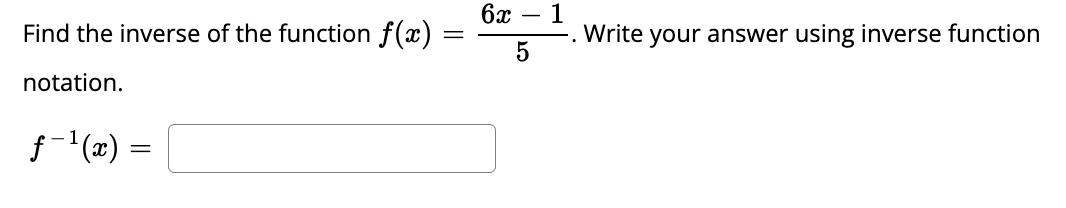
If an object is dropped near the surface of Mars, then the distance d, in meters, that it will fall in t seconds is given by the function d = 1.85t. Use the following diagram to find a formula for the function t(d) which gives the time it will take the object to fall a given distance. Time t(d) = = (intermediate result) Distance 7 2 Part A: Give the coordinates of the vertex of f(x). Let f(x) 1-1/72- + x + Answer: Part B: Starting with g(x) 11/122, apply a sequence of transformations that will transform g back - into the original function f. Your formula for f should be in the form f(x) = A(x H) + K for some constants A, H, and K. f(x) = Find the inverse of the function f(x) notation. f-(x) = - 6x - 1 5 . Write your answer using inverse function